
یک مدل تحلیلی شبکه صف بندی با ظرفیت محدود دربرگیرنده انتشار ازدحام و انسداد
چکیده
مدل تحلیلی شبکه صف بندی معمولاً به دلیل مشکل بودن فهمیدن همبستگی بین صف ها فرض می کند که ظرفیت صف بندی بی نهایت می باشد. این همبستگی می تواند به توضیح انتشار ازدحام کمک کند. ما یک مدل برای شبکه صف بندی تحلیلی ارائه می دهیم که ظرفیت محدود صف ها را حفظ می کند و از پارامترهای ساختاری برای فهمیدن همبستگی بین صف ها استفاده می کند. برخلاف مدل های موجود قبلی توپولوژی شبکه و ظرفیت برون زایی صف ها حفظ می گردد. علاوه بر این، ازدحام مستقیماً توسط فرمولبندی جدید فضای حالت صف ها که به طور صریح فاز انسداد را مشخص می نمایند، مدلسازی می شود. بنابراین مدل می تواند منابع و اثرات ازدحام را توصیف نماید.
این مدل برای شبکه ها با توپولوژی دلخواه، چندین سرور برای صف و انسداد بعد از خدمات فرمولبندی می شود. این روش با مقایسه با مدل های موجود و نتایج شبیه سازی اعتبارسنجی گردید. سپس این روش برای مطالعه جریان بیمار در شبکه ای از واحدهای بیمارستان دانشگاه جنوا کاربردی گردید. این مدل به ما اجازه داده است که سه منبع اصلی انسداد تخت ها و تاثیر آن ها بر واحدهای مختلف بیمارستانی را کمی سازی نماییم.
1. مقدمه
یافتن منبع و اثرات ازدحام در داخل یک شبکه به ما اجازه می دهد تا رفتار آن را بهتر بشناسیم و عملکرد آن را بهبود بخشیم. مطالعه ازدحام در بخش های متنوعی کاربرد دارد، مانند تحلیل سرریز بازگشتی (انتشار بازگشتی ازدحام) در ترافیک شهری یا ترافیک پیاده رو ها (چه و اسمیت، 1994) و انسداد تخت های بیمارستانی (کوزومی و همکارانش، 2005) یا انسداد سلول های زندان (کورپورال و همکارانش، 2000).
متداول ترین رویکرد برای تحلیل ازدحام شبکه توسعه یک مدل شبیه سازی می باشد که جزئیات سیستم را در نظر بگیرد. استفاده از آن ها در یک چارچوب بهینه سازی طاقت فرسا می باشد. از سویی دیگر، مدل های تحلیلی به طور طبیعی برای این چارچوب مناسب می باشند، اما چنین مدل هایی به دلیل پیچیدگی مدلسازی انتشار ازدحام همزمان با حفظ انعطاف پذیری مدل به ندرت توسعه داده شده اند. ما بر روی مدل های تحلیلی و به طور خاص بر مدل های تحلیلی شبکه های صف بندی تمرکز می نماییم.
7. نتیجه گیری و کارهای آینده
ما یک مدل شبکه صف بندی تحلیلی ارائه دادیم که ویژگی محدود بودن ظرفیت یک سیستم واقعی را حفظ می نماید. این مدل برای چندین سرور با ظرفیت صف بندی محدود با توپولوژی دلخواه و سرویس بعد از انسداد فرمولبندی گردید. این مدل مبتنی بر تجزیه شبکه به صف بندی های واحد می باشد. پارامترهای ساختاری صف بندی به طور تقریبی به صورتی هستند که می توانند همبستگی بین صف بندی ها را لحاظ نمایند. برخلاف روش های قبلی توپولوژی شبکه و پیکربندی آن (تعداد صف بندی ها و پیکربندی آن ها) در طول تحلیل حفظ شده و نیاز به چک کردن هیچ قیدی از پیش نمی باشد.
Abstract
Analytic queueing network models often assume infinite capacity queues due to the difficulty of grasping the between-queue correlation. This correlation can help to explain the propagation of congestion. We present an analytic queueing network model which preserves the finite capacity of the queues and uses structural parameters to grasp the between-queue correlation. Unlike pre-existing models it maintains the network topology and the queue capacities exogenous. Additionally, congestion is directly modeled via a novel formulation of the state space of the queues which explicitly captures the blocking phase. The model can therefore describe the sources and effects of congestion.
The model is formulated for networks with an arbitrary topology, multiple server queues and blocking-after-service. It is validated by comparison with both pre-existing methods and simulation results. It is then applied to study patient flow in a network of units of the Geneva University Hospital. The model has allowed us to identify three main sources of bed blocking and to quantify their impact upon the different hospital units.
1. Introduction
Detecting the sources and effects of congestion within a network allows us to better understand its behavior and to improve its performance. The study of congestion is relevant in a variety of sectors ranging from the analysis of spillbacks (i.e. the backwards propagation of congestion) in urban traffic or pedestrian traffic (Cheah and Smith, 1994) to that of hospital bed blocking (Koizumi et al., 2005) or prison cell blocking (Korporaal et al., 2000).
The most common approach to analyze network congestion is the development of simulation models that capture the details of the underlying system. They are cumbersome to use within an optimization framework. On the other hand, analytic models naturally fit within such a framework but are rarely developed due to the complexity of modeling the propagation of congestion while preserving a flexible model. We focus on analytic models and more specifically on analytic queueing network models.
7. Conclusions and future work
We have presented an analytic queueing network model that preserves the finite capacity property of the real system. The model is formulated for multiple server finite capacity queueing networks with an arbitrary topology and blocking-after-service. The model is based on a decomposition of the network into single queues. The structural parameters of the queues are approximated so that they can account for the between-queue correlation. Unlike pre-existing methods the network topology and its configuration (number of queues and their capacity) are preserved throughout the analysis thus no constraints need to be checked a posteriori.
چکیده
1. مقدمه
2. چارچوب کلی
3. مروری بر ادبیات
3.1. روش های دقیق
3.2. روش های تقریبی
4. مدل
4.1. معادلات توازن کلی
4.2. نرخ های گذر
4.3. سیستم معادلات
5. اعتبارسنجی
5.1. اعتبارسنجی در مقابل روش های قبلی
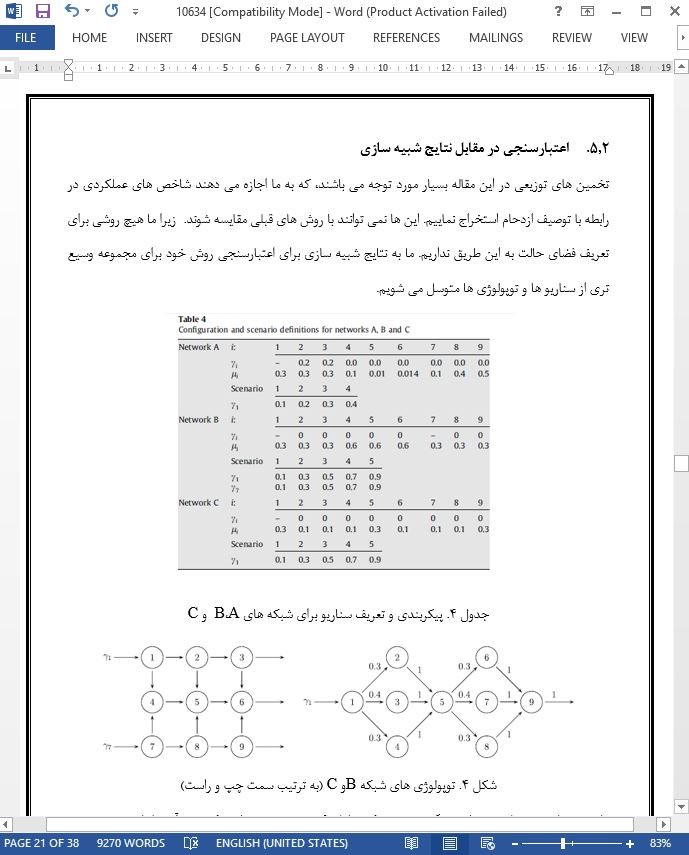
5.2. اعتبارسنجی در مقابل نتایج شبیه سازی
5.3. همگرایی اجراهای اعتبارسنجی
5.4. آزمایشات در شبکه های بزرگ
6. مطالعه موردی
6.1. شبکه های HUG
6.2. مقایسه با نتایج شبیه سازی
6.3. تحلیل ازدحام
7. نتیجه گیری و کارهای آینده
Abstract
1. Introduction
2. General framework
3. Literature review
3.1. Exact methods
3.2. Approximation methods
4. Model
4.1. Global balance equations
4.2. Transition rates
4.3. System of equations
5. Validation
5.1. Validation versus pre-existing methods
5.2. Validation versus simulation results
5.3. Convergence of the validation runs
5.4. Tests on larger networks
6. Case study
6.1. HUG network
6.2. Comparison with simulation results
6.3. Congestion analysis
7. Conclusions and future work
- ترجمه فارسی مقاله با فرمت ورد (word) با قابلیت ویرایش، بدون آرم سایت ای ترجمه
- ترجمه فارسی مقاله با فرمت pdf، بدون آرم سایت ای ترجمه
