
اندازه های نمونه کارآمد در برنامه ریزی غیرخطی تصادفی
چکیده
ما یک کلاس از برنامه های غیرخطی را که برای آن یک تقریب به راه حل بهینه موضعی از نظر کاهش کسری خطای هزینه اولیه مشخص شده است را در نظر می گیریم. ما نشان می دهیم که چنین راه حل تقریبی می تواند با حل تقریبی یک دنباله از تقریبهای متوسط نمونه یافت شود. مسئله کلیدی در این روش عبارتست از تعیین توالی تقریبهای متوسط نمونه و همچنین تعداد تکرارهایی که برای تقریب متوسط هر نمونه در این توالی صورت می-گیرد. ما نشان می دهیم که یکی می تواند این نیازمندی را بصورت یک مسئله بهینه سازی آرمانی که تابع هزینه آن کار محاسباتی مورد نیاز برای حصول کاهش خطای مورد نیاز است بیان کند. مشخصات این مسئله بهینه سازی آرمانی نیازمند دانش دقیق از مسائل و پارامترهای الگوریتمی است. از آنجا که مقادیر دقیق این پارامترها در دست نیست، ما از تخمین استفاده می کنیم که می تواند به روزرسانی شود. ما روش خود را با استفاده از دو نمونه عددی از طراحی مهندسی ساختاری نشان خواهیم داد.
6. نتیجه گیری
ما نشان داده ایم که حل کردن مسئله قطری کارآمد برای حصول یک استراتژی قطری می تواند زمانهای محاسبه کلی را در برنامه ریزی غیرخطی تصادفی کاهش دهد. بطور خاص این روش نیاز به تعیین پارامترهای الگوریتم را به وسیله حدس و گمان و روشهای عددی هزینه بردار کاهش می دهد. به جای آن مسئله قطری کارامد اندازه نمونه ها و تعداد تکرارها در هر مرحله را با استفاده از مقادیر تخمینی هزینه، نرخ همگرایی و خطای نمونه برداری تعیین می کند. حتی با استفاده از متلب، راه حل مسئله قطری تنها نیازمند زمان محاسبه است. تجربه محاسباتی ما نشان می دهد که مزیت روش قطری برای مسائل پیچیده تر و عظیم تر و زمانیکه یک راه حل با دقت بالا مطلوب باشد خیلی قابل توجه است.
Abstract
We consider a class of stochastic nonlinear programs for which an approximation to a locally optimal solution is specified in terms of a fractional reduction of the initial cost error. We show that such an approximate solution can be found by approximately solving a sequence of sample average approximations. The key issue in this approach is the determination of the required sequence of sample average approximations as well as the number of iterations to be carried out on each sample average approximation in this sequence. We show that one can express this requirement as an idealized optimization problem whose cost function is the computing work required to obtain the required error reduction. The specification of this idealized optimization problem requires the exact knowledge of a few problems and algorithm parameters. Since the exact values of these parameters are not known, we use estimates, which can be updated as the computation progresses. We illustrate our approach using two numerical examples from structural engineering design.
6. Conclusions
We have demonstrated that solving an auxiliary, efficient diagonalization problem to obtain a diagonalization strategy can reduce the overall computing times in stochastic nonlinear programming. In particular, this approach eliminates the need for determining algorithm parameters by means of guesswork or costly numerical experimentation. Instead, the efficient diagonalization problem determines sample sizes and numbers of iterations at each stage using estimated values of cost-to-go, rate of convergence, and sampling error. Even using Matlab, the solution of the diagonalization problem requires only seconds of computing time. Our computational experience indicates that the advantage of an efficient diagonalization approach is more substantial for larger, more complicated problems and when a high-precision solution is sought.
چکیده
1. مقدمه
2. قطری کارآمد
3. پیاده سازی مسئله قطری کارآمد
4. الگوریتم کلی
5. مطالعه موردی
6. نتیجه گیری
Abstract
1. Introduction
2. Efficient diagonalization
3. Implementation of efficient diagonalization problem
4. Overall algorithm
5. Numerical study
6. Conclusions
- ترجمه فارسی مقاله با فرمت ورد (word) با قابلیت ویرایش، بدون آرم سایت ای ترجمه
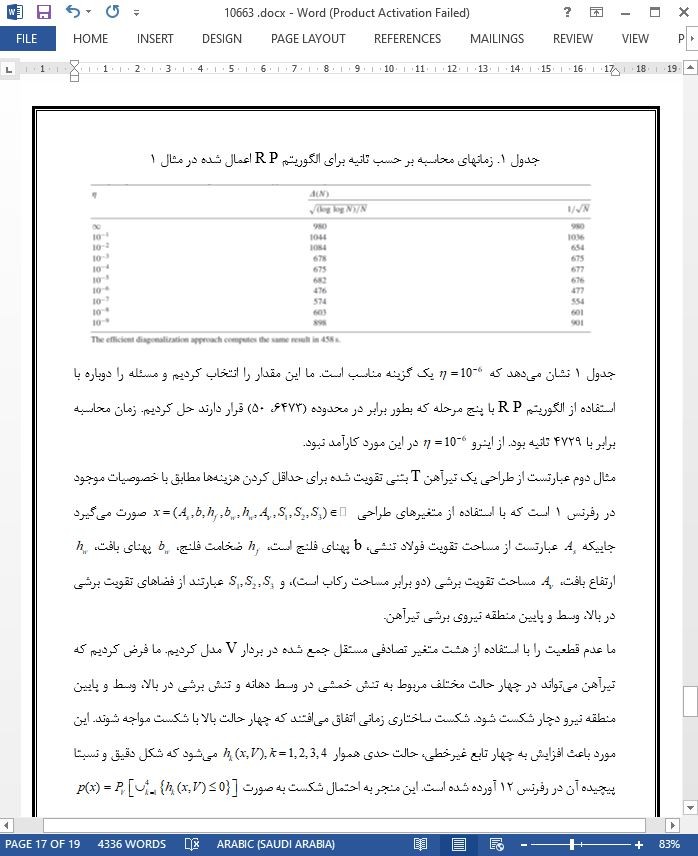
- ترجمه فارسی مقاله با فرمت pdf، بدون آرم سایت ای ترجمه
