
مدل آسیب لرزه ای مکانیکی برای بتن آرمه
چکیده
یک مدل برای ارزیابی آسیب ساختاری در ساختارهای بتن مسلح تحت حرکات زمین ناشی از زلزله ارائه می شود. آسیب به عنوان یک تابع خطی از حداکثر تغییر شکل و تاثیر بارگذاری تناوبی تکراری بیان می شود. داده های آزمایش دینامیکی (تناوبی) و استاتیکی (یکنواخت) موجود برای ارزیابی استاتیک پارامترهای مناسب مدل آسیب ارائه شده تجزیه و تحلیل شدند. همچنین عدم قطعیت در ظرفیت ساختاری نهایی مورد بررسی قرار گرفت.
مقدمه
در طراحی سازه های بتن مسلح مقاوم در برابر زلزله، به طور کلی لازم است تا اجازه درجاتی از آسیب داده شود. در غیر این صورت طراحی بیش از حد پرهزینه است. به منظور اجرای این فلسفه به درستی، مدل ها ملزم به ارزیابی آسیب ساختاری در چارچوب محیط زلزله تصادفی می شوند. یک سازه بتن مسلح با ترکیبی از معکوس استرس و گردش بالای استرس ضعیف یا آسیب دیده می شود. در نتیجه، هرگونه معیار آسیبی نباید تنها شامل حداکثر پاسخ باشد، بلکه باید اثر بارگذاری تناوبی تکراری نیز در برگیرد. (4،12). کدهای کنونی طراحی لرزه ای، مانند کدهای UBC و SEAOC، اصولاً براساس نتایج تحقیقاتی رفتار خمشی بتن مسلح تحت بارگذاری یکنواخت هستند (برای نمونه به مراجع 5، 11 و 23 رجوع کنید). تحت بارگذاری یکنواخت، می توان از حالات شکست ترد مانند شکست برشی و شکست پیوستگی از طریق جزئیات دقیق اعضاء اجتناب شود، و ظرفیت خمشی نهایی می تواند با دقت مورد بررسی قرار گیرد (5). با این حال، تحت بارگذاری تناوبی تکراری، اطمینان از اینکه چنین حالات شکست تردی اتفاق نخواهد افتاد، دشوار است.
خلاصه و نتیجه گیری
یک مدل آسیب لرزه ای برای اعضای بتن آرمه ارائه می شود. این مدل بر اساس این فرض است که آسیب کلی ناشی از حرکات زلزله از آسیب ایجاد شده توسط ماکزیمم تغییر شکل ساختاری و انرژی پسماند جذب شده تشکیل می شود. آسیب بر اساس یک شاخص D ، با مقادیر D≥1 با نشان دادن ویرانی کل اندازه گیری می-شود. داده های آزمایشی متناوب و یکنواخت موجود برای ارزیابی آمار پارامترهای مناسب مدل پیشنهادی آسیب تجزیه و تحلیل شدند. مشخص شد که توزیع لگاریتم طبیعی برای شاخص آسیب اجزای بتن آرمه مناسب میباشد. مقادیر شاخص آسیب باید با آسیب ساختارهای لرزه ای مشاهده شده کالیبره شوند؛ این کالیبراسیون در مرجع 29 توصیف می شود.
ABSTRACT
A model for evaluating structural damage in reinforced concrete structures under earthquake ground motions is proposed. Damage is expressed as a linear function of the maximum deformation and the effect of repeated cyclic loading. Available static (monotonic) and dynamic (cyclic) test data were analyzed to evaluate the statistics of the appropriate parameters of the proposed damage model. The uncertainty in the ultimate structural capacity was also examined.
INTRODUCTION
In earthquake-resistant design of reinforced concrete structures, it is generally necessary to permit some degree of damage; otherwise the design would be too costly. In order to implement this philosophy properly, models for assessing structural damage within the context of a random earthquake environment are required. A reinforced concrete structure is weakened or damaged by a combination of stress reversals and high stress excursion. Consequently, any damage criterion should include not only the maximum response, but also the effect of repeated cyclic loadings (4,12). Current seismic design codes, such as the UBC and SEAOC codes, are based primarily on research results of flexural behavior of reinforced concrete under monotonic loadings (e.g., Refs. 5, 11, and 23). Under monotonic loadings, brittle failure modes such as shear and bond failures can be avoided through careful detailing of the members, and the ultimate flexural capacity can be accurately evaluated (5). However, under repeated cyclic loadings, it is difficult to ensure that such brittle failure modes will not occur.
SUMMARY AND CONCLUSIONS
A seismic damage model for reinforced concrete members is proposed. The model is based on the premise that the total damage from earthquake motions is composed of the damage caused by the maximum structural deformation and the absorbed hysteretic energy. Damage is measured in terms of an index, D, with values D s 1.0 representing total collapse. Available monotonic and cyclic test data were analyzed to evaluate the statistics of the appropriate parameters of the proposed damage model. The lognormal distribution was determined to be appropriate for the damage index of reinforced concrete components. Values of the damage index should be calibrated with observed seismic damage of structures; this calibration is described in Ref. 29.
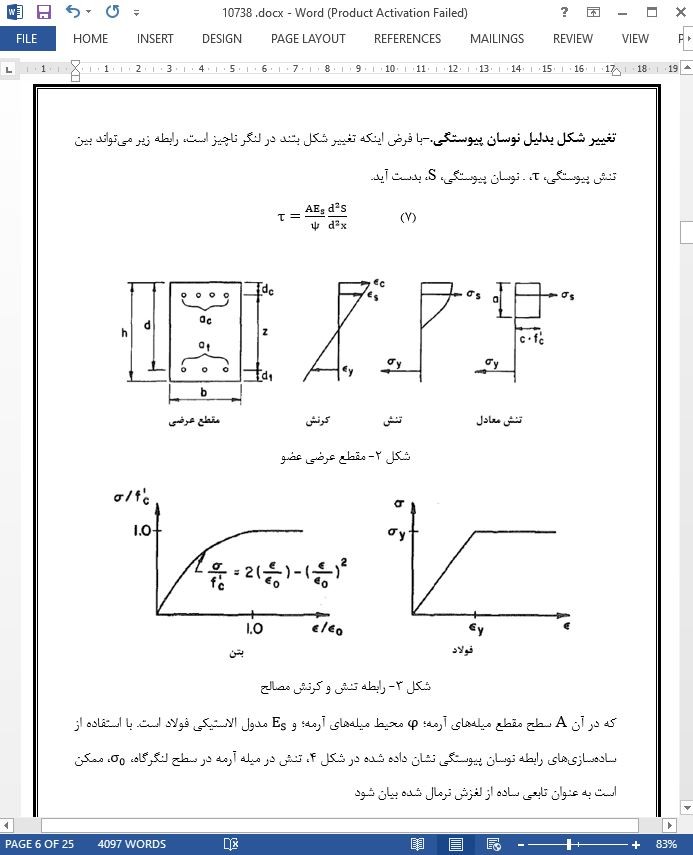
چکیده
مقدمه
مدل آسیب
تعیین پارامترهای مدل
آمارهای شاخص آسیب
خلاصه و نتیجه گیری
ABSTRACT
INTRODUCTION
DAMAGE MODEL
DETERMINATION OF MODEL PARAMETERS
DAMAGE INDEX STATISTICS
SUMMARY AND CONCLUSIONS
- ترجمه فارسی مقاله با فرمت ورد (word) با قابلیت ویرایش، بدون آرم سایت ای ترجمه
- ترجمه فارسی مقاله با فرمت pdf، بدون آرم سایت ای ترجمه
