مساله بوزینسک-میندلین برای یک نیم فضای غیرهمگن الاستیک
چکیده
مسأله ی بوزینسک برای فرورفتگی یک نیم فضای همگن و همسان الاستیک با یک پانچ دایره ای صلب و هم چنین مسأله ی میندلین در اعمال نیروی متمرکز بر داخل یک نیم فضای همگن و همسان الاستیک، از مسائل اساسی در نظریه ی مکانیک تماس هستند. ترکیب فرورفتگی سطح در حضور یک بارگذاری داخلی تحت عنوان مسأله ی بوزینسک-میندلین شناخته می شود و کاربردهای مهمی در حوزه ی ژئومکانیک دارد. مسأله ی بوزینسک-میندلین که به صورت بارگذاری خودتنیدگی است، مدلی مفید برای تفسیر مکانیک فرورفتگی در محیط خاکی جهت برآورد خواص الاستیسیته ی حجمی است. در این مقاله تحلیل مسأله برای تغییرات نمایی در مدول برشی الاستیک خطی واقع در نیم فضا انجام می شود.
1- مقدمه
مسأله ی فرورفتگی یک نیم فضای همسان الاستیک به وسیله ی یک فرورونده دایره ای صلب با یک پایه ی صاف بدن اصطکاک از مسائل اساسی در مکانیک تماس بوده که کاربردهای فراوانی در حوزه های مختلف علوم مهندسی از مهندسی مواد تا علوم زمین داشته است. بوزینسک [1] با استفاده از نتایج نظریه ی پتانسیل، و متعاقباً هاردینگ و اسندون [2] با استفاده از نظریه ی معادلات انتگرال دوگانه به حل این مسأله پرداخته اند. پس از این پیشرفت ها محققان به طور گسترده و جامع به بررسی حوزه ی مکانیک تماس پرداخته اند به حدی که نمی توان لیست جامعی از مقالات را در متن یک مقاله ی معمولی ردیف کرد. برخی از مطالعات برجسته که مرتبط با مکانیک تماس الاستواستاتیک باشد شامل هرتز [3]، شتائرمن [4]، گالین [5]، یوفلیاند [6] و لوره [7] بوده و هم چنین کورنوف [8]، لینگ [9]، گودمن [10]، سلوادورای [14-11]، گلادول [15]، جانسون [16]، کرنیر [17] و سلوادورای و آتلوری [18] ارجاعاتی را به مطالعات در زمینه ی مکانیک تماس داده اند.
7- نتیجه گیری
در این مقاله راه حل های مسأله ی آزمایش کابل پیش کشیدگی مرتبط با یک نیم فضای غیرهمگن الاستیک که در آن مدول برشی الاستیک خطی به صورت نمایی با تغییر فاصله ی عمودی از سطح فرورفته عمودی تغییر می کند، ارائه شده است. اما معادلات انتگرال حاصل را نمی توان با روش های تحلیلی قدیمی که در بررسی مسائل تماس از آن ها استفاده شده حل کرد. در این مقاله از یک روند گسسته سازی [11، 53، 61، 62] برای توسعه ی یک راه حل تقریبی استفاده شده است. نتایج حاصل از این تکنیک ارائه شده است و اثر ضریب پواسون، تغییرات مدول برشی با عمق و عمق بارگذاری نقطه ای میندلین بررسی شده است. باید خاطر نشان کرد که هدف اصلی آزمایش کابل پیش کشیدگی شامل تفسیر خواص ژئومکانیکی توده ی سنگی مورد آزمایش از طریق تفسیر رابطه ی بارگذاری – جابجایی در صفحه ی آزمایش می باشد.
Abstract
Boussinesq’s problem for the indentation of an isotropic, homogeneous elastic halfspace by a rigid circular punch constitutes a seminal problem in the theory of contact mechanics as does Mindlin’s problem for the action of a concentrated force at the interior of an isotropic, homogeneous elastic halfspace. The combined action of the surface indentation in the presence of the interior loading is referred to as the Boussinesq–Mindlin problem, which has important applications in the area of geomechanics. The Boussinesq–Mindlin problem, which represents a self-stressing loading configuration, serves as a useful model for interpreting the mechanics of indentation of geologic media for purposes of estimating their bulk elasticity properties. In this paper, the analysis of the problem is extended to include an exponential variation in the linear elastic shear modulus of the halfspace region.
1. Introduction
The problem of the indentation of an isotropic elastic halfspace by a rigid circular indenter with a frictionless flat base is a seminal problem in contact mechanics that has applications in a variety of areas in the engineering sciences ranging from materials engineering to the earth sciences. The solution to the problem was obtained by Boussinesq [1] using results of potential theory, and subsequently it was solved by Harding and Sneddon [2] using the theory of dual integral equations. Since these developments, the area of contact mechanics has been extensively and exhaustively examined by researchers to the point that an extensive list of articles cannot be documented within the context of a regular article. Some of the landmark studies related to elastostatic contact mechanics in general are due to Hertz [3], Shtaerman [4], Galin [5], Ufliand [6] and Lur’e [7], and references to studies in contact mechanics are also given by Korenev [8], Ling [9], Goodman [10], Selvadurai [11–14], Gladwell [15], Johnson [16], Curnier [17] and Selvadurai and Atluri [18].
7. Concluding remarks
The present work provides the solutions for the case of a Cable Jacking Test related to an isotropic non-homogeneous elastic halfspace region where the linear elastic shear modulus varies exponentially with distance normal to the indented surface. The resulting integral equations, however, cannot be solved using the conventional analytical procedures that have been used to examine contact problems. In this paper, a discretization procedure [11,53,61,62] is used to develop an approximate solution. The results developed using this technique are presented to examine the effect of the Poisson’s ratio, variation of shear modulus with depth and the depth of location of the internal Mindlin-type point load. It should be noted that the basic objective of the Cable Jacking Test is to interpret the geomechanical properties of the tested rock mass through an interpretation of the load-displacement relationship for the test plate.
چکیده
1- مقدمه
2- معادلات حاکم
3- فرورفتگی عادی در نیم فضای غیرهمگن الاستیک
4- یک روش گسسته سازی برای تحلیل مسائل تماس
5- اندرکنش صفحه صلب آزمایش و نیروی میندلین
6- نتایج عددی
7- نتیجه گیری
Abstract
1. Introduction
2. Governing equations
3. The normal indentation of the non-homogeneous elastic halfspace
4. A discretization approach for the analysis of the contact problem
5. Interaction of the rigid test plate and the Mindlin force
6. Numerical results
7. Concluding remarks
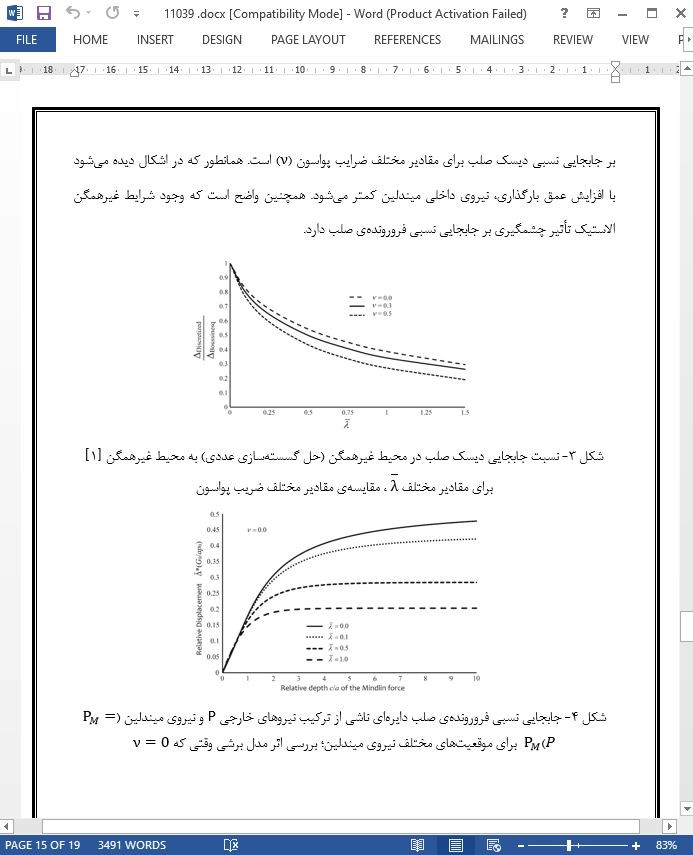
- ترجمه فارسی مقاله با فرمت ورد (word) با قابلیت ویرایش، بدون آرم سایت ای ترجمه
- ترجمه فارسی مقاله با فرمت pdf، بدون آرم سایت ای ترجمه