مطالعه ای بر جواب های امواج غبار صوتی رونده و مسیر نیمه دوره ای به آشوب در پلاسماهای غیرحرارتی
چکیده
رفتارهای دوشاخه شدگی و آشوبی امواج رونده ی غبار صوتی در پلاسماهای مغناطیسی با یون های غیرحرارتی با توزیع کایرنز-تسالیس در چارچوب معادله ی اصلاح شده ی کادومتسو-پتویاشیلی(FMKP) مورد بررسی قرا گرفته است. معادله ی FMKP با به کار گرفتن روش اختلال کاهشی(RPT) به دست آمده است. دو شاخه شدگی امواج رونده ی غبار صوتی به دست آمده از معادله ی FMKP نشان داده شده است. با استفاده از تئوری دو شاخه شدگی سیستم های دینامیکی خطی، دو جواب تحلیلی برای امواج رونده ی منفرد و تناوبی، بسته به پارامترهای و ، به دست آمده است. با در نظر گرفتن یک اختلال تناوبی خارجی، رفتار آشوبی امواج رونده ی غبارصوتی از طریق مسیر نیمه دوره ای به آشوب، مورد بررسی قرار گرفته است. پارامتر به طور چشمگیری رفتار آشوبی معادله ی مختل شده ی FMKP را تحت تاثیر قرار می دهد.
1-مقدمه
فیزیک پلاسماهای غباری موضوع حائز اهمیتی در تحقیقات روبه رشد است که طی چند دهه ی گذشته نه تنها از نقطه نظر آزمایشگاهی، بلکه از دیدگاه جنبه های جدید آن ]1[ در فضا و اخترفیزیک مدرن، تکنولوژی نیمه هادی، دستگاه های فیوژن، شیمی پلاسما، فیزیک کریستال و بیوفیزیک، بیشتر و بیشتر مورد توجه قرار گرفته اند. در سال 1989 گورتز ]2[ ، در مورد اثرات جمعی در پلاسماها که امواج گوناگونی مانند امواج چگالی در حلقه های سیارات و امواج پلاسمایی با فرکانس پایین را تحت تاثیر قرار میدهد، بحث و گفتگو کرد. محققان، احتمال تشکیل یک شبکه ی کولنی توسط ذرات باردار را مختصرا شرح داده اند. پلاسماهای غباری با دمای پایین در ساخت تراشه ها و پردازش مواد ]3،4[مورد استفاده قرار می گیرند که یکی از بزرگترین تاثیرات را در زندگی روزمره دارند.
نتایج
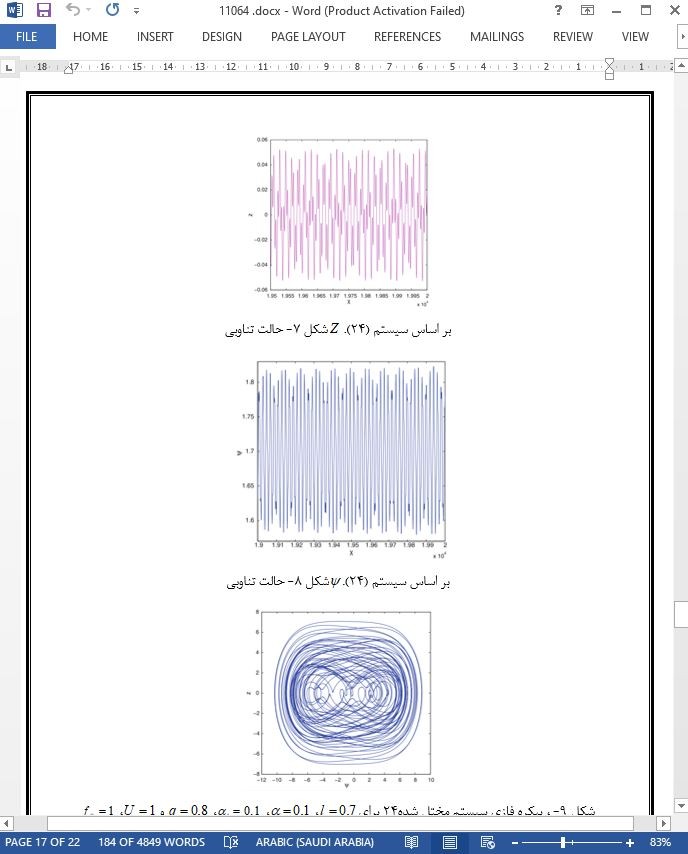
در این مقاله، معادله ی FMKP را برای امواج غبار صوتی در پلاسماهای مغناطیسی با یون های غیرحرارتی دارای توزیع کایرنز-تسالیس، به دست آورده ایم. با به کارگیری تئوری دوشاخه شدگی سیستم های دینامیکی تخت برای معادله ی FMKP، وجود امواج رونده ی منفرد و تناوبی را از طریق آنالیز صفحه ی فازی، نمایش داده ایم. دو جواب جدید تحلیلی برای امواج منفرد(فشرده و رقیق) و امواج تناوبی بسته به پارامترهای و به دست آمده است. با درنظر گرفتن یک اختلال تناوبی خارجی، رفتارهای شبه تناوبی و آشوبی امواج غبار صوتی از طریق محاسبات عددی، مورد مطالعه قرار گرفته است. حضور پارامترهای ، و به طور چشمگیری بر روی دوشاخه شدگی جواب های امواج رونده ی از معادله ی FMKP ، و بر روی رفتارهای شبه تناوبی و آشوبی معادله ی مختل شده ی FMKP تاثیر می گذارد. لازم به ذکر است که برای مجموعه ی یکسانی از پارامترهای و ، معادله ی مختل شده ی FMKP ، بر اساس مقدار اختلال تناوبی خارجی، رفتارهای شبه تناوبی و یا آشوبی را از خود نشان می دهد. همچنین باید ذکر شود که امواج غبار صوتی معادله ی مختل شده ی FMKP ، از طریق مسیر نیمه تناوبی به آشوبی، رفتارهای آشوبی را نمایش می دهند.
Abstract
Bifurcations and chaotic behaviors of dust acoustic traveling waves in magnetoplasmas with nonthermal ions featuring Cairns–Tsallis distribution is investigated on the framework of the further modified Kadomtsev–Petviashili (FMKP) equation. The FMKP equation is derived employing the reductive perturbation technique (RPT). Bifurcations of dust acoustic traveling waves of the FMKP equation is presented. Using the bifurcation theory of planar dynamical systems, two new analytical traveling wave solutions for solitary and periodic waves are derived depending on the parameters a; a1; q; l and U. Considering an external periodic perturbation, the chaotic behavior of dust acoustic traveling waves is investigated through quasiperiodic route to chaos. The parameter q significantly affects the chaotic behavior of the perturbed FMKP equation.
Introduction
The physics of dusty plasmas is an important topic of growing research which has gained more and more interest over the last few decades not only from the academic point of view, but also from the view of its new aspects [1] in space and modern astrophysics, semiconductor technology, fusion devices, plasma chemistry, crystal physics, and biophysics. In 1989, Goertz [2] discussed collective effects in dusty plasmas which affect various waves, such as density waves in planetary rings and low-frequency plasma waves. The authors described briefly the possibility of charged grains forming a Coulomb lattice. Low temperature dusty plasmas is used in manufacturing of chips and material processing [3, 4] in industry, which is one of the greatest impacts on our everyday lives.
Conclusions
In this paper, we have derived the FMKP equation for dust acoustic waves in magnetoplasmas with nonthermal ions featuring Cairns–Tsallis distribution. Applying the bifurcation theory of planar dynamical systems to the FMKP equation, we have presented the existence of solitary and periodic traveling waves through phase plane analysis. Two new analytical solutions for the solitary waves (compressive and rarefactive) and periodic waves are obtained depending on parameters a; a1; q; l and U. Considering an external periodic perturbation, the quasiperiodic and chaotic behaviors of dust acoustic waves are studied through numerical computations. The presence of the parameters q; a; and a1 affects significantly on bifurcation of traveling wave solutions of the FMKP equation, the quasiperiodic and chaotic behaviors of the perturbed FMKP equation. It should be noted that for same set of values of parameters a; a1; q; l and U, the unperturbed FMKP equation has solitary and periodic wave solutions, but the perturbed FMKP equation shows the quasiperiodic and chaotic behaviors based on the strength of the external periodic perturbation. It is also important to note that the dust acoustic waves of the perturbed FMKP equation represent the chaotic motions through quasiperiodic route to chaos.
چکیده
مقدمه
معادلات اساسی
به دست آوردن معادله FMKP
تشکیل سیستم دینامیکی
آنالیز صفحه فازی
جواب های تحلیلی امواج رونده
مسیر شبه تناوبی به آشوبی
نتایج
Abstract
Introduction
Basic equations
Derivation of the FMKP equation
Formation of dynamical system
Phase plane analysis
Analytical traveling wave solutions
Quasiperiodic route to chaos
Conclusions
- ترجمه فارسی مقاله با فرمت ورد (word) با قابلیت ویرایش، بدون آرم سایت ای ترجمه
- ترجمه فارسی مقاله با فرمت pdf، بدون آرم سایت ای ترجمه