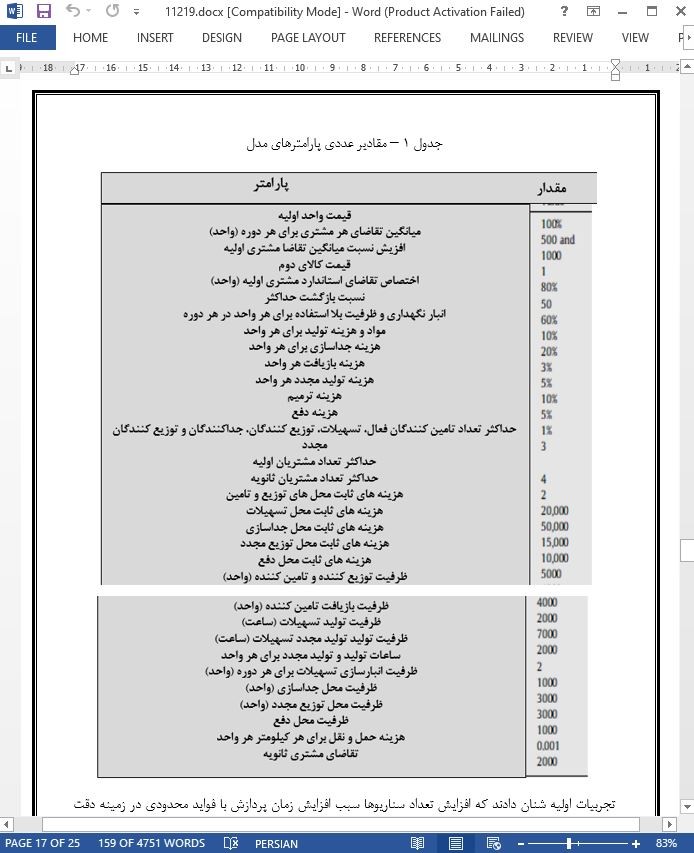
مدل تصادفی رو به جلو – معکوس برای طراحی شبکه لجستیک تحت ریسک
چکیده
توجه به شبکه های منطقی در طول دهه گذشته، از زمانی که اثر اقتصادی آن ها به شکل حائز اهمیتی افزایش یافته و با توجه به اینکه قوانین زیست محیطی سختگیرانه تر شده اند، بیشتر شده است. در این مقاله، یک طراحی شبکه منطقی چند مرحله ای (رده) جلو – معکوس تحت ریسک انجام شده است. ساختار شبکه پیشنهادی متشکل از سه رده در جهت رو به جلو (تامین کنندگان، امکانات و مراکز توزیع) و دو رده در جهت معکوس (امکانات و مراکز توزیع) می باشد، منطقه اولیه مشتری که تقاضا تصادفی بوده و سپس منطقه دوم که در آن تقاضا قطعی فرضی می گردد، اما ممکن است تصادفی نیز فرض گردد. مسئله در یک برنامه ریزی تصمیم گیری چند مرحله ای به صورت خطی عدد صحیح مختلط تصادفی فرموله شده است (SMILP). هدف به حداکثر رساندن سود مورد انتظار کل می باشد.
1. مقدمه
یک شبکه منطقی معکوس ارتباطی را میان بازار فراهم کننده محصولات دست دوم و محصولات جدید فراهم می آورد. هنگامی که این دو بازار با یکدیگر منطبق می شوند، آن را شبکه حلقه بسته می نامند در غیر این صورت به آن شبکه حلقه باز می گویند. (Salema, Barbosa Póvoa, & Novais, 2007a) تعداد بسیاری کمی از مدل های بهینه سازی زنجیره تامین با جریان معکوس به صورت غیرقطعی وجود دارند که از سناریوهایی به منظور حل مدل، با توجه به این که عدم قطعیت حل مدل های MILP را بسیار مشکل می سازد موجود دارند. (Salema, Barbosa Póvoa, & Novais, 2005).
7. نتیجه گیری
از این مطالعه، نتایج زیر را می توان بدست آورد.
مدل ارائه شده در طراحی شبکه منطقی جلو – معکوس موفق می باشد در حالی که چند دوره تقاضای تصادفی با چند رده (تامین کنندگان، تسهیلات و توزیع کنندگان) در جهت جلو و دو دسته (جداکنندگان و توزیع کنندگان مجدد) در جهت معکوس را در نظر می گیرد. تنها می تواند برای موارد منفرد، مشکلات محصول واحد در نظر گرفته شود. مدل به منظور حل مسائل بزرگتر انعطاف پذیر می باشد: با این حال، با توجه به اینکه تعداد سناریوها به صورت تصاعدی با بیشتر شدن تعداد دوره ها افزایش می یابند به سخت افزار قدرتمندتری نیاز دارد.
استفاده از مدل ارائه شده نشان داد که سود مورد انتظار کل به صورت مستقیم از تقاضای میانگین و نسبت بازگشت برای یک ظرفیت مشخص شبکه تاثیر می پذیرد. تنها دسته های با اعداد صحیح در طول یک دوره منتقل می شوند که کاربردهای مدل را محدود می نماید.
Abstract
Attention with reverse logistics networks has increased during the last decade since their economic impact has been increasingly important and as environmental legislation has been becoming stricter. In this paper, A multi-period multi-echelon forward–reverse logistics network design under risk model is developed. The proposed network structure consists of three echelons in the forward direction, (suppliers, facilities and distribution centers) and two echelons, in the reverse direction (disassembly, and redistribution centers), first customer zones in which the demands are stochastic and second customer zones in which the demand is assumed to be deterministic, but it may also assumed to be stochastic. The problem is formulated in a stochastic mixed integer linear programming (SMILP) decision making form as a multi-stage stochastic program. The objective is to maximize the total expected profit.
1. Introduction
A reverse logistics network establishes a relationship between the market that releases used products and the market for ‘‘new” products. When these two markets coincide, then it is called a closed loop network, otherwise it is called an open loop network (Salema, Barbosa Póvoa, & Novais, 2007a). Very few optimization models for the design of supply chains with reverse flows are available in literature under uncertainty using scenarios to solve the model as uncertainty makes MILP models very hard to solve (Salema, Barbosa Póvoa, & Novais, 2005).
7. Conclusion
From the previous study, the following conclusions can be derived.
The proposed model is successful in designing forward–reverse logistics networks while considering multi-period stochastic demand with three echelons (suppliers, facilities and distributors) in the forward direction and two echelons (disassemblies and redistributors) in the reverse direction. It can only be used for single item, single product problems. The model is flexible to solve larger problems; however, it requires more powerful hardware since the number of scenarios increases exponentially with the increase of the number of periods.
The application of the proposed model showed that the total expected profit is directly affected by demand mean and return ratio for a given capacity of the network. Only integer number of batches can be transported during a period which limits the application of the model.
چکیده
1. مقدمه
2. توضیحات مدل
3. فرضیان مدل و محدودیت ها
4. مدل فرمول
4.1 تابع هدف
4.2 محدودیت ها
4.2 محدودیت ها
5. نتایج و بحث
5.1 تاثیر میانگین تقاضا
5.2 اثر نسبت بازگشت
6. نمونه
7. نتیجه گیری
Abstract
1. Introduction
2. Model description
3. Model assumptions and limitations
4. Model formulation
4.1. Objective function
4.2. Constraints
5. Results and discussions
5.1. Effect of demand mean
5.2. Effect of return ratio
6. Example
7. Conclusion
- ترجمه فارسی مقاله با فرمت ورد (word) با قابلیت ویرایش، بدون آرم سایت ای ترجمه
- ترجمه فارسی مقاله با فرمت pdf، بدون آرم سایت ای ترجمه