آزمون CUSUM برای تشخیص تغییر میانگین پانل
چکیده
در این مقاله یک آزمون برای تغییر میانگین ساختاری پانل از CUSUM فرایندهای پانل توسعه یافته است. محدودکردن توزیع صفر آزمون و همچنین سازگاری آزمون نیز برقرار شده است. آزمون نشان داده است که اندازه نمونه محدود پایداری را نسبت به آزمون موجود از Horvath and Huskova (2012) که مبتنی بر CUSUM مجذور می باشد، دارا می باشد. اگر تغییرات میانگین در آن (آزمون) لغو نشود، میانگین آن ها از صفر دور می شود، آزمون پیشنهادی قدرت بهتری نسبت به آزمون موجود دارد. ازطرفی، اگر میانگین تغییرات تا حدی لغو شود، آنگاه آزمون موجود قدرت بهتری خواهدداشت. در این مقاله، آزمون های پیشنهادی با تجزیه وتحلیل مجموعه داده های واقعی نشان داده شده است.
1. مقدمه
مسائل تغییر ساختاری در مدل های داده ای پانل موضوعات مهمی برای تحلیل داده های اقتصادی و مالی هستند زیرا تغییر بزرگ سیاست اقتصاد کلان یا بحران های مالی تاثیر همزمانی بر بسیاری از متغیرهای اقتصادی و مالی دارند. تحقیقات برای مسائل تغییر ساختاری در مدل های پانل به دلیل مقدار گسترده داده ها در دنیای اقتصاد مدرن یا بازارهای مالی بسیار فعال می باشد. برخی مطالعات مانند مطالعات انجام شده توسط Bai (1997)، Bai et al. (1998) و Han and Park (1989) برای برآورد نقطه تغییر و آزمایش سری مدل های زمانی چند متغیره، و توسط Emerson and Kao (2001, 2002) برای آزمایش تغییر ساختاری رگرسیون روند زمانی در داده های پانل انجام شده است.
6. نتایج
ما آزمون تغییر میانگین پانل را مبتنی بر CUSUM فرایندهای پانل درنظر گرفتیم. آزمون اندازه عملکردی بهتری نسبت به آزمون موجود از Horvath and Huskova (2012) دارا می باشد. در مقایسه با آزمون موجود، آزمون پیشنهادی قدرت بهتری دربرابر تغییرات غیرلغو دارد اما در عوض قدرت بدتری نیز در برابر لغو تغییرات دارا می باشد. بنابراین، وقتی ما اطلاعات قبلی داریم که میانگین ها به جهت مشترکی همانند بحران مالی گسترده منتقل می شوند، ما آزمون پیشنهادی را به آزمون موجود ترجیح می دهیم. وقتی ما اطلاعات قبلی از لغو تغییرات داریم، آزمون موجود باید ترجیح داده شود. وقتی ما اطلاعات درباره جهت انتقال میانگین نداریم، نیاز به درنظر گرفتن هردو آزمون پیشنهادی و آزمون موجود داریم زیرا هیچ یک از دو آزمون در قدرت عملکردی بر دیگری غالب نمی باشند. اگر یک توضیح نظری درباره تفاوت مونته کارلو بین Sup B آزمون پیشنهادی و Sup H آزمون موجود داشته باشیم، بهتر خواهدبود. بررسی نقطه نظری نیز می تواند یک موضوع خوب برای تحقیق در آینده باشد.
Abstract
A test for panel structural mean change is developed from the CUSUM of the panel processes. Limiting null distribution and consistency of the test are established. The test is shown to have stable finite sample sizes than the existing test of Horvath and Huskova (2012) based on the squared CUSUM. If the mean changes are not cancelled in that their average is away from zero, the proposed test has better power than the existing test. On the other hand, if the mean changes are nearly cancelled, the existing test has better power. The proposed tests are illustrated by a real data set analysis.
1. Introduction
Structural change problems in panel data models are important issues for economic or financial data analysis because a big macroeconomic policy change or a financial crisis has simultaneous influence on many economic or financial variables. Researches for structural change problems in panel models have been activated due to a vast amount of data in the modern economic world or financial markets. Some studies were made by Bai (1997), Bai et al. (1998) and Han and Park (1989) for change point estimation and testing of multivariate time series models and by Emerson and Kao (2001, 2002) for testing of structural change of a time trend regression in panel data.
6. Conclusion
We have considered a panel mean change test based on the CUSUM of the panel processes. The test has better size performance than the existing test of Horvath and Huskova (2012). Compared with the existing test, the proposed test has better power against non-cancelling changes but has worse power against cancelling changes. Therefore, when we have prior information that the means are shifted to a common direction as in the world wide financial crisis, we may prefer the proposed test to the existing one. When we have the prior information of cancelling changes, the existing test should be preferred. When we do not have information on the direction of mean shifts, we need to consider both of the proposed test and the existing one because none of the two dominates the other one in power performance. It would be better if we have a theoretical explanation on the Monte-Carlo difference between the proposed test SupB and the existing test Sup H. Investigation of the theoretical point may be a good topic of future research.
چکیده
1. مقدمه
2. تشخیص تغییر میانگین
3. مقایسه مونته کارلو (Monte Carlo)
4. تشخیص تغییر نوسانی
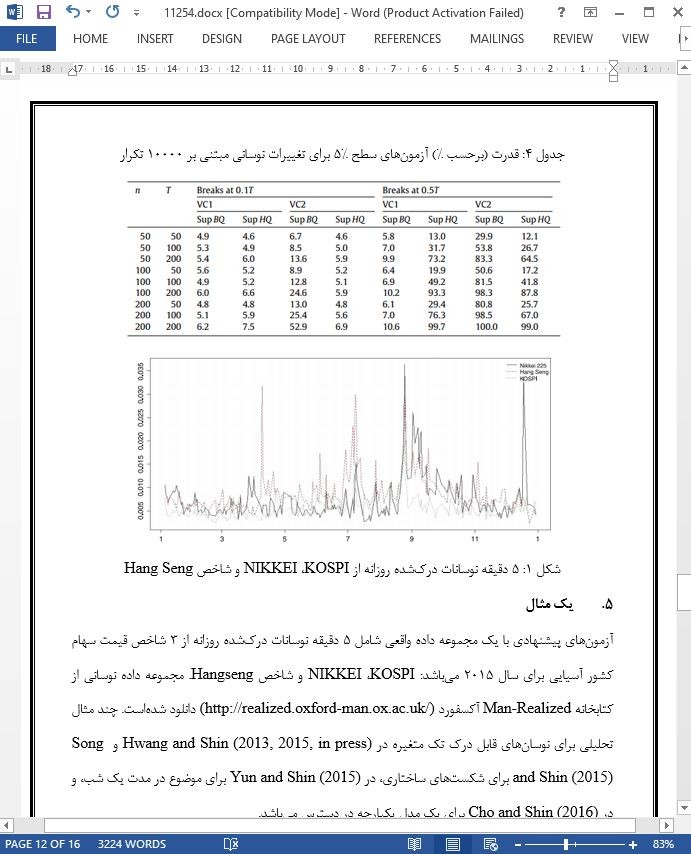
5. یک مثال
6. نتایج
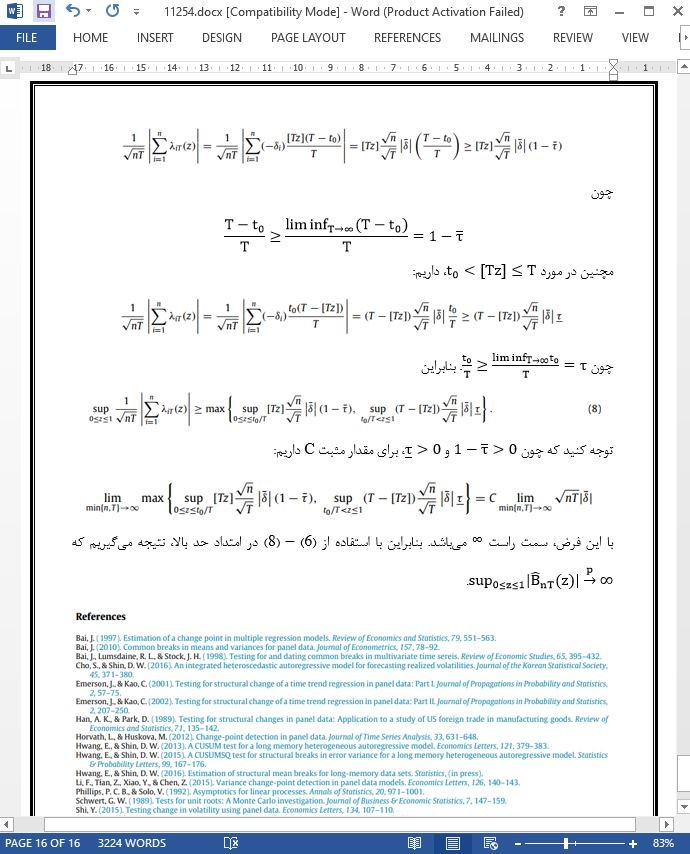
7. اثبات ها
Abstract
1. Introduction
2. Mean change detection
3. Monte Carlo comparison
4. Volatility change detection
5. An example
6. Conclusion
7. Proofs
- ترجمه فارسی مقاله با فرمت ورد (word) با قابلیت ویرایش، بدون آرم سایت ای ترجمه
- ترجمه فارسی مقاله با فرمت pdf، بدون آرم سایت ای ترجمه