اثرات عمق روی پاسخ لرزه ای تونل های دایره ای
چکیده
تونلها در نواحی که مستعد زلزله هستند در مقابل اثرات شدید بارگذاری زلزله آسیب پذیر میباشند. رویدادهای لرزه ای اخیر نشان داده است که نیازی برای صحت سنجی روشهای طراحی کنونی وجود دارد تا بتوان مکانیزمهای تغیر شکل متناظر با رفتار دینامیکی تونلها را بهتر درک نمود. تحقیق توصیف شده در این مقاله شامل مدلسازی فیزیکی و عددی از تونلهای دایره ای با آزمایشهای مرکز گریز دینامیکی و شبیه سازیهای مکمل المان محدود، میباشد. هدف این است تا درکی از اثرات عمق تونل روی رفتار لرزه ای تونلها را به دست آوریم. تونلهایی که دارای نسبتهای متفاوت عمق-به-قطر در ماسه ی سیلیسی، شل و خشک بودند آزمایش شدند. شتابهای اطراف تونل و فشارهای زمین روی پوشش داخلی تونل اندازه گیری شدند. یک دوربین دیجیتالی سرعت بالا برای ثبت تغیر شکل های خاک و پوشش داخلی تونل مورد استفاده قرار گرفت. تحلیل سرعت سنجی تصویر ذرات روی تصاویر ضبط شده برای اندازه گیری تغیر شکلها انجام گرفت. شبیه سازیهای مکمل المان محدود دینامیکی نیز با یک برنامه که قابلیت مدیریت شبیه سازیهای تماسی در سطح مشترک خاک-سطح داخلی تونل را داشت، انجام شدند. اندازه گیریهای آزمونهای مرکز گریز و تحلیل المان محدود نشان میدهند که به محض شروع شدن زمین لرزه تونل از یک تعادل استاتیکی به یک حالت تعادل دینامیکی تغیرحالت میدهد. هرچند، پیش بینی ماهیت تعادل دینامیکی با استفاده از روشهای تحلیلی مرسوم سخت است.
مقدمه
تونلها در نواحی که از لحاظ لرزه ای فعال اند مستعد این میباشند که در معرض بارهای دینامیکی قرار بگیرند که این ممکن است باعث شود فرارتر از حدهای ایمن طراحیشان تغیر شکل پیدا کنند. رویدادهای اخیر، از قبیل زمین لرزه کوب در ژاپن (1995)، زمین لرزه دوزس در ترکیه (1999)، زمین لرزه چی-چی در تایوان (1999)، زمین لرزه بام در ایران (2003)، و زمین لرزه ونچوان در چین (2008) تناسب این جمله را نشان دادند: دیده شده که بعضی از تونلها خسارتهای جبران ناپذیری را متحمل شدند. یکی از دلایل اصلی برای طراحی ناکافی این تونلها کمبود داده های تجربی و شواهد میدانی است ، که برای صحت سنجی پیش بینی های روشهای موجود طراحی مورد نیاز است. این چنین روشهایی از حل های ساده شده ی الاستیسیته ی شبه دینامیکی تا مدلهای پیچیده ی عددی برهمکنش خاک-ساختار گستردگی دارند. بررسی های فراگیر روشهای طراحی لرزه ای توسط وانگ (1993) و هاشاش و همکاران (2001) ارائه شده است.
خلاصه و نتیجه گیری ها
نتایج آزمونهای گریز از مرکز و تحلیل های FE نشان میدهد که رفتار دینامیک تونلهای دایره ای را میتوان به سه مرحله تقسیم نمود: مرحله ی گذرا، سیکلهای حالت دائم، و مرحله ی پسماند (پس از زلزله). طی مرحله ی گذار، که به مدت چندین سیکل اول طول میکشد، ساختار تونل به یک پیکربندی تعادل دینامیکی میرسد. بیشتر نیروهای پسماند که پس از توقف لرزش باقی میمانند در طی این دوره ساخته میشوند. مرحله ی گذار با سیکلهای حالت دائم همراه میباشد، که در طی آنها هم فشار زمین در اطراف تونل و هم نیروهای درون سطح داخلی تونل حول یک مقدار پسماند میانگین نوسان میکنند. پس از توقف لرزش، تنشهای پسماند در سطح داخلی تونل باقی میمانند.
Abstract
Tunnels in seismically active areas are vulnerable to adverse effects of earthquake loading. Recent seismic events have shown that there is a need to validate current design methods to better understand the deformation mechanisms associated with the dynamic behaviour of tunnels. The research described in this paper consists of physical and numerical modelling of circular tunnels with dynamic centrifuge experiments and complementary finite element simulations. The aim is to develop an understanding of the effects of tunnel depth on the seismic behaviour of tunnels. Tunnels with different depth-to-diameter ratios were tested in dry, loose silica sand. Accelerations around the tunnel and earth pressures on the lining were measured. A high-speed digital camera was used to record soil and lining deformations. Particle image velocimetry analyses were carried out on the recorded images to measure the deformations. Complementary dynamic finite element simulations were also conducted with a code capable of managing contact simulations at the soil–lining interface. Measurement of centrifuge experiments and finite element analyses show that the tunnel shifts from a static equilibrium to a dynamic equilibrium state as soon as the earthquake starts. The nature of the dynamic equilibrium, however, is difficult to predict using conventional analysis methods.
Introduction
Tunnels in seismically active areas are likely to be subjected to dynamic loads that may cause them to deform beyond their safe design limits. Recent events, such as the Kobe Earthquake in Japan (1995), Duzce Earthquake in Turkey (1999), Chi-Chi Earthquake in Taiwan (1999), Bam Earthquake in Iran (2003), and Wenchuan Earthquake in China (2008), demonstrated the appropriateness of this statement: some tunnels were seen to suffer damage beyond the limits of possible refurbishment. One of the main reasons for the inadequate design of these tunnels is the lack of experimental data and field evidence, which is needed to verify the predictions of available design methods. Such methods range from simplified pseudo-dynamic elasticity solutions to complex numerical soil–structure interaction models. Comprehensive reviews of seismic design methods are given by Wang (1993) and Hashash et al. (2001).
Summary and conclusions
Results of both centrifuge experiments and FE analyses show that the dynamic behaviour of circular tunnels can be split into three stages: transient stage, steady-state cycles, and residual (post-earthquake) stage. During the transient stage, which lasts for the first few cycles, the tunnel structure reaches a dynamic equilibrium configuration. Most of the residual forces that remain after the shaking stops are built up during this period. The transient stage is followed by the steady-state cycles, during which both the earth pressures around the tunnel and the forces in the tunnel lining oscillate around a mean residual value. After the shaking stops, residual stresses are left in the tunnel lining.
چکیده
مقدمه
انعطاف پذیری سطح داخلی تونل
مدلسازی گریز از مرکز دینامیک
مدل های تونل
محفظه مدل
چیدمان آزمونها و دستگاه ها
اماده سازی مدل و آزمایش کردن
مدلسازی المان محدود
مدلسازی تماسی
مدلهای ماده و تابع میدان خارجی
گسسته سازی فضایی
گسسته سازی زمان
نتایج
شتاب ها
تقویت- تضعیف شتابها
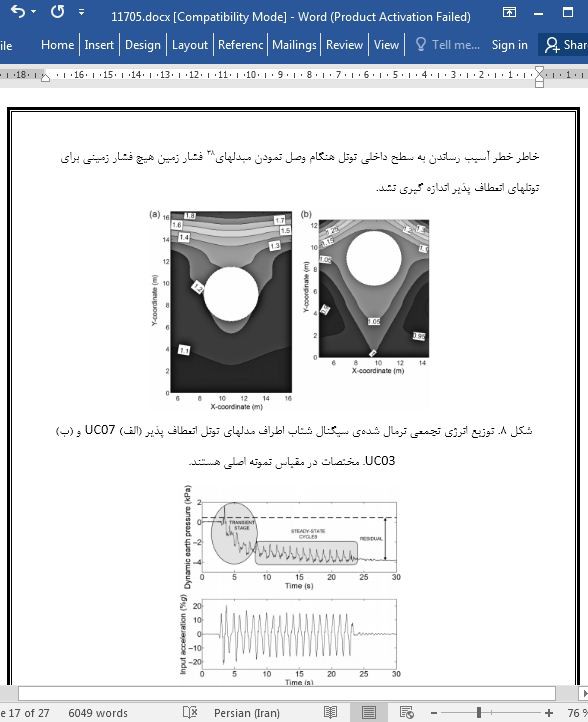
توزیع انرژی تجمعی
فشارهای زمین
تغیر شکلهای سطح داخلی و نیروها
ممانهای خمشی
نیروهای محوری سطح داخلی
خلاصه و نتیجه گیری ها
منابع
Abstract
Introduction
Flexibility of the tunnel lining
Dynamic centrifuge modelling
Tunnel models
Model container
Layout of tests and instrumentation
Model preparation and testing
Finite element modelling
Contact modelling
Material models and external field function
Spatial discretization
Time discretization
Results
Accelerations
Amplification–attenuation of accelerations
Distribution of cumulative energy
Earth pressures
Lining deformations and forces
Bending moments
Axial lining forces
Summary and conclusions
References
- اصل مقاله انگلیسی با فرمت ورد (word) با قابلیت ویرایش
- ترجمه فارسی مقاله با فرمت ورد (word) با قابلیت ویرایش، بدون آرم سایت ای ترجمه
- ترجمه فارسی مقاله با فرمت pdf، بدون آرم سایت ای ترجمه