واکنش های دینامیکی کوتاه مدت یک تونل کم عمق پوشانده شده در خاک اشباع شده
چکیده
تحقیقات قبلی، نتایج با ارزشی را در مورد واکنش های دینامیکی کوتاه مدت تونل های حفر شده در فضای کامل ارائه کرده اند. به هر حال، مدل نیم فضا از نظر کاربردی جذابیت بیشتری دارد، زیرا معمولا تونل ها دارای عمق محدود حفاری هستند. در این تحقیق، واکنش دینامیک یک تونل پوشانده شده بررسی شده است که خاک مجاور آن با استفاده از نظریه بیوت توصیف شده و پوشش تونل هم با استفاده از نظریه دینامیک ارتجاعی توصیف شده است. مرز مستقیم نیم فضا با استفاده از کمان محدب دارای شعاع بزرگ نشان داده می شود. با توجه به نظریه جمع گراف، راه های کلی در یک سیستم مختصات مستطیل تبدیل به جواب های موجود در سیستم مختصات قطبی می شود. جواب های به دست آمده برای جابه جایی و فشار خاک و پوشش ها و همچنین فشار روزنه های خاک در حوزه انتقال لاپلاس بر اساس شرایط مرزی به دست آمده اند. جواب های حوزه زمانی هم با استفاده از انتقال وارونه لاپلاس تعیین شدند. نتایج عددی ارائه شده نشان دهنده توزیع مقادیر پیک برای جابه جایی هلی زمین، فشارها و فشار روزنه های خاک می باشند.
7. نتیجه گیری ها
نتایج اصلی این تحقیق به صورت زیر جمع بندی شده اند:
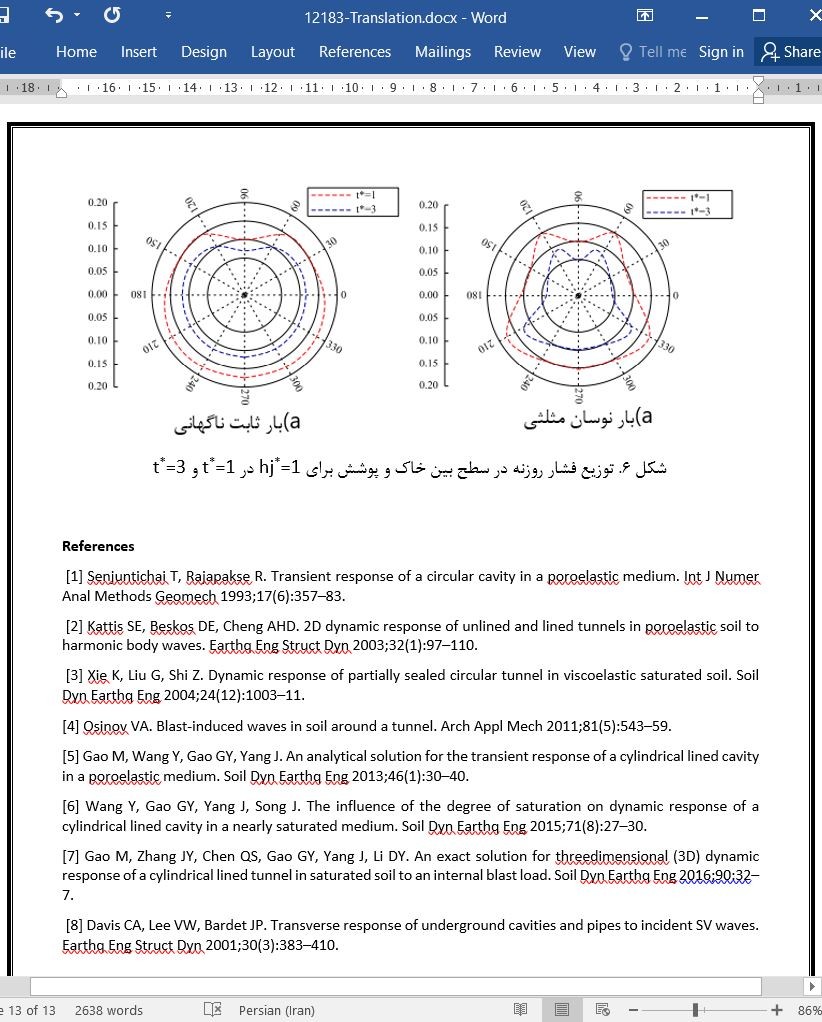
1) مقادیر حداکثر جابجایی های عمودی زمین با افزایش عمق حفر تونل کمتر می شوند. حداکثر مقادیر بیشینه در امتداد خط مرکزی تونل می باشند. حداکثر مقادیر و میدان نوسان حاصل از بار ثابت ناگهانی و بار تدریجی شبیه یکدیگر هستند و بیشتر از مقدار حاصل از بار نوسانی مثلثی هستند. میدان نوسان حاصل از جابه جایی های زمین در مرکز تونل به بیشترین مقدار می رسد و و بتدریج به سمت خارج کاهش می باید.
2) نقشه برجسته جابه جایی در خاک اشباع شده دارای شکل قلب است، زیرا جابه جایی روی تونل بیشترین اندازه است. با فرض اینکه عمق حفاری نامحدود است، نقشه برجسته جابه جایی باید یک دایره باشد. شیب جابه جایی در روی قسمت پوشانده شده تونل بیشتر است.
Abstract
Previous research has produced valuable results on the transient dynamic response of tunnels buried in full-space. However, a half-space model is of more practical interest because tunnels normally have finite buried depths. In this paper, the dynamic response of a lined tunnel is studied where the surrounding soil is described using Biot's theory and the lining is described by the theory of elastodynamics. The half-space straight boundary is approximately represented by a convex arc of large radius. In accordance with Graff's addition theorem, the general solutions in a rectangular coordinate system are converted to ones in a polar coordinate system. The solutions for displacements and stresses of both the soil and the lining as well as the pore pressure of the soil in the Laplace transform domain are derived based on boundary conditions. Time domain solutions are then obtained by the use of inverse Laplace transform. Numerical results are presented showing the distributions of peak values of ground displacements, stresses and pore pressures of the soil.
7. Conclusions
The main results of this study are summarized as follows:
(1) The peak values of vertical ground displacements tend to be smaller with increasing the buried depth of the tunnel. The maximum peak value appears along the center line of the tunnel. The peak values and amplitude induced by a suddenly applied constant load and a gradually applied step load are similar to each other and far greater than that induced by a triangular pulse load. The amplitude of ground displacement reaches the maximum value in the center of the tunnel and gradually reduces outwardly.
(2) The displacement contour in saturated soil shows a heart shape because the displacement above the tunnel is at its maximum. Assuming the buried depth value is infinite, the displacement contour should be a circle. Displacement contour induced by a suddenly applied constant load is much closer to a heart shape. The displacement gradient is greater right over the lining of the tunnel.
چکیده
1. مقدمه
2. معادلات کنترل کننده
3. انتقال هم پایه
4. روابط بین واکنش دینامیکی و پتانسیل ها
5. شرایط مرزی در مختصات قطبی
6. تاثیر عمق حفاری بر واکنش دینامیکی تونل
7. نتیجه گیری ها
منابع
ABSTRACT
1. Introduction
2. Governing equations
3. Coordinate transformation
4. The relations between dynamic response and potentials
5. Boundary conditions in polar coordinate
6. The effect of buried depth on dynamic response of the tunnel
7. Conclusions
References
- اصل مقاله انگلیسی با فرمت ورد (word) با قابلیت ویرایش
- ترجمه فارسی مقاله با فرمت ورد (word) با قابلیت ویرایش، بدون آرم سایت ای ترجمه
- ترجمه فارسی مقاله با فرمت pdf، بدون آرم سایت ای ترجمه