توسعه تیرهای فولاد سرد نورد شده (CFS) برای وضعیت حد خدمات و حد نهایی
چکیده
اجزای فولاد سرد نورد شده (CFS) به طور روز افزون به عنوان اجزای سازه ای اصلی در فعالیت های مدرن ساخت و ساز مورد استفاده قرار می گیرند. در حالی که انعطاف پذیری در فولاد سرد نورد شده برش های مقطعی امکان دست یابی به توانایی بیشتر مقاومت در برابر بار را از طریق استفاده از شکل های موثرتر فراهم می آورد، اما دست یابی به راه حل های بهینه در طراحی می تواند بدلیل وجود محدودیت های کاربر نهایی و رفتار پیچیده اجزای CFS در حالت های منطقه ای ، سراسری و خمیدگی متغیر کنترل شود. هدف این تحقیق، توسعه روشی تجربی برای طراحی بهینه مقاطع تیرهای CFS دارای حداکثر مقاومت نوسان و حداقل جابجایی ها در شرایط وجود بار و خدمات نهایی متناسب با استاندارد یوروکد 3 می باشد که در آن محدودیت های طراح کاربر نهایی و همچنین محدودیت های ساختاری در نظر گرفته می شوند. روش بهینه سازی Big Bang–Big Crunch برای بدست آوردن جواب های طراحی بهینه برای دوازده سازه CFS دارای برش های مقطعی مختلف مورد استفاده قرار گرفته است. برای تائید مقاومت نوسان و قدرت مقاطع تیرهای بهینه، مدلهای غیر خطی المان محدود (FE) با استفاده از ABAQUS و با در نظر گرفتن غیر خطی بودن مواد و تغییرات غیر خطی مواد و تغییرات هندسی اولیه ارائه شده اند. نشان داده شده است که مقاطع بهینه سازی شده بر اساس و ضعیت حد خدمات (SLS) و حد نهایی(ULS) می توانند کارایی 44% بیشتر و مقاومت خمیدگی 58 درصد بیشتر در مقایسه با تیرهای لوله ای دارای عرض و ضخامت صفحه ای یکسان داشته باشند. استفاده از برش های صفحه ای ساده و لبه دار دولا شده عموما منجر به بهترین داه حل های طراحی برای SLS و ULS می شود. در نهایت، نتایج دقیق بدست آمده از مدلهای FE برای ارزیابی دقت روشهای سه بعدی مطرح شده جهت برآورد توانایی تیرهای CFS و تغییر شکل در SLS و ULS استفاده می شوند.
7. خلاصه و نتیجه گیری
یک چارچوب جدید بهینه سازی برای توسعه تیرهای اقتصادی تر CFS دارای آتل های جانبی در وضعیت محدودیت خدمات و حد نهایی با توجه به روش عرض موثر EC3 و از طریق بهینه سازی ابعاد نسبی برش های مقطعی و امکان وجود لبه های تک لایه یا دو لایه و شبکه های واسطه مثلثی و سفت کننده های لبه دار معرفی شده است. برای داشتن جواب های بهینه، الگوریتم بهینه سازی Big Bang–Big Crunch (BB-BC) به کار گرفته شد، در حالی که متغیرهای طراحی با در نظر گرفتن محدودیت های طراحیEC3 و همچنین بازه محدودیت کاربر نهایی و ساخت تعیین شدند. چارچوب بهینه سازی معرفی شده برای 12 نمونه محتلف اعمال شد و دقت نتایج برای SLS و ULS از طریق مدلهای دقیق FE بررسی شدند. بر اساس نتایج ارائه شده در این تحقیق، نتایج زیر را می توان بیان کرد:
1- برای مواد یکسان، چارچوب بهینه سازی معرفی شده می تواند مقاومت خمیدگی و استقامت برش های محرک و استاندارد را تا 44 و 58 درصد افزایش دهد. در مجموع، برش های مقطعی بهینه سازی شده (در SLS و ULS) از شبکه های بلندتر و لبه های باریکتر استفاده می کنند. اختلافات اصلی بین شکل های بهینه سازی شده برای ULS,SLS مربوط به اندازه و زاویه سفت کننده های لبه ها و همچنین موقعیت شبکه واسطه و سفت کننده لبه ها می باشند.
Abstract
Cold-formed steel (CFS) elements are increasingly used as main structural members in modern construction practice. While flexibility of CFS cross-sectional shape allows achieving higher load carrying capacities by using more efficient shapes, obtaining optimum design solutions can be a challenging task due to end-use constraints and complex behaviour of CFS elements controlled by local, global and distortional buckling modes. This study aims to develop a practical methodology for optimum design of CFS beam sections with maximum flexural strength and minimum deflection under ultimate and serviceability load conditions, respectively, in accordance with Eurocode 3 by taking into account manufacturing and end-use design constrains. Population-based Big Bang–Big Crunch Optimisation method is employed to obtain optimum design solutions for twelve different CFS cross-sectional prototypes. To verify the flexural strength and stiffness of the optimum beam sections, detailed nonlinear finite element (FE) models are developed using ABAQUS by considering both material nonlinearity and initial geometrical imperfections. It is shown that the optimised sections based on serviceability limit state (SLS) and ultimate limit state (ULS) can provide, respectively, up to 44% higher effective stiffness and 58% higher bending moment capacity compared to a standard lipped channel beam section with the same plate width and thickness. Using plain channel and folded-flange sections generally leads to the best design solutions for SLS and ULS conditions, respectively. Finally, the results of detailed FE models are used to evaluate the adequacy of EC3 proposed procedures to estimate CFS beam capacity and deflection at ULS and SLS, respectively.
7. Summary and conclusions
A new optimisation framework was presented to develop more economical laterally braced CFS beam based on serviceability and ultimate limit states, according to EC3 effective width method, by optimising the relative dimensions of cross-sections and allowing for the inclined single or double edge lips and triangular intermediate web and flange stiffeners. To obtain optimum solutions, Big Bang–Big Crunch (BB-BC) optimisation algorithm was adopted while design variables were determined by taking into account EC3 design constraints as well as a range of manufacturing and end-use limitations. The proposed optimisation framework was applied on twelve different prototypes and the accuracy of the results at both SLS and ULS was examined through detailed FE models. Based on the results presented in this paper, the following conclusions could be drawn:
1. For the same amount of material, the proposed optimisation framework could increase the flexural capacity and stiffness of the standard benchmark section by 58% and 44%, respectively. In general, optimised cross-sections (at both SLS and ULS) tend to use taller web and narrower flanges. The main differences between optimised shapes for ULS and SLS are the size and the angle of edge stiffeners as well as the location of the intermediate web and flange stiffeners.
چکیده
1.مقدمه
2.اصول طراحی یوروکد
2.1خمیدگی منطقه ای
2.2خمیدگی نوسانی
2.3خمیدگی سراسری
3.تعریف مسئله
4.الگوریتم Big Bang-Big Crunch
5.طراحی بهینه برای تیرهای CFS
5.1 بهینه سازی وضعیت حد نهایی (ULS)
5.2 بهینه سازی برای وضعیت حد خدمات (SLS)
6. بررسی های تحلیلی
6.1 مدلهای تشریحی FE
6.2 نتایج FE برای برشهای بهینه و استاندارد
7. خلاصه و نتیجه گیری
منابع
ABSTRACT
1. Introduction
2. Eurocode design principals
2.1. Local buckling
2.2. Distortional buckling
2.3. Global buckling
3. Problem definition
4. Big Bang-Big Crunch algorithm
5. Optimum design of CFS beams
5.1. Optimisation for Ultimate Limit State (ULS)
5.2. Optimisation for Serviceability Limit State (SLS)
6. Analytical investigation
6.1. Detailed FE model
6.2. FE results of the standard and optimum sections
7. Summary and conclusions
References
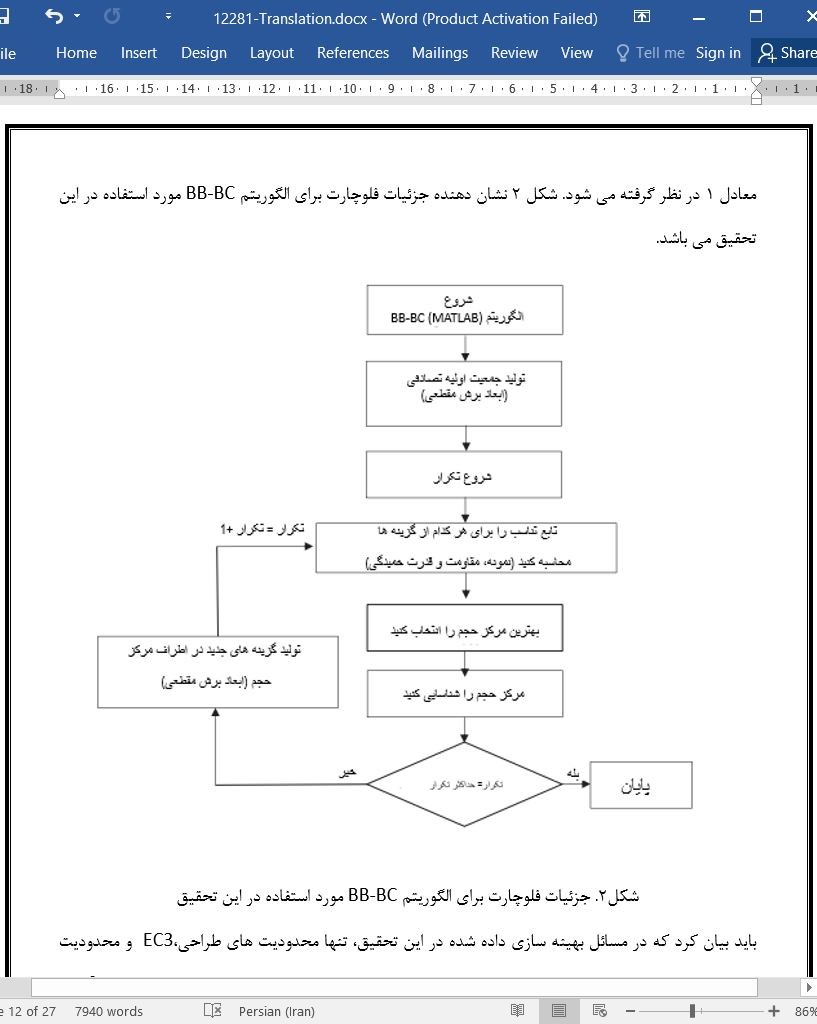
- اصل مقاله انگلیسی با فرمت ورد (word) با قابلیت ویرایش
- ترجمه فارسی مقاله با فرمت ورد (word) با قابلیت ویرایش، بدون آرم سایت ای ترجمه
- ترجمه فارسی مقاله با فرمت pdf، بدون آرم سایت ای ترجمه