دانلود مقاله حداقل سازی هزینه کلی در شبکه های توزیع با استفاده از پیکره بندی زمانی مقطعی فیدر و کنترل توان راکتیو
چکیده
در این مقاله، یک روش کارا و مطلوب جهت بررسی مسئله پیکره بندی زمانی مقطعی شبکه های توزیع همراه با کنترل توان راکتیو زمانی مقطعی پیشنهاد می شود. هدف، یافتن برنامه زمانی توزیع کلیه سوییچ های قابل کنترل و توان راکتیو ژنراتور های توزیع شده به منظور حداقل کردن هزینه کل است. محدودیت های دیگر شامل پروفیل ولتاژ، حداکثر تعداد عملیات سوییچ روزانه مجاز (MADSON)، حدود توان راکتیو و غیره، در نظر گرفته می شوند. استراتژی گروه بند انشعاب ها جهت ساده سازی مسئله ریاضی فرمول بندی شده استفاده شده و یک روش بهینه سازی ازدحام ذرات ترکیبی (HPSO) جهت جستجوی پاسخ بهینه، ارائه می شود. استنتاج تطبیقی فازی در روش HPSO به منظور عدم محصور شدن در شرایط بهینه محلی ترکیب می شود. الگوریتم PSO ترکیبی مبتنی بر استنتاج تطبیقی فازی پیشنهادی (FAHPSO) با زبان برنامه نویسی VC++6.0 پیاده سازی می شود. نسخه اصلاح شده شبکه توزیع 70 گرهی و چندین شبکه توزیع واقعی به منظور ارزیابی عملکرد روش پیشنهادی مورد استفاده قرار می گیرند. نتایج عددی نشان می دهند که روش پیشنهادی به عنوان یک روش کارا برای حداقل سازی هزینه کلی در سیستم های توزیع با ژنراتور های توزیع شده محسوب می شود.
1. مقدمه
پیکره بندی مجدد شبکه توزیع (DNR) از کارایی زیادی در عملکرد بهینه سیستم های توزیع برق برخوردار است. با تنظیم ساختار توپولوژی شبکه های توزیع، شرکت های تولید کننده برق می توانند انرژی برق را به شکل بهتری عرضه کرده و به منافع بیشتری دست یابند. فرمول بندی متناظر به صورت یک مسئله برنامه ریزی غیر خطی مختلط (MINLP) است. از طرفی، امروزه بسیاری از ژنراتور های توزیع شده به شبکه های توزیع متصل می شوند. ژنراتور های توزیع شده می توانند توان راکتیو را برای شبکه های توزیع به منظور بهبود عملکرد شبکه توزیع به عنوان شبکه های فرعی هنگام تامین توان اکتو نا مشخص به شبکه های برق عرضه کنند. از این رو، تلفیق پیکره بندی مجدد شبکه توزیع با کنترل توان راکتیو ژنراتور های توزیع شده حائز اهمیت است. با توجه به پیش بینی بار ساعتی و پیش بینی توان اکتیو ژنراتورهای توزیع شده، لازم است تا پیکره بندی مجدد شبکه توزیع و کنترل توان راکتیو ژنراتور های توزیع شده در حالت زمانی مقطعی انجام شود. به منظور انجام این موضوع، لازم است تا برنامه زمانی توزیع روز بعد محاسبه شود. در نتیجه، مسئله MINLP متناظر با پیچیدگی بیشتری همراه می شود. علاوه براین، با افزایش تعداد سوییچ های قابل کنترل و ژنراتور های توزیع شده، ممکن است مشکل چند بعدی بودن بروز کند.
5. نتیجه گیری
در این مقاله، مسئله پیکره بندی مجدد شبکه توزیع زمان مقطعی همراه با کنترل توان راکتیو بهینه ژنراتور های توزیع شده مورد بررسی قرار می گیرد. کارکرد های اصلی این تحقیق در زیر مورد اشاره قرار گرفته اند.
فرمول بندی پیکره بندی شبکه توزیع زمان مقطعی همراه با کنترل توان راکتیو بهینه ژنراتور های توزیع شده ارائه شده است. می توان فرمول بندی ارائه شده را برای شبکه های توزیع بزرگ اعمال کرد.
استراتژی گروه بندی انشعاب ها به منظور کاهش تعداد آیتم های خصوصیات ذرات ارائه شده است. با توجه به این استراتژی، می توان ترکیبی از آیتم های خصوصیات دودویی را با یک آیتم خصوصیت عدد صحیح ساده سازی کرد. این موضوع موجب تسریع این فرآیند و ذخیره شدن زمان مصرفی می شود.
Abstract
In this paper, an efficient methodology is proposed to deal with segmented-time reconfiguration problem of distribution networks coupled with segmented-time reactive power control of distributed generators. The target is to find the optimal dispatching schedule of all controllable switches and distributed generators' reactive powers in order to minimize comprehensive cost. Corresponding constraints, including voltage profile, maximum allowable daily switching operation numbers (MADSON), reactive power limits, and so on, are considered. The strategy of grouping branches is used to simplify the formulated mathematical problem and a hybrid particle swarm optimization (HPSO) method is presented to search the optimal solution. Fuzzy adaptive inference is integrated into basic HPSO method in order to avoid being trapped in local optima. The proposed fuzzy adaptive inference-based hybrid PSO algorithm (FAHPSO) is implemented in VC++ 6.0 program language. A modified version of the typical 70-node distribution network and several real distribution networks are used to test the performance of the proposed method. Numerical results show that the proposed methodology is an efficient method for comprehensive cost minimization in distribution systems with distributed generators.
I. INTRODUCTION
DISTRIBUTION network reconfiguration (DNR) is very useful to the optimal operation of electric distribution systems [1]–[3]. By regulating the topology structure of distribution networks, electric power companies can provide better electric power and gain more profits. The corresponding formulation is a mixed-integer nonlinear programming (MINLP) problem. At the same time, many distributed generators are connected into distribution networks today. Distributed generators can also provide reactive power to distribution networks to improve the operation of distribution network as ancillary contributions when they supply uncertain active powers to power grids. Hence, it is very significant to couple distribution network reconfiguration with reactive power control of distributed generators. Because of the hourly load forecast and active power forecast of distributed generators, both distribution network reconfiguration and reactive power control of distributed generators need to be done in the segmented-time mode. In order to do this, the dispatching schedule of next day should be calculated in advance. As a result, the corresponding MINLP problem becomes more complex. Moreover, as the number of the controllable switches and distributed generators increases, it may be curse of dimensionality.
V. CONCLUSION
In this paper, the problem of segmented-time distribution network reconfiguration, coupled with optimal reactive power control of distributed generators, is investigated. The main contributions are summarized as follows.
The formulation of segmented-time distribution network reconfiguration coupled with optimal reactive power control of distributed generators is presented. The presented formulation can be applied to large distribution networks.
The strategy of grouping branches is presented to reduce the number of particle property items. By this strategy, a couple of binary property items can be simplified to merely one integer property item. This can accelerate the search process and save the consumed time.
چکیده
1. مقدمه
2. فرمول بندی مسئله
a) محدودیت های شبکه شعاعی
b) محدودیت تعادل توان فعال:
c) محدودیت تعادل توان راکتیو
d) حداکثر محدودیت تعداد عملیات سوییچ مجاز روزانه
e) محدودیت حدود ولتاژ:
f) محدودیت حد جریان (یا حد حرارتی)
g) محدودیت حد ظرفیت توان راکتیو ژنراتور توزیع شده
3. روش پیشنهادی
A. مروری بر PSO
B. استراتژی گروه بندی انشعابات و مدل ذرات
C. الگوریتم PSO ترکیبی اصلی
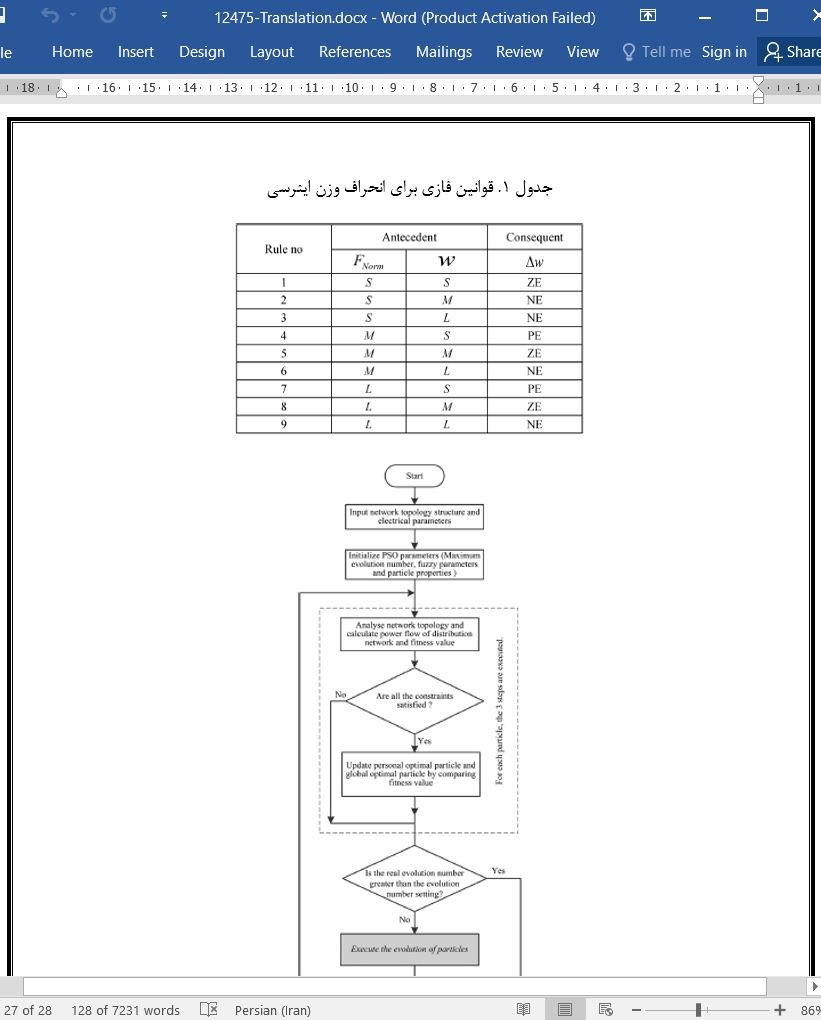
D. بهبود الگوریتم PSO ترکیبی اصلی با استفاده از استنتاج تطبیقی فازی
4 . آزمایشات عددی
A. شبکه آزمون موردی
B. آزمون گویایی استفاده از هزینه کل به عنوان هدف بهینه سازی
C. آزمون عملکرد استنتاج تطبیقی فازی
D. آزمون عملکرد FAHPSO در مقایسه با دیگر روش های PSO بهبود یافته
E. آزمون عملکرد FHAPSO در مقایسه با دیگر روش های هوش مصنوعی
F. آزمون گویایی ترکیب پیکره بندی مجدد شبکه توزیع با کنترل تان راکتیو ژنراتور های توزیع شده
G. آزمون تطبیق پذیری روش پیشنهادی جهت پیش بینی خطاها
H. آزمون تطبیق پذیری روش پیشنهادی برای شبکه های توزیع بزرگ
5. نتیجه گیری
منابع
Abstract
1. INTRODUCTION
2. PROBLEM FORMULATION
a) Radial network constraint:
b) Active power balance constraint:
c) Reactive power balance constraint:
d) Maximum Daily allowable switching operation number constraint:
e) Voltage limit constraint:
f) Current limit (Or heat limit) constraint:
g) Capacity limit constraint of reactive power of distributed generator:
.3 PROPOSED METHOD
A. Overview of PSO
B. Strategy of Grouping Branches and Particle Model
C. Basic Hybrid PSO Algorithm
D. Improvement of Basic Hybrid PSO Algorithm Using Fuzzy Adaptive Inference
4. NUMERICAL EXPERIMENTS
A. Test Case Network
B. Test of the Rationality of Using Comprehensive Cost as the Optimization Objective
C. Performance Test of Fuzzy Adaptive Inference
D. Performance Test of FAHPSO Compared With Other Improved PSO Methods
E. Performance Test of FAHPSO Compared With Other Artificial Intelligent Methods
F. Rationality Test of Coupling Reconfiguration of Distribution Network With Reactive Power Control of Distributed Generators
G. Adaptability Test of the Proposed Method to Forecast Errors
H. Adaptability Test of the Proposed Method to Large Distribution Networks
5. CONCLUSION
REFERENCES
- ترجمه فارسی مقاله با فرمت ورد (word) با قابلیت ویرایش، بدون آرم سایت ای ترجمه
- ترجمه فارسی مقاله با فرمت pdf، بدون آرم سایت ای ترجمه