دانلود مقاله استفاده از تکنیک RBF-FD بدون مش بندی تجزیه متعامد مناسب (POD) برای شبیه سازی معادلات آب کم عمق

چکیده
     هدف اصلی این مقاله ایجاد یک روش بدون مش بندی محلی موثر و سریع برای حل معادلات آب کم عمق در موارد یک و دوبعدی می باشد. معادلات یاد شده در دسته معادلات فرارفت طبقه بندی می شوند. جواب های معادلات فرارفت گیج کننده هستند، بنابراین، روش های عددی تجربی از جمله روش گالرین ناپیوسته و روش حجم محدود باید استفاده شوند. دراینجا، براساس روش تجزیه متعامد مناسب، می خواهیم یک روش بدون مش بندی سریع را بسازیم. برای این منظور، ما مدل های آب کم عمق را درنظر می گیریم و یک طرح گسسته زمانی مناسب را براساس تکنیک پیش بینی کننده-تصحیح کننده به دست می آوریم. بنابراین با استفاده از تکنیک تجزیه متعامد مناسب، یک مجموعه جدید از توابع پایه می توانند برای فضای جواب ایجاد شوند که در آن اندازه فضای جواب جدید کمتر از مساله اصلی می باشد. بنابراین، با استفاده از پایه های جدید، زمان CPU کاهش خواهد یافت. برخی مثال  ها برای نشان دادن کارایی تکنیک عددی حاضر مطالعه شده اند.

1- مقدمه
     انواع پدیده های فیزیکی و طبیعی مانند صدا، گرما، الکتروستاتیک، الکترودینامیک، جریان سیال یا برق با استفاده از معادلات دیفرانسیل جزیی (PDEها) توصیف شده اند. خوانندگان علاقمند می توانند به [104] برای کاربردهای مختلف معادلات دیفرانسیل جزیی در علوم و مهندسی و همچنین برای برخی روش ها در به دست آوردن جواب های آن ها مراجعه کنند.
    معادلات آب کم عمق (SW) می توانند بصورت یک ساده سازی از معادلات ناویر-استوکس درنظر گرفته شوند [102]. در مدل های آب کم عمق، طول موج افقی بسیار بزرگتر از عمق سیال است[102]. به این دلیل، برخی روش های عددی خاص مانند روش گالرکین پیوسته، حجم محدود و روش مش متحرک انطباقی می توانند برای حل مسائل فرارفت استفاده شوند.

1-1 بررسی اجمالی معادلات SW
در مقاله حاضر، ما سه مساله مقدار مرزی را در علوم آب درنظر می گیریم:
1- معادلات آب کم عمق 1بعدی و 2بعدی بدون جمله اصطکاک:
     تراهان و داوسون [101] یک روش گام زمانی محلی مرتبه دوم در یک چارچوب گالرکین ناپیوسته رانگ-کوتا (RKDG) را برای حل معادلات آب کم عمق بررسی کردند. مسائل شکست سد در معادلات آب کم عمق یک بعدی و دوبعدی مسائل جذابی در تحلیل عددی هستند و تحقیقات بسیاری در این مسائل انجام شده اند. جواب عمومی برای مساله شکست سد بوسیله کوزولینو و همکارانش توسعه یافته است [31].
     بنکالدون و همکارانش [2] یک روش بدون مش بندی پایدار شده جدید را براساس توابع پایه شعاعی برای حل عددی مسائل جریان همرفتی ایجاد کرده اند. این دسته از مسائل شامل معادلات برگرز چسبنده و معادلات ناویر-استوکس تراکم ناپذیر در اعداد رینولدز بالا می باشند. یک روش حجم محدود جدید در [3] براساس مرحله پیش بینی کننده و یک مرحله تصحیح کننده برای حل عددی معادلات آب کم عمق برای توپوگرافی هموار یا غیرهموار ارائه شده است. هدف اصلی [4] ارائه محدود کننده های شیب در توابع پایه شعاعی بدون مش بندی برای حل معادلات غیرخطی قوانین پایستگی با تابع شار است که وابسته به ضرایب ناپیوسته می باشد چونکه این روش براساس فرمول بندی گردآوری محلی می باشد. نویسندگان [1] حلی را مشابه به مساله ریمان معادلات آب کم عمق یک بعدی (SWE) با یک ناپیوستگی پله ای پایین ارائه کرده اند. با استفاده از دسته روش های پله ای-کسری، یک روش حجم محدود تصویری ساده و دقیق در [5] برای حل معادلات آب کم عمق در فضای دوبعدی ایجاد می شود. یک دسته جدید از روش حجم محدود در ]6[ برای حل جریان های آب کم عمق در محیط های متخلخل در شبکه های مثلثی نامنظم ارائه می شود که در آن این روش شامل دو مرحله است که می تواند بصورت یک روش پیش بینی کننده-تصحیح کننده تفسیر شود.

6- نتیجه گیری
     در بسیاری از زمینه های علوم کاربردی، مانند شیمی، فیزیک، مکانیک سیالات و غیره، ما باید معادلات فرارفتی و فرارفت-پخش-واکنش را حل کنیم. این معادلات بسیار مهم هستند و گاهی اوقات یافتن جواب های تحلیلی آن ها بسیار مشکل می باشد. بنابراین، استفاده از یک روش عددی مناسب برای این معادلات موضوع مورد علاقه محققان است. معادله آب کم عمق برای یک معادله مهم در مکانیک سیالات برای شبیه سازی ارتفاع و سرعت آب می باشد. در این مقاله، ما یک روش عددی سریع را با استفاده از روش تجزیه متعامد مناسب برای حل معادلات یاد شده پیشنهاد می کنیم. تکنیک گردآوری محلی برای به دست آوردن یک الگوریتم عددی برای حل معادلاتی که در علوم آب وجود دارند ارائه می شود. روش ارائه شده در اینجا براساس تقریب RBF و روش تفاضل محدود است که تکنیک RBF-FD را ایجاد می کند. همچنین، در مقاله حاضر، ما روش RBF-FD را با روش تجزیه متعامد مناسب برای کاهش زمان CPU ترکیب کردیم. تکنیک توسعه یافته در شش مساله آزمایشی استفاده شده است و نتایج شبیه سازی تایید می-کنند که روش جدید برای یافتن جواب های تقریبی مدل های ما مناسب است.

Abstract

     The main aim of this paper is to develop a fast and efficient local meshless method for solving shallow water equations in one- and two-dimensional cases. The mentioned equation has been classified in category of advection equations. The solutions of advection equations have some shock, thus, especial numerical methods should be employed for example discontinuous Galerkin and finite volume methods. Here, based on the proper orthogonal decomposition approach we want to construct a fast meshless method. To this end, we consider shallow water models and obtain a suitable time-discrete scheme based on the predictor-corrector technique. Then by applying the proper orthogonal decomposition technique a new set of basis functions can be built for the solution space in which the size of new solution space is less than the original problem. Thus, by employing the new bases the CPU time will be reduced. Some examples have been studied to show the efficiency of the present numerical technique.

1 Introduction

     A wide variety of physical and natural phenomena such as sound, heat, electrostatics, electrodynamics, fluid flow or elasticity have been described using partial differential equations (PDEs). We refer the interested reader to [104] for various applications of partial differential equations in sciences and engineering and also for some approaches in obtaining their solutions. The shallow water (SW) equations can be considered as a simplification of the Navier-Stokes equations [102]. In the shallow water models, the horizontal wave length is much larger than the depth of the fluid [102]. For this reason, some special numerical methods such as discontinuous Galerkin, finite volume and adaptive moving mesh methods can be used for solving the advection problems.

1.1 A brief review on SWs equations

In the current paper, we consider three boundary value problems in water science:

1. The 1D and 2D shallow water equations without friction term:

     Trahan and Dawson [101] investigated a second-order, local time stepping procedure within a Runge-Kutta discontinuous Galerkin (RKDG) framework to solve the shallow water equations. The dam-break problems in one- and two-dimensional shallow water equations are attractive problems in numerical analysis and many investigations have been done on these problems. The public solution for the dam-break problem has been developed by Cozzolino and his co-workers [31].

     Benkhaldoun and his co-workers [2] developed a new stabilized meshless method based on the radial basis functions for the numerical solution of convection-dominated flow problems. This class of problems includes viscous Burgers equations and incompressible Navier–Stokes equations at high Reynolds numbers. A new finite volume method has been proposed in [3] based on the predictor stage and a corrector stage for the numerical solution of shallow water equations for either flat or non-flat topography. The main aim of [4] is to propose slope limiters in meshless radial basis functions for solving nonlinear equations of conservation laws with flux function that depends on discontinuous coefficients as the method is based on the local collocation formulation. Authors of [1] presented the similarity solution to the Riemann problem of the one dimensional shallow water equations (SWE) with a bottom step discontinuity. Using the class of fractional-step procedures, a simple and accurate projection finite volume method is developed in [5] for solving shallow water equations in two-space dimensions. A new class of finite volume method is presented in [6] for solving shallow water flows in porous media on unstructured triangular grids in which the method consists of two stages which can be interpreted as a predictor-corrector procedure.

6 Conclusion

     In many areas of of applied sciences, such as chemistry, physics, fluid mechanics and etc, we have to solve the advection and advection-diffusion-reaction equations. These equations are very important and sometimes finding their analytic solutions is so difficult. Thus, applying a useful numerical method for these equations is a topic of interest for researchers. The shallow water equation is an important equation in fluid mechanics for simulating the height and velocity of water. In this paper, we suggest a fast numerical method using the proper orthogonal decomposition approach for solving the mentioned equations. The local collocation technique is presented to obtain a numerical algorithm for solving equations which arise in water sciences. The method presented here is based on the RBF approximation and finite difference approach that produces the RBFFD technique. Also, in the current paper, we combined the RBF-FD method with the proper orthogonal decomposition approach to reduce the CPU time. The technique developed is applied on six test problems and simulation results confirm that the new procedure is appropriate for finding the approximate solutions of our models.

(جهت بزرگ نمایی روی عکس کلیک نمایید)

چکیده
1- مقدمه
1-1 بررسی اجمالی معادلات SW
2- معادلات آب کم عمق 1بعدی و 2بعدی با جمله اصطکاک:
1-2 مرور اجمالی تکنیک های RBF و RBF-FD
1-3 روش تجزیه متعامد مناسب (POD)
1-4 اهداف اصلی و سازماندهی مقاله
2- دو پایه در موضوع تقریبات بدون مش بندی
2-1 درونیابی کریگینگ متحرک (MK)
2-2 تکنیک توابع پایه شعاعی (RBFها)
2-3 استفاده از یک پارامتر شکل مناسب
2-4 روش RBF-FD
2-5 مورد یک بعدی
6-2 ابرچسبندگی
2-7 مورد دوبعدی
3- یک ویژگی پایستگی (ویژگی C)
4- روش تجزیه متعامد مناسب
5- آزمایشات عددی
1-5 مساله آزمایشی 1. (برای بررسی ویژگی C)
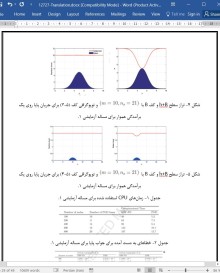
5-2 مساله آزمایشی 2 (مساله برآمدگی آب)
5-4 مساله آزمایشی 4 (مدل آب در وسط یک وان حمام یا انتشار یک موج سطحی هموار).
5-5 مساله آزمایشی 5 (شبیه سازی یک جریان شکست سد).
5-6 مساله آزمایشی 6 (مدل آب در یک وان حمام با جمله اصطکاک).
6- نتیجه گیری
منابع

Abstract
1 Introduction
1.1 A brief review on SWs equations
2. The 1D and 2D shallow water equations with friction term:
1.2 A brief review on RBF and RBF-FD techniques
1.3 Proper orthogonal decomposition (POD) approach
1.4 The main objectives and organization of the paper
2 Two bases in topic of meshless approximations
2.1 The Moving Kriging (MK) interpolation
2.2 The radial basis functions (RBFs) technique
2.3 Applying a suitable shape parameter
2.4 The RBF-FD procedure
2.5 One dimensional case
2.6 Hyperviscosity
2.7 Two-dimensional case
3 A conservation property (C-property)
4 Proper orthogonal decomposition method
5 Numerical experiments
5.1 Test problem 1. (To check the C-property)
5.2 Test problem 2 (Hump of water problem):
5.3 Test problem 3 (The shallow water equation with friction term).
5.4 Test problem 4 (Model of water in the middle of a bathtub or a smooth surface wave propagation).
5.5 Test problem 5. (Simulation of a dam-break flow)
5.6 Test problem 6 (Model of water in a bathtub with friction term).
6 Conclusion
References