دانلود رایگان مقاله ضریب کاهش مقاومت مبتنی بر آسیب برای ساختارهای غیرخطی

چکیده

       این مقاله ضریب کاهش مقاومت سیستم با یک درجه آزادی (SDOF) را که در معرض حرکات زمینی توالی نوع لرزه اصلی – پس لرزه قرار دارد، بررسی می کند. هم شکل پذیری جابجایی و هم آسیب تجمعی در ضریب کاهش درنظر گرفته می شوند. ثبت زلزله های اصلی و پس لرزه جمع آوری و برطبق ویژگی های مکان طبقه بندی شده است. حرکات زمین پس لرزه به طور متوالی به پنج سطح شدت نسبی مقیاس بندی شده اند. براساس تحلیل زمان تاریخ غیرخطی سیستم های SDOF، اثرات دوره طبیعی، ضریب شکل پذیری، شاخص آسیب و پس لرزه به طور آماری مطالعه شده است. نتایج نشان می دهند که حرکت زمین پس لرزه تاثیر چشمگیری بر فاکتورهای کاهش مقاومت دارد و ضریب کاهش مقاومت مبتنی بر آسیب حدودا 0.6 – 0.9 برابر ضریب کاهش مقاومت مبتنی بر شکل-پذیری می باشد. سرانجام، یک بیان تجربی برای ضریب کاهش مقاومت به وسیله تحلیل رگرسیون ایجاد شده است.

1. مقدمه

        بر طبق آمار، تقریبا 88% زلزله های قوی با پس لرزه همراه بوده اند. پس لرزه به عنوان زلزله کوچک پس از زلزله اصلی تعریف می شود، که بزرگترین زلزله در دنباله می باشد. آسیب ساختاری ناشی از زلزله اصلی تحت پس لرزه ها شدیدتر می شود و حتی می تواند منجربه فروریختن ساختاری شود. زلزله های نیوزیلند در سال 2010 [1] و نپال در سال 2015 [2] هر دو حرکات زمین لرزه اصلی و پس لرزه تجربه شد، و این دو مورد مثال هایی مناسب برای چرایی اهمیت موضوعات حرکات زمین توالی نوع در مرحله طراحی ساختاری می باشند. در سال های اخیر، محققان اثر زمین لرزه را از جنبه های مختلفی بررسی کردند. برخی مطالعات اثرات حرکات زمین توالی نوع را در طیف غیرالاستیک مانند طیف ضریب کاهش مقاومت [3, 4]، طیق آسیب [5]، طیف ضریب شکل پذیری [6, 7] و غیره بررسی کردند. دیگر مطالعات بر تغییرات پاسخ ساختاری، برای مثال، ساختمان های قاب فولادی [8] و قاب RC [9]، تحت حرکات زمین توالی نوع تمرکز کرده اند. تمامی نتایج به وضوح جابجایی اوج بزرگتر یا افزایش آسیب ساختاری ناشی از حرکات زمین توالی نوع را نسبت به زلزله اصلی نشان می دهند. اثر پس لرزه نبایستی در مرحله طراحی ساختاری نادیده گرفته شود.

        اصول طراحی لرزه ای کنونی شامل تحلیل رفتار الاستیک – پلاستیک سازه تحت زلزله های متوسط/نادر می باشد. چون مقاومت طراحی بیشتر سازه ها معمولا بسیار کمتر از حداقل مقاومت موردنیاز برای حفظ مرحله الاستیک تحت زلزله های قوی می باشد، اغلب ضریب کاهش برای کاهش تقاضای مقاومت الاستیک بکار می رود و به موجب آن تقاضای مقاومت الاستیک – پلاستیک سازه بدست می آید. تحلیل نظری و مطالعات تجربی ضرایب کاهش مقاومت نشان داده است که شکل پذیری ساختار یک اثر چشمگیر بر ضریب کاهش مقاومت دارد. ضریب شکل پذیری جابجایی به ارزیابی میزان آسیب سازه [10 – 12] کمک می کند. 

        علاوه برآن، آسیب تجمعی چرخه های پسماند غیرخطی نیز یک نقش مهم در تعیین سطح آسیب سازه ایفا می-کند. برخی مطالعات نشان دادند که آسیب تجمعی را می توان برای تصحیح ظرفیت شکل پذیری، مانند روش شکل پذیری معادل [13] یا معرفی ضریب شکل پذیری وزنی [14] لحاظ کرد. این روش ها به طور غیرمستقیم اثر آسیب تجمعی را لحاظ می کنند. برخی دیگر از مطالعات آسیب تجمعی را به طور مستقیم با بکارگیری مدل آسیب در تعیین تقاضای لرزه برای یک سطح آسیب مشخص یا سطح عملکردی معین درنظر گرفتند.

        همان طور که در بالا ذکرشد که پس لرزه آسیب سازه را تشدید می کند، با این حال، ضریب کاهش مقاومت مبتنی بر آسیب اثر حرکات زمین پس لرزه را بازتاب نمی کند. از این منظر، مطالعه حاضر این موضوع را از طریق محاسبات عددی گسترده در سیستم SDOF غیرخطی که در معرض حرکات زمین توالی نوع می-باشد، بررسی می کند. بخش 2 رکوردها (موارد ثبت شده) حرکت زمین زمین لرزه و پس لرزه واقعی را جمع آوری می کند که برای بررسی R_D بسیار ضروری می باشند. سپس رکوردهای جمع آوری شده برطبق شرایط مکان به دسته های مختلف تقسیم بندی می شوند. بخش 3 سطح عملکردی و پارامترهای محاسباتی مورد استفاده در محاسبه R_D را تعریف می کند. در بخش 4، تحلیل تاریخ زمانی الاستیک – پلاستیک گسترده سیستم SDOF غیرخطی با پارامترهای مختلف به منظور تعیین R_D برای دو مورد، یعنی، زمین لرزه اصلی و زمین لرزه به همراه پس لرزه انجام می شود. اثر ضریب شکل پذیری، شاخص آسیب و برخی دیگر از پارامترها در R_D نیز در بخش 5 از طریق مطالعات پارامتریک بررسی می شوند. سرانجام، یک فرمول تجربی برای ضریب کاهش مقاومت مبتنی بر آسیب در بخش 6 ارائه شده است.

2. رکوردها و طبقه بندی حرکات زمین توالی نوع

         رکورد حرکت زمین توالی نوع معمولا شامل یک رویداد لرزه اصلی و یک یا چند رویداد پس لرزه می باشد، که زمین لرزه (تنها لرزه اصلی)، ترتیبی از دو زلزله (لرزه اصلی بعلاوه یک پس لرزه)، ترتیبی از سه زلزله (لرزه اصلی بعلاوه دو پس لرزه) و غیره می باشد. سناریو لرزه اصلی بعلاوه یک پس لرزه معمولا در مطالعات قبلی درنظر گرفته شده است [5, 7, 8]. نتایج آن ها نشان می دهد که زلزله دو دنباله اطلاعات ارزشمندی را درباره اثر پس لرزه فراهم می کند. بنابراین، حرکت زمین توالی نوع در این مطالعه به عنوان یک لرزه اصلی بعلاوه یک پس لرزه مشخص می شود.

         برای ایجاد حرکت زمین از دو رویداد زلزله، می توان دو حرکت زمین مصنوعی [5] یا یک رکورد زلزله را با تکرار آن متصل کرد [16]. با این حال، این کاربرد از حرکات مصنوعی مصنوعی، ممکن است منجر به فرابرآورد (برآورد بیش از حد) مهم از حداکثر تقاضای رانش جانبی شود [17]. درجه فرابرآورد به دلیل ماهیت تصادفی شبیه سازی حرکت زمین مصنوعی مبتنی بر مورد می باشد. از طرفی، روش زلزله تکراری درواقع فرض می کند که لرزه اصلی و پس لرزه چگالی طیف قدرت یکسانی را دارا می باشند که ممکن است برای شرایط واقعی قابل دفاع نباشد. برای اجتناب از مشکلات بالا، این مطالعه از رکوردهای زلزله واقعی در مرکز تحقیقات مهندسی زلزله اقیانوس آرام (PEER) [18] و شبکه های لرزه نگار حرکت قوی (K-NET, KiK-net) [19] به منظور ایجاد حرکت زمین توالی نوع با استفاده از مراحل و معیارهای زیر استفاده می-کند: 1- جمع آوری رکوردها از ایستگاه های لرزه نگاری واقع شده در زمین آزاد یا ساختمان های کم ارتفاع برای اجتناب از اثرات احتمالی تعامل خاک – سازه؛ 2- در بین تمامی رکوردها از یک ایستگاه و از یک رویداد، رکوردی که اول رخ داده است و دارای حداکثر شتاب زمین (شتاب اوج) (PGA) بزرگتر از 0.10g است، به عنوان لرزه اصلی درنظر گرفته می شود، و رکوردی که دارای دومین PGA بزرگ می باشد و PGA آن بزرگتر از 0.05g است، به عنوان پس لرزه درنظر گرفته می شود؛ 3- قدر (بزرگی) زلزله لرزه اصلی و پس-لرزه به ترتیب بزرگتر از 0.6 و 0.5 می باشد؛ 4- اتصال لرزه اصلی و پس لرزه انتخابی با شکاف زمانی 100 s در بین آن ها، که برای توقف ارتعاشات ساختاری ناشی از لرزه اصلی کافی می باشد؛ 5- طبقه بندی حرکات زمین توالی نوع برطبق روش طبقه بندی مکان تحقیقات جغرافیایی ایالات متحده.

         به طور کلی، ما 342 رکورد حرکت زمین توالی نوع را برای کلاس های مکان B و C تشکیل دادیم که در جدول 1 لیست شده است. تعداد رکوردهای واجد شرایط برای کلاس های مکان A و D برای تشکیل یک تحلیل آماری معنی دار بسیار کم می باشند. برای تحلیل بیشتر، PGA لرزه اصلی تمامی رکوردهای حرکت زمین توالی نوع انتخابی در مقدار دقیق 0.2g درجه بندی شدند.

ABSTRACT

        This paper investigates the strength reduction factor of single-degree-of-freedom (SDOF) system subjected to the mainshock–aftershock sequence-type ground motions. Both displacement ductility and cumulative damage are considered in the reduction factor. Records of mainshock-aftershock earthquakes were collected and classified according to site properties. The aftershock ground motions in sequence are scaled to five relative intensity levels. Based on the nonlinear time-history analysis of inelastic SDOF systems, the effects of natural period, ductility factor, damage index and aftershock have been studied statistically. The results indicate that the aftershock ground motion has significant influences on strength reduction factors, and the damage-based strength reduction factor is about 0.6–0.9 times of the ductility-based strength reduction factor. Finally, an empirical expression for strength reduction factor was established by regression analysis.

1. Introduction

         According to statistics, about 88% of strong earthquakes are accompanied by aftershocks. An aftershock is defined as a smaller earthquake following the mainshock, which is the largest earthquake in the sequence. Structural damage caused by the mainshock is further aggravated under aftershocks and can even lead to structural collapse. The 2010 New Zealand [1] and the 2015 Nepal earthquakes [2] experienced both mainshock and aftershock ground motions, and are good examples of why sequence-type ground motions are important issues at the structural design stage. In recent years, researchers have explored the effect of aftershock from different aspects. Some studies explored the effects of sequence-type ground motions on inelastic spectra such as strength reduction factor spectra [3,4], damage spectra [5], ductility factor spectra [6,7], etc. Others focused on the changes of structural response, e.g. steel frame buildings [8] and RC frames [9], under sequence-type ground motions. All the results clearly show larger peak displacement or increased structural damage due to sequencetype ground motions than that of one major earthquake. The effect of aftershock should not be overlooked at the structural design stage.

         Current seismic design principles include analysis of a structure's elastic-plastic behavior under moderate/rare earthquakes. Since the design strength of most structures is generally muchower than the minimum strength required to maintain the elastic stage under strong earthquakes, a reduction factor is often used to reduce the elastic strength demand and thereby obtain the elasticplastic strength demand of a structure. Theoretical analysis and experimental studies of strength reduction factors have demonstrated that the structure ductility has a significant effect on the strength reduction factor. The displacement ductility factor helps to assess the extent of structural damage [10–12]. 

        Moreover, the cumulative damage of nonlinear hysteresis cycles also plays a significant role in determining the damage level of a structure. Some studies suggest that cumulative damage can be accounted for by modifying the ductility capacity, such as the equivalent ductility method [13] or introducing a weighted ductility factor [14]. These methods indirectly take into account the influence of cumulative damage. Some other studies consider the cumulative damage directly by employing a damage model in the determination of the seismic demand for a given damage level or performance level.

        As mentioned above that the aftershock will aggravate structural damage, current damage-based strength reduction factor, however, does not reflect the influence of aftershock ground motions. In this regard, the current study explores this issue through extensive numerical calculations on a nonlinear SDOF system subjected to sequence-type ground motions. Section 2 collects real mainshock and aftershock ground motion records that are essential to investigate RD. The collected records are then divided into different categories according to the site condition. Section 3 defines the performance level and computational parameters to be used in the calculation of RD. In Section 4, extensive elastic-plastic time history analysis of a nonlinear SDOF system with various parameters are then carried out to determine the RD for two cases, i.e., mainshock only and mainshock plus one aftershock. The influence of ductility factor, damage index and some other parameters on RD are explored in Section 5 through parametric studies. Finally, an empirical formula for damage-based strength reduction factor is proposed in Section 6.

2. Records and classification of sequence-type ground motions

         A sequence-type ground motion record usually consists of one mainshock event and one or multiple aftershock events, which are called as one earthquake (mainshock only), a sequence of two earthquakes (mainshock plus one aftershock), a sequence of three earthquakes (mainshock plus two aftershocks), and so on. Scenario of mainshock plus one aftershock was commonly considered in previous studies [5,7,8]. Their results demonstrated that twosequence earthquakes can provide valuable information about the influence of aftershock. Therefore, sequence-type ground motion in this study is specified as one mainshock plus one aftershock.

         To build up a ground motion of two earthquake events, one can connect two artificial ground motions [5] or connect a real earthquake record with its duplicate [16]. This usage of artificial ground motions, however, might lead to significant overestimation of maximum lateral drift demands [17]. The degree of overestimation is case-based due to the random nature of artificial ground motion simulation. The repeated earthquake methodology, on the other hand, actually assumes that the mainshock and aftershock have same power spectrum density which may not be tenable for real situation. To avoid the above problems, this study uses real earthquake records available in Pacific Earthquake Engineering Research Center (PEER) [18] and Strong-motion seismograph networks (K-NET, KiK-net) [19] to construct sequence-type ground motion by the following steps and criteria: (1) collect records from seismostations located on free-field or low-rise buildings to avoid possible soil-structure interaction effects; (2) among all the records from same station and same earthquake event, the one happening earlier and having a peak ground acceleration (PGA) larger than 0.10g is taken as the mainshock, the one having the second largest PGA and larger than 0.05g is taken as the aftershock; (3) the earthquake magnitude of mainshock and aftershock is larger than 6.0 and 5.0, respectively; (4) connect the selected mainshock and aftershock with a time gap of 100 s in between, which is long enough to cease structural vibrations caused by mainshock; (5) classify the connected sequence-type ground motion according to site classification method of United States Geological Survey.

        In total, we constructed 342 sequence-type ground motion records for site classes B and C as listed in Table 1. The number of qualified records for site classes A and D are too small to conduct any meaningful statistical analysis. For further analysis, the PGA of mainshock of all the selected sequence-type ground motion records were scaled to an identical value of 0.2g.

چکیده

1. مقدمه

2. رکوردها و طبقه بندی حرکات زمین توالی نوع

3. پارامترها و روندهای محاسباتی

3.1 تعریف سطح عملکردی و مقادیر حدی متناظر

3.2 روش تحلیل و پارامترهای سازه ای

4. میانگین ضرایب کاهش مقاومت

5. اثر پارامترهای مختلف

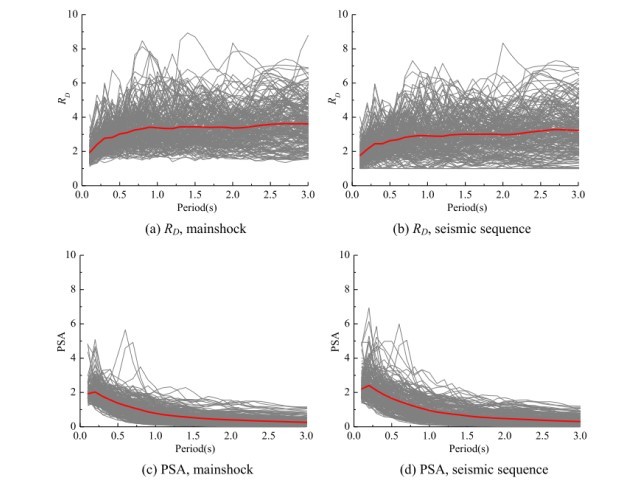
5.1 اثر پس لرزه بر طیف های R_D

5.2 اثر شرایط مکان بر طیف های R_D

5.3 اثر سختی پس از تسلیم بر طیف های R_D

5.4 اثر میرایی بر طیف های R_D

6. طیف های R_D پیشنهادی و مقایسه با طیف های R_μ  & R_D موجود

6.1 روابط تجربی پیشنهادی برای طیف های R_D

6.2 مقایسه طیف های R_D پیشنهادی با طیف های R_μ  & R_D موجود

7. نتایج

منابع

ABSTRACT

1. Introduction

2. Records and classification of sequence-type ground motions

3. Computational parameters and procedures

3.1. Definition of performance level and corresponding limit values

3.2. Analysis method and structural parameters

4. Mean strength reduction factors

5. Influence of various parameters

5.1. Effect of aftershock on the RD spectra

5.2. Effect of site conditions on the RD spectra

5.3. Effect of post-yield stiffness on the RD spectra

5.4. Effect of damping on the RD spectra

6. Proposed RD spectra and comparison with existing Rμ & RD spectra

6.1. Proposed empirical expressions for RD spectra

6.2. Comparison of the proposed RD spectra with existing Rμ & RD spectra

7. Conclusions

References