دانلود رایگان مقاله یک مدل تحلیلی برای رفتار تنش-کرنش بتن محدود

چکیده

            به خوبی شناخته شده است که قدرت و انعطاف پذیری بتن به شدت وابسته به سطح سلول های ارائه شده با تقویت جانبی هستند. سختی و رفتار سازنده تقویت محصور (الاستیک، الاستوپلاستیک، و غیره) عوامل مهم موثر بر رفتار بتن هستند. در این مطالعه، مدل بتن محصور ساده جدیدی برای توصیف ویژگی های تغییر شکل محوری و جانبی بتن تحت فشار سه محوری توسعه یافته است. رابطه تنش-کرنش بتن محصور در جهت محوری با منطقه الاستیک پیرو یک منحنی غیر خطی تعریف شده است. منطقه نزولی منحنی تنش-کرنش با استفاده از معیار انرژی شکست ثابت تعریف شده است. حد الاستیک، استحکام نهایی و ظرفیت باقی مانده بتن محصور شده با استفاده از معیار لئون-پرامونو تعیین شد. تغییر شکل جانبی بتن محصور است با استفاده از یک تابع توصیف شد که یک انتقال راحت از رفتار الاستیک به غیر ارتجاعی را فراهم می کند و شرایط کرنش حجمی صفر در مقاومت نهایی را برآورده می سازد. مدل در مقایسه با نتایج حاصل از آزمون سه محوری فشرده سازی بتن، الیاف و پلیمرهای تقویت شده (FRP) و آزمون های بتن فولاد محدود در مقالات پیشین گزارش شده است. سازش خوبی از نظر قدرت نهایی، قدرت باقی مانده و محوری و رفتار تغییر شکل جانبی مشاهده شده بود. مشاهده شده است که با از بین بردن نیاز به تنظیم پارامترهای مختلف برای انواع تقویت جانبی این مدل می تواند با موفقیت برای FRP و فولاد بتن محصور اعمال شود. در نهایت، یک مطالعه پارامتریک به منظور بررسی اثر نسبت آرماتور جانبی ، بتون قدرت و انرژی شکست فشاری بر رفتار فولاد و FRP بتن محصور انجام شد.

1. مقدمه

            ستون های بتن آرمه و شمع های پل با آرماتور جانبی برای افزایش قدرت و انعطاف پذیری اجزای به ویژه هنگامی که در معرض نیروهای ناشی از زلزله قرار می گیرند، حمایت می شود. از سوی دیگر، استفاده از الیاف پلیمر برای افزایش ظرفیت حمل بار محوری ستون ناکارا با افزایش محبوبیت در دهه گذشته به دست آورده است. علاوه بر این، بتن پر شده با لوله های فولادی که در آن بار محوری تنها توسط بتن محصور شده با لوله های فولادی تحمل می شود [1،2]، سیستم های سازه ای بسیار عالی را پیشنهاد می دهند که در آن هر دو مواد بطور موثر مورد استفاده قرار می گیرند. طراحی و تجزیه و تحلیل دقیق این اعضا می تواند به روشی ایمن و اقتصادی انجام شود تا زمانی که یک درک کامل از فعل و انفعالات بتن تقویتی محصور همراه با مدل هایی موجود باشد که قادر به ارائه رفتار بتن محصور است.

           از آنجا که شناسایی افزایش قدرت در بتن به عنوان تقویت محصور شده به دست می آید [3]، تلاش فوق العاده ای برای درک و رفتار مدل بتن محدود می باشد. برخی از مدل های قبلی بر اساس فشرده سازی دو محوره بودند [4/7] در حالی که برخی بر اساس شواهد تجربی محدود با اشاره به رفتار نرم شدن بتن محصور بودند [8]. آزمایش های فشرده سازی سه محوری [9/13] یک پایگاه داده گسترده در رفتار فشاری بتن ارائه کرد که می تواند برای توسعه و بررسی مدل های بتن محصور مورد استفاده قرار گیرد.

            مدلهایی برای توصیف رفتار تنش-کرنش محوری بتن محصور با فولاد بر اساس یک پایگاه داده گسترده ای از تحقیقات تجربی توسعه یافته است [14-16]. در این مدلها مقاومت نهایی و ناحیه افت منطقه از منحنی تنش-کرنش به عنوان تابعی از سلول های ارائه شده توسط نسبت آرماتور جانبی و مقاومت فشاری تک محوری تنظیم شده است.

          سادگی از این مدل ساخته شده آنها را برای استفاده در محاسبات ظرفیت و تجزیه و تحلیل مقطعی متداول ساخته است. یکی از نقطه ضعف های مهم این مدل عدم اصل کلی است. آنها تنها برای بتن مسلح با فولاد قابل استفاده هستند که در آن تقویت محدود می تواند با یک رابطه پلاستیک و قدرت اوج به دست آمده از بازده تقویت جانبی تخمین زده شود. با پیشرفت اخیر در بتن با مقاومت بالا و استحکام بالای فولاد و با استفاده از مواد کامپوزیت (الیاف به عنوان مثال مسلح پلیمر ( (FRP) به عنوان تقویت محدود ، این مدل ها به اندازه گیری بیشتر برای غلبه بر محدودیت های خود نیاز دارند. این مورد با توسعه سایر مدل ها برای بتن با مقاومت بالا و طبیعی [17،18] و توسط بتن FRP محصور شده برانگیخته شد [19،20]. یکی دیگر از اشکال مهم این مدل عدم عینیت در رژیم نرم شدن است. مهم نیست چه نوع تابعی برای نرم شدن منطقه انتخاب شود، تا زمانی که محلی سازی در نظر گرفته نشود،  منطقه نرم یک اثر اندازه را نشان خواهد داد و  نتایج هدف را نمی توان بدست آورد [21]. تایید این بحث در بسیاری از آزمایشات فشرده سازی تک محوره مشاهده شده است [22،23]. ساده ترین راه منظم سازی منطقه نرم شدن فشرده تقریباً جایی است که معمولا در المان محدود مورد استفاده قرار گرفته است، در یک بتون بر اساس شکستگی معیار انرژی شبیه به موارد بکار رفته در تست کشش و ترک خوردگی بتن است [24]. علاوه بر این، اهمیت برآورد سویه جانبی برای تعزیف «شکست" مرغون زمانی است که شکست با  تقویت یا از دست دادن خویشتن داری جانبی اعمال شده باعث کمانش میله های طولی شود. 

            مدل های مبتنی بر پلاستیسیته توصیف مناسبی از سخت شدن، نرم شدن، و رفتار انبساطی بتن ارائه می دهند و آنها به طور کلی با هم با یک محرک سازنده یا در تجزیه و تحلیل المان محدود مورد استفاده قرار می گیرد [25-27]. برای تجزیه و تحلیل سازه با هندسه پیچیده و شرایط محدود ، روش تجزیه و تحلیل المان محدود با استفاده از مدل پلاستیسیته بتن ابزار بسیار عالی [27] می باشد. حال، به منظور برآورد دقیق رفتار بار تغییر شکل محوری اعضای بتن بار محصور شده با روابط مختلف تشکیل دهنده مواد، مدل های ساده تری با مهندسین سازه در طراحی اولیه از این بخش دارند. در دل مشکل شرح واقعی از رفتار بتن در معرض فشرده سازی سه محوری باقی می ماند. زمانی که هرگونه مکانیزم محصور فعال یا انفعالی را بتوان شبیه سازی کرد. هدف این پژوهش، ارائه یک مدل مشابه و به منظور بررسی بتن محصور فولاد و FRP در معرض فشرده سازی محوری است. اعتقاد بر این است که مدل ارائه شده می تواند در طراحی اولیه ستون های فلزی محدود و یا در الیاف ستون ها طراحی مقاوم سازی پلیمر مفید باشند.

2. توضیحات مدل

           ابتدا، مدل پدیدارشناختی در این بخش توصیف می شود. این مدل یک نمایش جهانی به جای یک نمایش محلی برای بتن محوری در معرض بار ثابت است. سپس، این روش برای به دست آوردن رفتار ستون ها با سلول های غیر فعال (ستون حمایت شده با فولاد یا الیاف پلیمر) با برآوردن سازگاری کشیدگی بین بتن و تقویت جانبی پوشش برای سوابق محوری بارگذاری ارائه شده است. از آنجا که این مدل را می توان به عنوان نماینده عامل بتن در معرض فشرده سازی در نظر گرفت، تغییر شکل های استفاده شده زیر مقادیر متوسط بیش از نمونه به جای فشارها واقعی است. پس از آنکه محلی سازی شروع شود، توزیع تغییر شکل در امتداد ارتفاع نمونه یکدست نیست[28]. به جای ردیابی این توزیع کرنش غیر یکنواخت ، مقدار فشار متوسط در توضیحات زیر به طوری استفاده شده است که با رفتار متوسط تنش-کرنش یک ستون محدود می توان بدست آورد.
 

2.1. تغییر شکل محوری

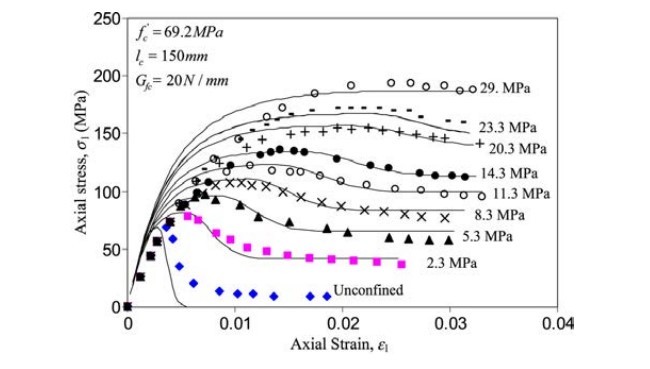
             برای بحث حاضر، جهت محوری و جانبی به ترتیب با 1 و 3 مشخص می شود. فرض بر این است که تنش ها و کرنش در دو جهت جانبی مشابه استε2 ≈ ε3) ، .(σ2 ≈ σ3 علاوه بر این، تنش فشاری و تغییر شکل مثبت فرض می شود در حالی که گسترش حجم منفی می باشد. پاسخ تنش-کرنش محوری بتن محصور شده با فشار جانبی ثابت را می توان با استفاده از سه مکان مشخص در منحنی تنش-کرنش توصیف کرد (شکل 1 ):

(1) حد الاستیک که بتن همسانگرد و الاستیک خطی فرض شود(σ1e, ε1e). . 
(2)  مقاومت نهایی مقاومتی است که تابع فشار جانبی است، ε10) ،. (σ10 
(3)  ظرفیت باقی مانده به عنوان نتیجه اصطکاک داخلی است (σ1r).

Abstract

          It is well known that the strength and ductility of concrete are highly dependent on the level of confinement provided by the lateral reinforcement. The stiffness and the constitutive behavior of the confining reinforcement (elastic, elastoplastic, etc.) are the important factors affecting the behavior of concrete. In this study, a new simple confined concrete model is developed for describing the axial and lateral deformation characteristics of concrete under triaxial compression. The stress–strain relationship of confined concrete in the axial direction is defined with an elastic region followed by a nonlinear curve. The descending region of the stress–strain curve is defined using a constant failure energy criterion. The elastic limit, ultimate strength, and residual capacity of confined concrete are determined using the Leon–Pramono criterion. The lateral deformation of confined concrete is described using a function that provides a smooth transition from elastic to inelastic behavior and satisfies the zero volumetric strain condition at ultimate strength. The model estimations are compared with the results of triaxial concrete compression tests, and fiber reinforced polymer (FRP) and steel confined concrete tests reported in the literature. A good agreement was observed in terms of ultimate strength, residual strength, and axial and lateral deformation behavior. It was observed that the model can be successfully applied for FRP and steel confined concrete, removing the need to adjust parameters for different lateral reinforcement types. Finally, a parametric study was conducted to investigate the effect of the lateral reinforcement ratio, concrete strength, and compressive failure energy on the behavior of steel and FRP confined concrete.

1. Introduction

           Reinforced concrete columns and bridge piers are provided with lateral reinforcement to increase the strength and ductility of these members especially when subjected to earthquake induced forces. On the other hand, the use of fiber reinforced polymers to increase the axial load carrying capacity of deficient columns has gained increasing popularity within the last decade. Furthermore, concrete filled steel tubes where the axial load is solely carried by concrete confined by steel tubes [1,2] offer excellent structural systems where both materials are utilized efficiently. The design and detailed analyses of these members can be performed in safe and economic ways as long as a thorough understanding of the confining reinforcement–concrete interactions is available along with models that are capable of representing confined concrete behavior.

           Since the recognition of strength gain in concrete as a result of confining reinforcement [3], there has been a tremendous effort to understand and model confined concrete behavior. Some of the earlier models were based on biaxial compression experiments [4–7] whereas some were based on limited experimental evidence regarding softening behavior of confined concrete [8]. Recent triaxial compression experiments [9–13] provided an extended database on compressive behavior of concrete that can be used to develop and verify confined concrete models.

           Models for describing axial stress–strain behavior of steel confined concrete have been developed on the basis of an extensive database of experimental research [14–16]. In these models the ultimate strength and the descending region of the stress–strain curves have been adjusted as a function of confinement provided by the lateral reinforcement ratio and uniaxial compressive strength. The simplicity of these models has made them popular for use in capacity calculations and sectional analyses. One important disadvantage of these models is their lack of generality. They are applicable to only steel confined concrete where the confining reinforcement can be approximated by an elastic–perfectly plastic relationship and peak strength is achieved when the lateral reinforcement yields. With recent advances in high strength concrete and high strength steel and with the use of composite materials (i.e. fiber reinforced polymers (FRPs)) as confining reinforcement, these models require further calibrations to overcome their limitations. This motivated the development of other models for normal and high strength concrete [17,18] and FRP confined concrete [19,20]. Another important drawback of these models is the lack of objectivity in the softening regime. No matter what kind of function is selected for the softening region, as long as localization is not taken into account, the softening region will exhibit a size effect and objective results cannot be achieved [21]. The verification of this argument has been observed in many uniaxial compression experiments [22,23]. The simplest way of regularizing the compressive softening region approximately, which is usually used in finite element analyses, is based on a constant fracture energy criterion similar to that applied for tensile cracking of concrete [24]. Furthermore, the importance of estimating lateral strains to define ‘failure’ is appreciated when failure is dictated by the rupture of the confining reinforcement or loss of lateral restraint causing buckling of longitudinal bars.

           Plasticity based models provide a convenient description of the hardening, softening, and dilatation behavior of concrete and they are generally used together with a constitutive driver or within finite element analysis [25–27]. For the analyses of structures with complicated geometry and boundary conditions, finite element analysis methods using concrete plasticity models are excellent tools [27]. However, in order to estimate accurately the load–deformation behavior of axially loaded concrete members confined with materials having different constitutive relationships, simpler models may be preferred by structural engineers in the preliminary design of these members. At the heart of the problem remains the realistic description of the behavior of concrete subjected to triaxial compression. Once this is established any passive or active confining mechanism can be simulated. The objective of this research is to provide one such model and to verify it for steel and FRP confined concrete subjected to axial compression. It is believed that the proposed model can be useful in the preliminary design of steel confined columns or in the fiber reinforced polymer retrofit design of deficient columns.

2. Model description

           First, the phenomenological model is described in this section. The model is a global representation rather than a local one for axially loaded concrete subjected to constant confinement. Then, the procedure for obtaining the behavior of columns with passive confinement (columns provided with steel or fiber reinforced polymers) by satisfying lateral strain compatibility between concrete and the reinforcing jacket for the axial loading history is presented. Since the model can be thought of as a single element representation of concrete subjected to compression, the strains used below are average quantities over the specimen rather than actual strains. After localization starts, strain distribution along the specimen height is no longer uniform [28]. Instead of tracing this non-uniform strain distribution, average strain values are used in the description below such that average stress–strain behavior of a confined column can be obtained.

2.1. Axial deformations

           For the present discussion, axial and lateral directions are denoted by 1 and 3, respectively. It is assumed that stresses and strains are similar in the two lateral directions (σ2 ≈ σ3, ε2 ≈ ε3). Furthermore, compressive stresses and strains are assumed to be positive whereas volume expansion is taken as negative. The axial stress–strain response of concrete confined by constant lateral pressure can be described using three distinct locations on the stress–strain curve (Fig. 1):

(1) The elastic limit up to which concrete is assumed to be isotropic and linear elastic (σ1e, ε1e).

(2) The ultimate strength which is a function of lateral pressure, (σ10, ε10).

(3) The residual capacity remaining as a result of internal friction, (σ1r).

چکیده
1. مقدمه
2. توضیحات مدل
2.1. تغییر شکل محوری
2.2. تغییر شکل عرضی
2.3. محاسبات برای بتن محصور ثابت و غیر فعال
3. تایید مدل
3.1. محصوری ثابت
3.2. بتن محصور با فولاد
3.3. بتن محصور شده توسط FRP 
4. مطالعه پارامتری
5. خلاصه و نتیجه گیری
منابع

Abstract
1. Introduction
2. Model description
2.1. Axial deformations
2.2. Transverse deformations
2.3. Calculations for constant and passive confined concrete
3. Model verification
3.1. Constant confinement
3.2. Steel confined concrete
3.3. FRP confined concrete
4. Parametric studies
5. Summary and conclusions
References