دانلود رایگان مقاله تست ارتعاش یک ساختمان 5 طبقه با قاب فولادی

چکیده

        این مقاله روند طراحی میراگرهای ویسکوالاستیک و نتایج آزمون تجربی یک سازه های فلزی 5 طبقه تک دهانه با میراگر ویسکوالاستیک را ارائه می دهد. خواص مکانیکی میراگر ویسکوالاستیک و ویژگی های دینامیکی ساختار مدل، از طریق آزمایش و با استفاده از تحریک هارمونیک به دست آمد. نتایج این آزمایش در روند طراحی مورد استفاده قرار گرفته است. در ابتدا، نسبت میرایی اضافی لازم، برای کاهش حداکثر پاسخ سازه به یک سطح مورد نظر به دست آمد. سپس به اندازه لازم میراگر برای رسیدن به نسبت میرایی مورد نیاز، با استفاده از روش انرژی کرنشی مودها و با مشاهده تغییرات در نسبت میرایی مودها و با توجه به تغییر در سختی میراگر تعیین شد. میراگر ویسکوالاستیک در طبقه ی اول و دوم مدل نصب شده است. نتایج حاصل از آزمایشاتی که از روش نویزتصادفی با باند محدود و یا هارمونی استفاده می کنند، نشان داد که پس از نصب میراگر های، پاسخ دینامیکی مدل سازه ای با مقیاس واقعی تا میزان مطلوب راحی کاهش یافته است.

1. مقدمه

         میراگرهای ویسکوالاستیک (VED) در کاهش پاسخ دینامیکی ساختمان ها، در وزش باد و یا تحریک لرزه ای بسیار موثر هستند، و به صورت عملی در برج های دوقلوی مرکز تجارت جهانی در نیویورک برای کاهش ارتعاشات ناشی از باد استفاده شده اند. بسیاری از تحقیقات به استخراج مدل تحلیلی برای VED و به منظور بررسی اثر آن بر کنترل ساختاری از طریق آزمایش [1-4] پرداخت اند. مطالعات قبلی نشان داد که VED می توانید میرایی سازه را به طرز قابل توجهی افزایش دهد، که در نتیجه ی آن شاهد کاهش پاسخ سازه، مانند جابجایی و شتاب مطلق خواهیم بود. همچنین نتایج حاصل از این مطالعات نشان داد که عملکرد VED بستگی به عواملی مانند فرکانس تهییج و دمای محیط بستگی دارد. لذا عملکرد VED باید با در نظر گرفتن این عوامل مورد بررسی قرار گیرد. دربسیاری از آزمایشات، سازه در مقیاس کوچک و با  VED کوچک، مورد استفاده و بررسی قرار گرفت است و تنها چند تحقیق با استفاده از VED در مقیاس واقعی شده انجام شده است.

        موضوع دیگر مربوط به استفاده های عملی از VED ، توسعه روش طراحی VED برای رسیدن به سطح پاسخ سازه ای مطلوب است. برای این هدف، یک تصمیم حساب شده و با در نظر گرفتن تمامی عوامل طراحی مانند تعداد، اندازه، محل بهینه و روش نصب، لازم است. در بسیاری از مطالعات، VED با اندازه های دلخواه نصب شده و تاثیر آن بر کنترل ارتعاش مورد بررسی قرار گرفته است. ژانگ و همکارانش یک روش مرحله ای برای جایگذاری بهینه  VED با استفاده از مفهوم درجه ی قابلیت کنترل [5] ارائه داده اند. لی و همکارانش محل و اندازه VED را با استفاده از روش انتساب قطبها [6]ارائه دادند. این مطالعات نشان داد که قرارگیری VED در موقعیتی که بیشترین جابجایی داخل طبقه را دارد، بهترین و موثرترین گزینه است. چانگ و همکارانش یک راهنما برای طراحی سازه های دارای قاب فولادی با  VED ارائه دادند. آنها برای ارائه ی این راهنما از نتایج مطالعات قبلی استفاده کرده، و اثربخشی روش طراحی را با انجام یک آزمایش با مدل سازه ی در مقیاس 2/5 مورد بررسی قرار دادند و پس از بررسی نتایج آن را تائید کردند [7]. اگر چه این روش طراحی، توضیحات دقیق ارائه داده و اثر بخشی آن را از طریق مطالعه تجربی تایید می کند، اما مطالعات تجربی بیشتری برای استفاده ی عملی از آن مورد نیاز است. به خصوص، آزمایش در مقیاس واقعی، می تواند توضیحات دقیق و واقعی ارائه داده و نتایج آن می تواند به عنوان یک پایه و اساس برای استفاده واقعی VEDدر ساختمان مورد استفاده قرار گیرد.

       در این مطالعه، بر اساس نتایج حاصل از آزمون سیکلی المان های میراگر و نتایج حاصل از آزمون شناسایی سیستم ساختمان 5 طبقه ی با مقیاس واقعی، و با استفاده از میز لرزه ی دوگانه (HMD) واقع در طبقه پنجم، VED برای برآوردن حد مطلوب و مورد نظر، طراحی شد. نسبت میرایی اضافی مورد نیاز برای کاهش پاسخ دینامیکی سازه تا سطح مطلوب، برای اولین بار توسط مدل محدب به دست آمد [8]. اندازه میراگر با استفاده از روش انرژی کرنشی مودها، با استفاده از نسبت میرایی مودها مورد نیاز تعیین شد [7]. سختی مهاربندها نیز در تعیین خواص مودها در نظر گرفته شد. سپس سازه ی دارای VED در دو طبقه ی پایینی که دارای بیشترین جابجایی داخل طبقه بود، برای بررسی اثر آن بر کنترل ارتعاش آن مورد آزمایش قرار گرفت. ساختار توسط HMD که نیروهای سینوسی و باند محدود تولید می کند، بارگذاری و تهییج شد. تنها پاسخ الاستیک خطی در نظر گرفته شده و از اثر متقابل خاک-سازه و پاسخ پیچشی چشم پوشی شده است.

2. ویژگی های مواد ویسکو الاستیک

        طراحی VED نیاز به دانش کافی راجع به خواص مکانیکی ماده ویسکوالاستیک دارد. خواص ماده ویسکوالاستیک مبتنی بر متغیرهایی مانند دما، فرکانس تحریک و فشار می باشد، بنابراین، اثر این متغیرها بر خواص مکانیکی VED توسط آزمایش مورد بررسی قرار گرفت. نمونه آزمون نشان داده شده در شکل 1 از دو لایه از مواد ویسکوالاستیک تشکیل شده است، که هر لایه 10 میلی متر ضخامت و 150 سانتی مربع مربع مساحت دارد. در نمونه ها ده آزمایش سیکلی و  با استفاده از تحریک سینوسی با 0.5 هرتز در دمای 24 درجه ی سلسیوس و با مقادیر متفاوتی از کرنش 20، 25، 50، 75، و 100٪ انجام شد. جدول 1 نتایج آزمون سختی باقیماند و فاکتور کاهش که برای کرنش های مختلف بدست آمده را نشان می دهد. همچنین این جدول بیان می کند که عامل کاهش کرنش به تغییرات کرنش ماکزیمم بستگی نداشت. علاوه بر این، ده آزمون سیکلی نیز با استفاده از تحریک سینوسی در 24 در دمای 24 درجه ی سلسیوس با فرکانس های مختلف و ماکزیمم کرنش انجام شد. نتایج آزمون در جدول 2 نشان داده شده که بیان می کند که سختی باقیمانده و عامل کاهش کرنش  به میزان قابل توجهی به فرکانس تحریک بستگی دارد. در نهایت رفتار وابسته به دما، توسط یک سری از آزمایشات در دماهای مختلف با فرکانس تحریک 0.5 هرتز و کرنش ها 20 و 50٪ بررسی شد. جدول 3 نشان می دهد که فاکتور کاهش بسیار حساس به دما است. شکل 2 نشان می دهد که مدول برشی باقیمانده با افزایش فرکانس تحریک افزایش می یابد.

3. پیکربندی آزمایش

3.1  سازه ی مدل برای انجام تست

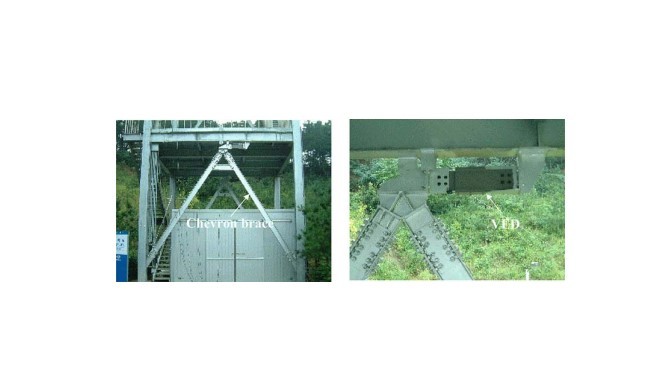
        مدل تجربی نشان داده شده در شکل 3، سازه در مقیاس اصلی دارای پنج طبقه اسکلت فلزی و با ارتفاع طبقه 6 متر، اندازه ی 6 متر در 6 متر، و وزن طبقه ی 20 تن است. هر طبقه از چهار ستون فولادی با تیرآهن بال پهن یکسان تشکیل شده است. HMD نشان داده شده در شکل 4، در طبقه پنجم و با منظور تحریک مدل سازه نصب شده است. شکل. 5 محل VED و شتاب سنج را نشان می دهد.

3.2  شناسایی سیستم

        بعد از تحریک سازه توسط بازدارنده های HMD،  از طریق سنجش پاسخ ارتعاش آزاد سیستم بدست آمد که فرکانس پایه 0.5 هرتز می باشد. با توجه به اینکه جرم طبقات معیین بوده و سختی طبقات بطور یکسان توزیع گردیده بود، میزان سختی طبقه قابل تخمین بود و میزان آن 2440 kN/m برآورد گردید. سپس آنالیز مقادیر ویژه برای ساختمان واقعی انجام گرفت. برای انجام ارزیابی، ساختمان، برشی و با درجه آزادی 5 فرض شده و همچنین وزن هر طبقه متمرکز بر روی کف طبقه در نظر گرفته شد. از آنالیز مقادیر ویژه، 5 فرکانس طبیعی بدست آمد: 0.51، 1.46، 2.3، 2.95 و3.37. برای به دست آوردن نسبت میرایی مودی تجربی، بارگذاری سینوسی با محدوده فرکانس 0.4-0.8 هرتز با میزان افزایش 0.05 هرتز در هر دفعه، استفاده شد. در نزدیکی فرکانس طبیعی پیش بینی شده، میزان افزایش فرکانس در هر دفعه کاهش می یابد. شکل 6 تابع انتقال شتاب در بالاترین طبقه، که در آن فرکانس طبیعی پایه ای 0.50 هرتز و نسبت میرایی 1.98٪ مشاهده شد، را نشان می دهد. برای بدست آورن نسبت میرایی در این طبقه از روش پهنای باند نیم توان استفاده شد. در تجزیه و تحلیل لرزه ای، فاکتورهای شرکت پذیری جرم مودها، از طبقه ی اول تا پنجم به ترتیب، 87.95%، 8.72%، %2.42، %0.75، و %0.16، می باشد که نشان دهنده ی غالب بودن مود اول نسبت به سایر مودهاست. 

Abstract

        This paper presents a design process for viscoelastic dampers and experimental test results of a 5-storey single bay steel structure with added viscoelastic dampers. The mechanical properties of viscoelastic dampers and the dynamic characteristics of the model structure were obtained from experiments using harmonic excitation, and the results were used in the design process. The additional damping ratios required to reduce the maximum response of the structure to a desired level were obtained first. Then the size of dampers to realize the required damping ratio was determined using the modal strain energy method by observing the change in modal damping ratio due to the change in damper stiffness. The designed viscoelastic dampers were installed in the first and the second stories of the model structure. The results from experiments using harmonic and band limited random noise indicated that after the dampers were installed the dynamic response of the full-scale model structure reduced as desired in the design process. # 2004 Elsevier Ltd. All rights reserved.

1. Introduction

        Viscoelastic dampers (VED) are highly effective in mitigating the dynamic responses of building structures due to wind or seismic excitation, and practically were used in the twin towers of the World Trade Center in New York for the reduction of wind-induced vibrations. Many researches have been conducted to derive analytical models for VED and to verify the effect on structural control through experiments [1–4]. Previous studies showed that VED can increase structural damping significantly, which brings the decrease of structural responses, such as displacement and absolute acceleration. The results of these studies also indicated that the performance of VED depends on factors such as excitation frequency and environmental temperature, and so the effects of VED should be evaluated by considering these factors. However, in most experiments, small-scale model structures with scaled VED were used, and only few experimental researches were conducted which verified the effectiveness of VED in a fullscale model structure.

        Another issue related to practical applications of VED is the development of the design procedure for VED to achieve a prescribed structural response level. For this purpose, a systematical decision of such design factors as number, size, optimal location, and installation method is required. In many studies, however, VED of arbitrary sizes were installed and their vibration control effect was observed. Zhang et al. proposed a sequential procedure for optimally placing VED based on the concept of degree of controllability [5]. Lee et al. determined the location and the size of VED by using pole assignment method [6]. These studies showed that placing VED at the position with the largest inter-storey displacement is most effective. Chang et al. presented a design guideline for steel frame structures with VED, based on the results of previous studies, and verified the effectiveness of the design procedure by conducting a experiment for 2/5-scale structural model [7]. Although this design procedure provides detailed prescription and its effectiveness is verified through experimental study, further experimental studies are required for practical application. Especially, through full-scale experiment, many design considerations can be provided and the results will be a basis for structural application of VED.

        In this study, based on the results of cyclic tests of damper elements and on the results of system identification tests of a 5-storey full-scale model structure vibrated using the hybrid mass driver (HMD) located on the fifth floor, VED were designed to satisfy a given target response. The additional damping ratios required to reduce the maximum dynamic response of the structure to a given level were obtained first by convex model [8]. The size of dampers was determined using the modal strain energy method which uses the required modal damping ratio [7]. The stiffness of the supporting braces was also considered in the determination of the modal properties. Then the structure with the VED installed in the lower two stories which have the largest inter-storey displacement was tested to validate their vibration control effect. The structure was excited by the HMD which generates sinusoidal and band-limited excitation forces. Only linear-elastic response was considered and soil-structure interaction and torsional response were neglected.

2. Properties of viscoelastic materials

       The design of VED requires the knowledge of the mechanical properties of viscoelastic material. The properties of the viscoelastic material depend on variables such as temperature, excitation frequency, and strain, and therefore, the effect of these variables on the mechanical properties of VED were investigated by experiments. Test specimens shown in Fig. 1 were made of two layers of viscoelastic materials, each layer 10 mm in thickness and 150 cm2 in area. Ten cyclic tests were conducted on the specimens by using sinusoidal excitation with 0.5 Hz at the temperature of 24 v C with various maximum strains of 20, 25, 50, 75, and 100%. Table 1 presents the test results for storage stiffness and loss factor obtained for different strains, and shows that the loss factor was not sensitive to the maximum strain. In addition, ten cyclic tests were also conducted by using sinusoidal excitation at 24 v C with various forcing frequencies and maximum strains. Test results shown in Table 2 indicate that storage stiffness and loss factor significantly depend on excitation frequency. Finally temperature-dependent behavior was investigated by a series of tests at various temperatures with excitation frequency of 0.5 Hz and strains of 20 and 50%. Table 3 shows that the loss factor is very sensitive to temperature. Fig. 2 shows that the shear storage modulus increases with increasing excitation frequency.

3. Experimental setup

3.1. Model structure for experiment

       The experimental model, which is shown in Fig. 3, is a full-scale five-storey steel structure with storey height of 6 m, plan of 6  6 m, and storey mass of 20 ton. Each floor is composed of four identical wide-flange type steel columns. A HMD shown in Fig. 4, is installed on the fifth floor to excite the model structure. Fig. 5 shows the location of VED and accelerometers.

3.2. System identification

       The fundamental frequency was found experimentally to be 0.50Hz by investigating the free vibration response after the excitation by the HMD stops. Since each storey mass was known and storey stiffness was uniformly distributed, the storey stiffness could be estimated, which was 2440 kN/m. Then, eigenvalue analysis was performed for the full-scale structure. For the analysis, the structure was assumed to be a shear building with 5 DOF, and that the weight of each storey was concentrated on the floor. Five natural frequencies were identified from eigenvalue analysis: 0.51, 1.46,2.30, 2.95, and 3.37 Hz. To obtain the modal damping ratio experimentally, sinusoidal loadings with frequency ranges from 0.4 to 0.8 Hz were applied to the structure at the increment of 0.05 Hz. Near the predicted natural frequency the increment was further reduced. Fig. 6 plots the transfer function for the acceleration of the top storey, from which the fundamental natural frequency of 0.50 Hz was observed again and the corresponding damping ratio of 1.98% was obtained by using half power bandwidth method. In the case of seismic analysis, modal mass participation factors in terms of percentage of the 100 metric ton total mass for first to fifth mode are, respectively, 87.95, 8.72, 2.42, 0.75, and 0.16%, which indicates that the first mode predominates over other modes.

چکیده

1. مقدمه

2. ویژگی های مواد ویسکو الاستیک

3. پیکربندی آزمایش

3.1  سازه ی مدل برای انجام تست

3.2  شناسایی سیستم

4. تخمین نسبت میرایی مورد نیاز

5. تخمین نسبت میرایی مورد نیاز

6. نتایج آزمایشات

7. خلاصه

منابع

Abstract

1. Introduction

2. Properties of viscoelastic materials

3. Experimental setup

3.1. Model structure for experiment

3.2. System identification

4. Determination of required damping ratio

5. Design of viscoelastic dampers

6. Experimental results

7. Summary

References