دانلود رایگان مقاله آنالیز قابلیت اطمینان برای بتن تقویت شده

خلاصه 

     در این مقاله به بررسی عدم قطعیت های موجود در داده های هندسی و خواص مربوط به بتن تقویت شده در سازه ها، بر مبنای طراحی مهندسی لرزه ای خواهیم پرداخت. قابلیت اطمینان، به کمک روش های مختلف مانند آنالیز استاتیکی پوش آور که بر پایه المان محدود می باشد ارزیابی شده است. روش های مورد استفاده برای بررسی قابلیت اطمینان شامل کوپل کامل با کد المان محدود خارجی و پاسخ سطح خواهد بود که بر اساس قابلیت اطمینان مرتبه اول و روش نمونه سازی بنا شده اند. احتمال واماندگی با توجه به روش های مورد استفاده به دست آمده است. در این بررسی آنالیز همگرایی نیز انجام شده است. طول مدت انجام آنالیز به عنوان تابعی از روش مورد استفاده در نظر گرفته شده است. نتیجه به این صورت بود که زمان برای روش کوپلینگ، بیشتر از روش پاسخ سطح بود. برای سازه با بتن تقویتی که مد نظر این مقاله بوده است، متغیر های مهمی در روشهای مورد نظر وجود دارند. پیشنهاد ما در این مقاله روش کوپل کامل می باشد، اما روش قابلیت اطمینان مرتبه اول که مربوط به مدل پاسخ سطحی است نیز می تواند مورد استفاده قرار گیرد که دقت بالایی نیز خواهد داشت.

1 – مقدمه

     مدل سازی واقعی بر اساس آنالیز قابلیت اطمینان در ساختمان هایی با ریسک حوادثی مانند زمین لرزه، موضوعی جالب برای طراحان سازه های لرزه ای می باشد. لیل و همکاران [ 16 ]، مولر و همکاران [ 19 ]، پیلوسو و همکاران [ 22 ]، بوراتی و همکاران [ 2 ] و کلیک و الین وود [ 3 ]. در تمامی تحقیقات در این زمینه، عیب یابی و نو سازی سازه های قدیمی مانند موزه ها، از مهم ترین دلایل تحقیقات می باشد. سایر دلایل، مرتبط با مهندسی بر پایه عملکرد می باشند. 

     مهندسی بر پایه عملکرد، موفقیت های فراوانی را در زمینه زمین لرزه بدست آورده است. علاوه بر تنظیم کلاسیک و کدهای لرزه ای، که به منظور تضمین امنیت ساکنین ایجاد شده اند، این روش شامل حالت های بحرانی دیگری می شود که در طراحی ساختمان از اهمیت زیادی برخوردارند. این حالت های عملکردی وابسته به شاخص هایی مانند میزان تحمل خرابی می باشند. پیش بینی این حالت های عملکردی به صورت پارامترهایی است که ساکنین و مالکین ساختمان بتوانند از سطح خطری که با آن مواجه هستند آگاه باشند. بدین منظور، مدل سازی عددی رفتار سازه ای و انتشار عدم قطعیت مورد نیاز است. این موارد به طور کلی در چهارچوب آنالیز قابلیت اطمینان انجام می شوند. عدم قطعیت های ایجاد شده در مسئله، ناشی از خواص مواد، ابعاد هندسی و یا نیروهای اعمالی می باشد. این پارامترها به عنوان پارامترهای اتفاقی شناخته می شوند. همچنین عدم قطعیت ها می توانند ناشی از عدم اطلاعات کافی از مقادیر پارامترهای مختلف در سازه باشند.

     امروزه پیش بینی رفتار پیچیده سازه به صورت گسترده و با استفاده از روش المان محدود صورت می گیرد. به عنوان مثال، این روش به منظور ارزیابی گسترش ترک در تحقیقات سویا و همکاران [ 3 ] استفاده شده است. در حضور عدم قطعیت ها که پارامترهای سازه را تحت تاثیر قرار می دهند، می توان از روش های المان محدود اتفاقی نیز استفاده کرد. داده های ورودی برای محاسبات المان محدود، با متغیرهای اتفاقی در تعامل می باشند تا تغییرات ماده، هندسه و بارگذاری را به خوبی نشان دهند. توسط مدل سازی انتشار عدم قطعیت، مانند فرایند monte carlo، نتایج حاصل خواهند شد. آنالیز قابلیت اطمینان المان محدود روشی است که آنالیز المان محدود اتفاقی را با برخی توابع عملکردی ترکیب می کند. تابع عملکرد، وابسته به مقادیر پاسخ آنالیز المان محدود بوده و تابعی ضمنی از داده های ورودی می باشد. تابع عملکرد فضای داده را به دو ناحیه تقسیم می کند : ناحیه امن و ناحیه واماندگی. تا زمانی که تابع عملکرد به صورت صریح شناخته نشده باشد و فرایند monte carlo نیز زمان بر باشد، جستجوی نقطه طراحی توسط روش های آنالیز قابلیت اطمینان انجام می شود. 

     مروری بر کوپل بین آنالیز قابلیت اطمینان FORM و روش المان محدود در تحقیقات کیرگیان [ 4 ] انجام شده است. همچنین در این تحقیق، نتایج حاصل از بررسی های لیو [ 17 ]، ژانگ [ 5 ]، هالدار [ 11 ]، ایمای [ 14 ]، سودرت [ 25 ]، فرانکین [ 7 ] و سورنسن [ 8 ] نیز استفده شده اند.آنالیز المان محدود قابلیت اطمینان با استفاده از کوپل بین یک کد المان محدود و روش هایی مانند FORM یا monte carlo، با وجود اینکه محاسباتی زمان بر هستند ولی مورد استفاده قرار می گیرند. این نیز بدین دلیل است که در هر تکرار، تابع حدی و مشتقات آن توسط محاسبات المان محدود بدست می آیند. روشی موثر که FORM و نمونه سازی را در نقطه واماندگی ترکیب می کند توسط هاکاس و کیرگیان ارایه شده است. در روش نمونه گیری ISM، تعداد محدودی از ارزیابی های تابع حدی، برای بدست آوردن نقطه تقریبی استفاده می شود. هاکاس و کیرگیان، مثال های عددی شامل مدل های جامع المان محدود غیر خطی با 500 متغیر اتفاقی که بیانگر حالت همگرایی ISM می شود را ارایه کردند. 

2 – مورد مطالعه

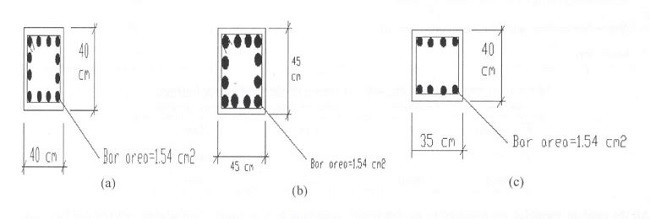
     به منظور ارزیابی روش های مختلف برای محاسبه عدم قطعیت ها در چهارچوب آنالیز قابلیت اطمینان المان محدود در محیط OPENSEES، یک سازه 4 طبقه با بتن تقویت شده در نظر گرفته شده است. در این سازه، آنالیز پوش آور غیرخطی استاتیکی به منظور تعیین عملکرد لرزه ای کافی می باشد. ارتفاع هر طبقه 3 متر در نظر گرفته شده است. شکل 1 ارتفاع عمودی و نمای صفحه ای را نشان می دهد. شکل 2 نشان دهنده مقاطع بتن به همراه تقویت کننده هاست که با استفاده از EUROCODE2 محاسبه شده است. جدول 1 نشان دهنده بارهای عمودی گره ها بر حسب KN می باشد و جدول 2 نیز بارهای جانبی لرزه ای را بر حسب KN نشان می دهد. 

     استحکام نهایی لایه خارجی بتن و همچنین مدول های یانگ فولاد تقویتی، به صورت متغیرهای تصادفی مدل شده اند. این پارامترها در مدل المان محدود به صورت ذاتی و غیر قابل کاهش خواهند بود. سایر پارامترهای مربوط به ماده، هندسه و بارگذاری نیز در نظر گرفته شده اند. جدول 3 مقادیر اسمی پارامترهایی مانند خواص مواد که در آنالیز قابلیت اطمینان سازه RC مورد استفاده قرار می گیرند را نشان می دهد. جدول 4 نیز نشان دهنده مدل سازی عدم قطعیت متغیرهای اتفاقی می باشد. 

4-نتیجه گیری

     نشان داده شد که آنالیز قابلیت اطمینان کوپل کامل، نتایجی مشابه با نتایج پاسخ سطح تقریبی را نخواهد داشت. این آنالیز در حالت کلی احتمال واماندگی را پیش بینی می کند. حداکثر اختلاف نسبی بین این روش ها 41.5 درصد می باشد. این مقدار اختلاف نیز بین روش های کوپل/FORM و RSM/ISM خواهد بود. در هر دو حالت، نتایج روش monte carlo بسیار دقیق تر از نتایج روش FORM خواهد بود. به طور کلی روش FORM، واماندگی را به صورت محتاطانه تر تقریب می زند. نتایج بدست آمده نشان می دهند که آنالیز قابلیت اطمینان کوپل کامل بهتر از آنالیز FORM عمل می کند زیرا که آنالیز FORM واماندگی را به صورت اغراق آمیز نشان می دهد. روش ISM همیشه زمان بر تر از روش FORM بوده است. در مقایسه این روش ها، روش سطح پاسخ نیاز به نیروی کار اضافی دارد. تاثیر داده های انتخابی بر روی مدل در آنالیز قابلیت اطمینان کوپل کامل بسیار مهم می باشد. این اثر در آنالیز قابلیت اطمینان پاسخ سطح کاهش می یابد. به منظور انجام آنالیز لرزه ای، باید در انتخاب پارامترهای عدم قطعیت دقت بیشتری به خرج داد. تنها استفاده از استانداردها کافی نبوده وPDF نیز باید مشخص شود. در حین استفاده از آنالیز قابلیت اطمینان پاسخ سطح، نشان داده شد که در اینجا نیز روش FORM، احتمال واماندگی را در مقایسه با روش ISM به صورت اغراق آمیزی نشان می دهد. از این نتایج می توان برای سایر مسائل مرتبط با آنالیز قابلیت اطمینان استفاده کرد. همچنین آنالیز کاملی برای ارزیابی عملکرد سایر روش ها مورد نیاز است.

Abstract

     Accounting for uncertainties that are present in geometric and material data of reinforced concrete buildings is performed in this work within the context of performance based seismic engineerin g design .  Reliability of the expected performance state is assessed by using various methodologies based on finite element nonlinear static pushover analysis and specialized reliability software package. Reliability approaches that were considered included full coupling with an external finite clement code and surface response based methods in conjunction with either first order reliability method or importance sampling method . The probability of failure according to the used reliability analysis method and to the selected distribution of probabilities was obtained. Convergence analysis of the importance sampling method  was performed. The required duration of analysis as function of the used reliability method was evaluated. It was found  that reliability results are sensitive to the used reliability analysis method. Durations of analysis for coupling methods were found to be higher than those associated to surface response based methods ; one should however include time needed to derive these lasts. For the reinforced concrete building considered in this study, it was found that significant variations exist between all the considered reliability methodologies. The full coupled importance sampling method is recommended , but the first order reliability method applied on a surface response model can be used with good accuracy.

1.Introduction

     Realistic modeling based on reliability analysis of structural behavior of buildings at risk of earthquake events is the subject of increasing interest from the community of seismic building designers Liel el al. [ 16), Moller el al. [ 19), and Piluso et al.[22), Buratti et al. [2], Celik and Ell ingwoodf3l . Among the reasons beyond  the  intensive  research activity in this field, one finds the huge need for diagnosis and rehabilitation of pre-code constructions, particularly in the case of historic monuments . Other reasons arc associated to the emergence of new design approaches which are founded on the concept of performance-based engineering.

     Performance-based  engineering  has  gained  large  success  in  the  field  of earthquake  engineering . Instead  of the classical regulatory and non-transparent seismic code rules which were elaborated to ensure essentially a priori life safety of buildings occupants, this new approach includes additional critical states that could be important for buildings use. These performance states are associated to indicators such as the tolerable amount of damage or the accepted economic loss resulting from temporarily loss of functionality .

     Predicting  these  performance  states  is  considered  in  terms  of  probabilities  such  that  occupants  or  owners  of buildings could be aware of the risk level they are undergoin g. To realize that , adequate numerical modeling of the building structural behavior and satisfactory description of uncertainty propagation arc required . This is generally performed within the framework of reliability analysis . Uncertainties arising in the problem could be the result of the inherent randomness in material characteristics , geometric dimensions or applied forces. These categories of uncertainties are termed stochastic parameters. But, uncertainties could be also epistemic such as those due to lack of knowledge regarding the real values of some parameters in existing constructed buildings : reinforcement sections in structural members or junctions features that exist between columns and beams .

Predicting  the  real  complex  behavior  of structures  is  nowadays  largely  performed  by  using  the  finite element method, as an example this method is used to assess crack propagat ion Souiyah et al [3], and Alshoaibi et al [3]. In the presence  of uncertainties  affecting structural  model  parameter s, stochastic finite clement has been  introduced . The  input  data  for  the  finite  element  computation  are  dealt  with  as  random  variables  to  depict  the  uncertain variations  present  in  the material , geometry  and  loading  parameters.  Through  uncertainty  propagating  modeling such  as  Monte  Carlo  process  the  resulting  probability  of  response  events  could  be  computed.  Finite  element reliability  analysis is a technique that combines stochastic finite element analysis with some performance  function defining a given limit-state. The performance  function depends on response quantities of the finite element analysis, and comes out to be an implicit function of the input data. The performance  function separates the data space into two regions: the safe region  and the failure region . The probability  of failure  is linked  to the minimum  distance separating the actual design realization from the most probable failure point layin g on the limit surface, called also the design  point.   Since the performance  function  is not  explicitly  known  and  Monte Carlo process  is too time consuming,  search  of the design  point  is  performed  habitually  through  various  approximate  reliability  analysis methods .

In the first and second order reliability  methods (FORM and SORM) the  limit-state is approximated, at the most likely failure point in the transformed space of uncorrelat ed standard normal  random variables , by respectively a hyper-plane  and a  paraboloid.  A  review  of coupling  between  FORM  reliability  analysis  and  the finite element method can be found in Der Kiureghian[4]. Other significant contributions have since been presented . They include developments due to Liu and Der Kiureghianl 17], Der Kiureghian and Zhangl5], Haldar and Mahadevan [11), Imai and Frangopol[l 4), Sudrct and Der Kiurcghian [25), Franchin[7),  and Frier , and Sorcnsen[8).

     Finite element reliability analysis using full coupling between a finite element code and reliability methods such as FORM or Monte Carlo tends however to be hi gh computational time consuming for practical problems that include large number of random variables . This is so becau se, at any iteration , the limit-state function and its derivatives are to be evaluated through finite element computations. An effective method which combines FORM and subsequent importance samplin g around the most probable failure point has been proposed by Haukaas and Der Kiureghian[l 2). The Importance Sampling Method (ISM) requires only a limited number of evaluations of the limit-state function (and its gradient with respect to the random variables) to find the approximation point , followed by efficient importance sampling analysis centered at this point. Haukaas and Der Kiureghianl 12) have presented numerical examples involving comprehensive nonlinear finite clement models with approximately 500 random variables that state convergence of ISM.

2.Case of study

     In order to evaluate the different methodologies introduced to account for uncertainties within  the framework  of finite element reliability analysis under OpenSees environment, a four-story reinforced concrete building structure is considered. It consists of a regular building for which the nonlinear static pushover analysis  is sufficient to assess seismic performance. The inter story height  is fixed at 3 m . The bay length  in both seismic directions is fixed at 4 m . Fig. I gives the vertical elevation and the plane view. Fig.2 gives concrete sections of members with their reinforcements as computed by using  Eurocode  2 code. Table  I the vertical  load  resultants  at the  structural  nodes  in kN. Table 2 the seismic l ateral  loads in kN.

     The  ultimate  strengths  of  outer  layer  of concrete  and  of the  core  concrete  as  well  as  Young's  modulus  of the reinforcement steel are modeled as random variables. These are intended to model the inherent, irreducible uncertainty in the finite element model parameters. All the other material and geometric parameters as well as loads will be considered to be deterministically known. Table 3 gives the deterministic nominal  parameters  values  of material data that were used during reliability analysis of the RC structure. Table 4 defines the uncertainty modeling of the random variables.

4. Conclusions

     It has been shown that the full coupling reliability analysis does not predict the same results than the approximate response surface based reliability method. This last underestimates in general the probability of  failure.  The maximum relative difference between these various methodologies  results has reached 41.5%. This occurs between the Coupling/FORM and the SRM/ ISM methods. Within the framework of the same methodology  of reliability analysis (either full coupling or response surface), the approximate method FORM does not give the same results than the more precise modified Monte Carlo ISM Method. In  general,  FORM  overestimates  the  probability  of failure. The obtained results have shown that full coupling reliability analysis conducted with ISM is recommended because FORM analysis could exaggerate sometimes the probability of failure. It is remarkable to observe  the antagonist effect resulting from the association of SRM/ FORM because this method gives results that are closer to the more exact Coupling/ISM. The ISM method is always more time consuming than FORM approximation.  To make an objective comparison regarding computational cost, one should recall also that response surfaces must be identified and that additional labor is required for that. Influence on reliability data of the chosen PDF's to model uncertainties is very significant when considering full coupling  reliability  analysis.  This effect  reduces  however when surface response based reliability analysis is performed . In order to perform reliability analysis of seismic performance based design, huge care should be given  to selection of PDF's  that model  parameters  uncertainties (these should be is general identified through experiments). It is not sufficient to give only means and standard deviations, the PDF's must also be specified. When applying surface  response  based  reliability  analysis, it  was shown that FORM approximation overestimates here again the probability of failure in comparison  with the accurate ISM. These conclusions could not be generalized without precautions to other problems dealing with reliability analysis and thorough analysis is needed to assess performance of the various methodologies .

خلاصه 

1 – مقدمه

2 – مورد مطالعه

3 – نتایج

روش های قابلیت اطمینان کوپل کامل FORM و ISM

روش های قابلیت اطمینان FORM و ISM اعمالی به مدل سطح پاسخ سازه 

4- نتیجه گیری

Abstract

1.Introduction

2.Case of study

3.Results

Full reliability coupling FORM and ISM methods

FORM and ISM reliability methods applied to a response surface model of the building

4. Conclusions

References