دانلود رایگان مقاله کمانش الاستیک پوسته های بیضوی کاسینی تحت فشار خارجی با شاخص های شکل متفاوت

چکیده

           این مطالعه به بررسی اثر شاخص شکل بر کمانش الاستیک پوسته‌های بیضوی کاسینی تحت فشار خارجی یکنواخت می‌پردازد. پوسته‌ها دارای ضخامت دیوار یکنواخت (2 میلیمتر) و ظرفیت (3.63 × 106 میلی متر)، با شاخص شکل، kc = c / a، در محدوده‌ی 0 تا 99، ارزیابی می‌شوند. چندین محاسبات عددی شامل تجزیه و تحلیل انشعابی الاستیک خطی کمانش و تجزیه و تحلیل کمانش غیر خطی هندسی با استفاده از عیوب خاص برای این پوسته‌ها انجام می‌شود. برخی از آنها به صورت آزمایشی مورد بررسی قرار می‌گیرند. نتایج نشان می‌دهد که یک پوسته‌ی بیضوی کاسینی با یک مولفه‌ی پایدار به دلیل کم بودن ظرفیت باربری، به نظر می‌رسد که یک شکل نامطلوب است، که با نتایج قبلی در ارتباط با این مسئله در تضاد است. نکته قابل توجه این است که هندسه بیضوی کاسینی با ابعاد kc = 0.1، دارای ظرفیت باربری بالایی، به شکل مطلوب در زمینه‌های مختلف مانند تانک های زیر آب، پوسته‌های تحت فشار و کپسول‌های مصنوعی می‌باشد.

1. مقدمه 

           پوسته‌های دورانیِ تحت فشار یکنواخت خارجی، به علت ویژگی‌های حمل بار موثر خود، برای مدت زمان طولانی مورد تحقیق واقع شده‌اند. آنها در زمینه‌های مختلف مهندسی مانند موشک‌های فضایی [1]، کپسول‌های مصنوعی [2]، واحدهای شناور [3]، مخازن تحت فشار [4،5] و مخازن زیرزمینی [6] استفاده می‌شوند. پیکربندی‌هایی که به صورت گسترده‌تری مورد استفاده قرار می‌گیرند، عبارتند از کره‌ها [7،8]، سیلندر‌ها [9،10]، مخروط‌ها [11،12] و ترکیب آنها [13]. پوسته‌های غیر معمول مانند بیضی‌ها [14]، بشکه‌ها [15،16]، تخم مرغی شکل‌ها [17،18] و بیضی کاسینی  [6] نیز مورد بررسی قرار گرفته‌اند. با این حال، مشخص شده است زمانی که این پوسته‌ها در معرض فشار خارجی یکنواخت قرار می‌گیرند، مستعد کمانش هستند که اغلب به آسیب غیر قابل تعمیر می‌انجامد؛ کمانش مخازن به شدت تحت تأثیر شکل هندسی آن، ضخامت دیواره و خواص مواد و همچنین نقص‌های اولیه هندسی [19-21] قرار دارد. 

           یکی از معیارهای بهبود ایمنی تغییر شکل پوسته است. مطالعات متعددی بر پوسته‌های غیر معمول دورانی با انحنای گاوس  مثبت یا منفی متمرکز شده است. به عنوان مثال، بلاچوت  مطالعات عددی و تجربی را با موضوع کمانش الاستیک-پلاستیک مجموعه‌ای از پوسته‌های دورانی شبیه به قوس‌های دایره‌ای [22] و بیضی‌های متعارف [23]، که همچنین با پوسته‌های استوانه‌ای معادل آن مقایسه شده است، انجام داده است. اخیرا برای رفع معایب پوسته‌ی مخازن کروی، مانند حساسیت بالای عیوب، ضعف ساده سازی و کم بودن میزان بهره وری از فضا، رفتارهای خمشی پوسته‌های تخم مرغی شکل [17] و همچنین اثر شاخص شکل در کمانش [18] بیشتر مورد مطالعه قرار گرفت. علاوه بر این، جاسیون  و مگنوکی  مطالعات عددی و تحلیلی با موضوع کمانش الاستیک پوسته‌های بیضوی کاسینی [6]، پوسته‌های کروی [24] و پوسته‌های کمان دایره‌ای [25] انجام دادند. جاسیون و مگنوکی متوجه شدند که مسیرهای متعادل برخی از پوسته‌های بیضوی کاسینی دارای کاراکتر پایدار [6] بوده است که برای بارها و صفحات الاستیک شایع است. آنها پیشنهاد کردند که پوسته‌های بیضی به صورت یک شکل مطلوب که برای ساختار تحت فشار قرار می‌گیرند، ظاهر شوند و این پوسته‌های بیضوی کاسینی می‌توانند در حین کمانش و در مراحل بعد از کمانش، ایمنی را تضمین کنند. با این حال، تنها پوسته‌های بیضوی کاسینی با شاخص‌های شکل در محدوده‌ی (0.6-0.9) مورد بررسی قرار گرفتند. افزون بر این، مقایسه بین پوسته‌هایی که بر اساس همان ظرفیت و جرم بود، موجب ایجاد ضخامت دیواره‌های مختلف برای هر پوسته شد، که ممکن است متقاعد کننده بودن نتایج را کاهش دهد، زیرا بیشتر مقایسه‌های مربوطه بر اساس همان ظرفیت و ضخامت دیواره یا همان جرم و ضخامت دیواره است [15،25].

           بنابراین، مطالعه‌ی حاضر بر کمانش الاستیک پوسته‌های بیضوی کاسینی با شاخص‌های شکل در محدوده‌ی 0-0.99، که تحت فشار خارجی هستند، متمرکز شده است. فرض شده است که پوسته‌ها از یک ماده‌ی مشابه، ظرفیت و ضخامت دیواره‌ی یکسان تشکیل شده‌اند. بقیه‌ی این مقاله به شرح زیر است: بخش 2 تعریف هندسه، بار، ظرفیت، ضخامت دیواره، مواد و مدل عددی پوسته‌های بیضوی کاسینی را ارائه می‌دهد. در بخش 3، رفتار خطی الاستیک خطی پوسته‌های بیضوی کاسینی و کمانش الاستیک غیرخطی پوسته‌های ناقص براساس تعداد محاسبات عددی ارائه می‌شود؛ اثر شاخص شکل بر روی کمانش نیز ارائه شده است. محاسبات نشان داد که پوسته‌های بیضوی کاسینی با یک کاراکتر پایدار دارای ظرفیت بار کم هستند. به طور مشخص، یک پوسته بیضوی کاسینی با kc = 0.1، ظرفیت بارگذاری بالاتر و حساسیت عیوب کمتری را نسبت به یک پوسته کروی در زمینه‌ی کمانش الاستیک و نسبت ضخامت به اندازه ضخامت عدسی، از خود نشان داد. این مشاهدات تا اندازه‌ای برای مدل‌های مقیاس آزمایشگاهی و محاسبات مربوطه تایید شده است.

2. تعریف مسئله

1.2 ویژگی‌های هندسی

یک پوسته بیضوی کاسینی (شکل 1 (a)) را با محور نیمه اقطر، xe، محور نیمه اقصر، r0 و ضخامت دیوار یکنواخت t، تحت فشار برخی فشارهای یکنواخت p0، در نظر بگیرید. 

           در این مطالعه، پوسته‌های بیضوی کاسینی با kc <0.99 > 0 با ضخامت دیواره 2 t =  میلیمتر و ظرفیت v = 3.63 × 106 میلی متر با استفاده از معادله‌ی (5) مورد بررسی قرار گرفتند. شاخص‌های شکل، پارامترهای معادله و محورهای نیمه اقطر و نیمه اقصر به ترتیب در جدول 1 آورده شده است. برای تسهیل ساخت مدل‌های مقیاس آزمایشگاهی با استفاده از روش سریع ساختن نمونه‌ی اولیه (RP)، فرض شد که پوسته‌ها از رزین حساس به نور ساخته شده‌اند. خواص مواد به عنوان الاستیک؛ مدول یانگ E = 2510MPa؛ نسبت پواسون μ = 0.41، در نظر گرفته شد.

2.2 محاسبات عددی 

           مجموعه‌ای از محاسبات عددی برای پوسته‌های بیضوی کاسینی انجام شده است که شامل تحلیل خطی الاستیک از هندسه‌ی کامل و تحلیل الاستیک هندسی غیر خطی با عیوب شامل هندسه‌ی ناقص می‌باشد. تجزیه و تحلیل قبلی با استفاده از روش تکرار زیرمجموعه انجام شد در حالی که بعدا به روش طول قوس پاسخ داد. تمام محاسبات با استفاده از سیستم عددی محدود آباکوس  مطابق با ENV 1993-1-6 (2007) انجام شد [27]. عنصر پوسته S4 کاملا یکپارچه برای جلوگیری از اثر ساعت شنی استفاده شد. مطالعات همگرایی پوسته‌ها در تجزیه و تحلیل خطی الاستیک خطی انجام شد [15،17،18،24]، در نتیجه مجموع 9600 المان برای مدل عددی است.

ABSTRACT

           This study examines the effect of shape index on the elastic buckling of Cassini oval shells under uniform external pressure. Shells are evaluated under a uniform wall thickness (2 mm) and capacity (3.63 10 × 6 mm3 ), with the shape index, k c c = /a, ranging from 0 to 0.99. Several numerical computations, involving linear elastic bifurcation analysis and geometrically nonlinear elastic analysis including eigenmode imperfections, are conducted for these shells. Some of them are investigated experimentally. The results show that a Cassini oval shell with a stable character seems to be an unfavorable shape due to its low load-carrying capacity, which is at variance with previous findings regarding this problem. Notably, the kc = 0.1 Cassini oval geometry, exhibiting a high load-carrying capacity, appears to be a favorable shape in various fields such as underwater tanks, pressure hulls, and artificial capsules.

1. Introduction

           Shells of revolution under uniform external pressure have long generated considerable research interest due to their effective loadcarrying properties. They are applied in various engineering fields such as space launch vehicles [1], artificial capsules [2], buoyancy units [3], pressure hulls [4,5], and underground tanks [6]. The most extensively adopted configurations are spheres [7,8], cylinders [9,10], cones [11,12] and their combinations [13]. Atypical shells such as ellipsoids [14], barrels [15,16], eggs [17,18], and Cassini ovals [6] have been explored as well. However, these shells have been found to be prone to buckling when subjected to uniform external pressure, which often results in an unrecoverable collapse; the buckling of a vessel is heavily affected by its geometrical shape, wall thickness, and material properties, as well as the initial geometric imperfections [19–21].

           One means of improving safety is to change the meridional shape of the shell. Numerous studies have focused on atypical shells of revolution with positive or negative Gaussian curvature. For instance, Blachut has performed a numerical and experimental study of the elastic–plastic buckling of a set of shells of revolution resembling circular arcs [22] and generalized ellipses [23], which were also compared with equivalent cylindrical shells. More recently, to resolve the disadvantages of spherical pressure hulls, such as high imperfection sensitivity, poor streamlining, and low space utilization rate, the buckling behaviors of egg-shaped shells were further studied [17], as well as the effect of shape index on buckling [18]. In addition, Jasion and Magnucki have carried out a numerical and analytical study of the elastic buckling of Cassini oval shells [6], clothoidal-spherical shells [24], and circular arc shells [25]. Notably, Jasion and Magnucki found that the equilibrium paths of some Cassini oval shells had a stable character [6], which is typical for elastic bars and plates. They suggested that the Cassini oval shells appeared to be a favorable shape for an externally pressurized structure, and that the Cassini oval shells could ensure safety both during buckling and in the postbuckling stages. However, only Cassini oval shells with shape indices within a limited range (0.6–0.9) were considered. Moreover, a comparison among shells that was based on the same capacity and mass led to a different wall thickness for each shell, which might reduce the convincingness of conclusions because most relevant comparisons were based on either the same capacity and wall thickness or the same mass and wall thickness [15,25].

          Therefore, the current study focused on the elastic buckling of externally pressurized Cassini oval shells with shape indices within the range of 0–0.99. The shells were assumed to be of the same material, capacity, and wall thickness. The rest of this paper is organized as follows. Section 2 presents the definition of the geometry, load, capacity, wall thickness, material, and numerical model of Cassini oval shells. In Section 3, the linear elastic buckling behaviors of perfect Cassini oval shells and the nonlinear elastic buckling of imperfect shells are proposed on the basis of a number of numerical computations; the effect of shape index on buckling is also proposed. The computations revealed that Cassini oval shells with a stable character had a low loadcarrying capacity. Notably, a Cassini oval shell with kc = 0.1 exhibited a higher load-carrying capacity and lower imperfection sensitivity than a spherical shell in the case of elastic buckling and small eigenmode imperfection size-to-wall thickness ratio. This observation was partially validated for laboratory scale models and corresponding computations.

2. Problem definition

2.1. Geometrical properties

           Consider a Cassini oval shell (Fig. 1(a)) with a semimajor axis, xe, a semiminor axis, r0, and a uniform wall thickness, t, subjected to some uniform external pressure, p0

          In this study, Cassini oval shells with 0 < < kc 0.99 were evaluated with the same wall thickness t = 2mm and capacity v = × 3.63 10 mm 6 3, directly determined using Eq. (5). The shape indices, equation parameters, and semimajor and semiminor axes were determined as listed in Table 1. To facilitate fabricating the laboratory scale models using rapid prototyping (RP) technique, it was assumed that shells were made of photosensitive resin. The material properties were assumed to be elastic, as follows: Young's modulus E = 2510MPa; Poisson's ratio μ 0.4 = 1.

2.2. Numerical computations

         Serials of numerical computations were carried out for Cassini oval shells, involving linear elastic bifurcation analysis of perfect geometry and geometrically nonlinear elastic analysis with imperfections included of imperfect geometry. The former analysis was performed using the subspace iteration method whilst the later corresponded to the arc length method. All computations were conducted using the ABAQUS finite element system in line with ENV 1993-1-6 (2007) [27]. A fully integrated S4 shell element was employed to prevent hourglassing effect. Convergence studies of shells were carried out in the linear elastic bifurcation analysis [15,17,18,24], resulting in a total of 9600 elements for the numerical model.

چکیده

1. مقدمه 

2. تعریف مسئله

2.1 ویژگی‌های هندسی

2.2 محاسبات عددی 

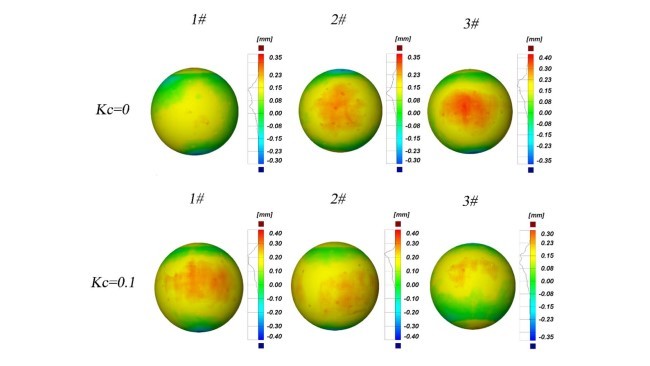
3. نتایج و بحث

3.1 تجزیه و تحلیل انشعاب الاستیک خطی

3.2 تجزیه و تحلیل الاستیک غیر خطی به صورت هندسی با نواقصی که شامل می‌شود. 

3.3 آزمایش

4.3 تحلیل عددی پوسته‌های آزمایش شده

4. نتایج 

منابع

ABSTRACT

1. Introduction

2. Problem definition

2.1. Geometrical properties

2.2. Numerical computations

3. Results and discussion

3.1. Linear elastic bifurcation analysis

3.2. Geometrically nonlinear elastic analysis with imperfections included

3.3. Experimentation

3.4. Numerical analysis of tested shells

4. Conclusions

References