دانلود رایگان مقاله مدل های شار نشت ترانسفورماتور برای ناپایداری های الکترومغناطیسی

چکیده

     این مقاله شواهدی تجربی از اعتبارسنجی مدل پیوندی ترانسفورماتور القایی نشت ارائه می کند. نشان داده شده که این رویکرد پیوندی نتایج مشابهی را روش ماتریس پذیرش نامحدود BCTRAN دارد. یک مدل ترانسفورماتور صحیح سه مرحله ای ارائه شده است. نقطۀ اتصال میان نشت و اندوکتانس مغناطیسی به درستی شناسایی شده است، که این مدل جدید را با ارائه پیوستگی فیزیکی نسبت به BCTRAN و مدل های ترکیبی برتری می دهد. علاوه بر این، تأیید تجربی یک روش برای محاسبۀ اندوکتانس های اتصال کوتاه ارائه شد. توضیحات جدید تقسیم شار نشت و همبستگی ریاضیاتی میان  T و مدل های معادل نیز ارائه شده است.

مقدمه

     دقیق ترین مدل های ترانسفورماتور برای ناپایداری های الکترومغناطیسی دارای فرکانس پایین (زیر سیم پیچ اولین شدت فرکانس، معمولاً چند کیلوهرتز ([1]، بخش 1)) مبنایی فیزیکی دارند. در این مدل ها، شار مغناطیسی به داخل مسیرهای از پیش تعریف‌شده‌ای به نام تیوب های شار محدود می شود. این مدل ها را توپولوژیکی می گویند، زیرا هر عنصر این مدل بخشی از مقاومت مغناطیسی را در مسیر فیزیکی میدان مغناطیسی نشان می دهد. این مدل ها به جای مدل های میدان برداری در برنامه های نوع ناپایداری های الکترومغناطیسی کاربرد دارند، زیرا هزینه های محاسباتی موجود در شبیه سازی های FEM به سه دلیل بازدارنده هستند: 1) ماهیت ناپایدار این پدیده، که برای هر مرحلۀ زمانی به یک راه حل میدانی نیاز دارد؛ 2) غیرخطی بودن هسته های ترانسفورماتور؛ و 3) نیاز به مدل بندی نه فقط یک ترانسفورماتور بلکه چند عدد از آنها (با توجه به تنظیمات سیستم مورد بررسی).

     با وجودی که مدل های اخیر که در آثار پیشین ارائه شده اند، توپولوژیکی هستند و مبنایی فیزیکی دارند، اما می توان دید که برای تنظیمات یک ترانسفورماتور مشخص، مدل های توپولوژیکی بسیار متفاوتی وجود دارد. برای مثال، برای ترانسفورماتور هسته ای سه پایه سه فاز (با دو یا سه سیم پیچ)، فهرست کوتاهی از مدل ها در ]2 شکل 5-5[، ]3 شکل 4-18[، ]4 شکل 3[، ]5 شکل 2-15[، ]6 شکل 2[، ]6 شکل 6 (ب)[ و ]8 شکل 3[ ارائه شده است. اگر مبنای این مدل ها فیزیکی باشد، چرا مدل های توپولوژیکی بسیار متفاوتی برای نوع مشابه ترانسفورماتور وجود دارد؟ پاسخ این است: به خاطر شار نشت.

     از لحاظ نظری، اگر نارساناهای مغناطیسی وجود داشته باشند، شار مغناطیسی می تواند کاملاً به رساناهای مغناطیسی محدود شود، مانند جریان های الکتریکی در فرکانس های پایین. تفاوت کلیدی میان جریان های الکتریکی و مغناطیسی تفاوت موجود در ارزش-های نسبی نفوذپذیری مغناطیسی و رسانایی میان رساناها و غیر رساناها است. ازآنجاکه نسبت نفوذپذیری های روغن (یا هوا) و آهن در محدودۀ 103-104 قرار دارد، برخی از شارهای مغناطیسی از هستۀ مغناطیسی نشت می کنند، درحالی که نسبت رسانایی هوای خالص (یا پلیمر) و مس به ترتیب 1022-1028 است. با توجه به [9]، یافتن یک مدار مغناطیسی معادل با شار نشت مشابه راه اندازی یک مدار الکتریکی معادل برای شبکه ای از رساناهای مسی موجود در محلول الکترولیت است. از این رو، مسیرهای انتخابی (یا دقیق تر تیوب های شار) و نقاط اتصال نشت ها به مدار می تواند از یک مدل به مدل دیگر متفاوت باشد.

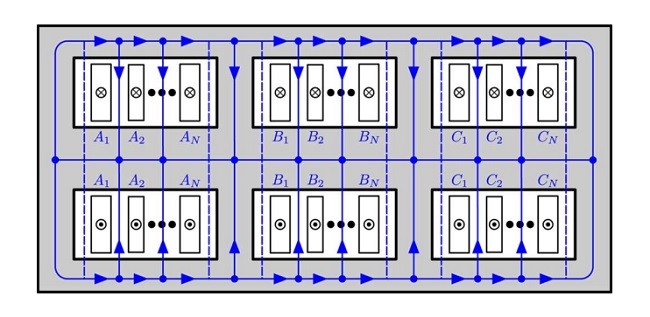
     علی رغم این حقیقت که اختلافات به خاطر انتخاب خاص مسیرهای شار وجود دارند، تفاوت کلیدی دیگر از این حقیقت ناشی می-شود که برخی از مدل ها شار نشت را میان جفت سیم پیچ های دو شار تقسیم می کنند، درحالی که سایر مدل ها این کار را انجام نمی-دهند. این مورد را می توان در ]10، شکل 7 و 8[ مشاهده کرد، که در آن شار نشت تقسیم می شود، در مقایسه با ]6، شکل 1 و 2[، که تقسیم نشده است. این تفاوت در مدل سازی شار نشت در ]5% فصل های 1 و 2[ برجسته شده، که در آن دو رویکرد به ترتیب با نام های رویکرد شار انتگرال و رویکرد شار تقسیم شده وجود دارد. اما هر دو رویکرد در بخش دو توضیح داده می شوند.

     مسئلۀ موجود در مدل های توپولوژی عدم موفقیت آنها در نمایش شرایط مدار اتصال کوتاه برای ترانسفورماتورهای دارای دو سیم-پیچ یا بیشتر است (11، بخش 3). برای مدل های توپولوژیکی ترانسفورماتورهای N-سیم پیچ بر مبنای رویکرد شار انتگرال، اندوکتانس های نشت N-1 وجود دارد، و برای آنهایی که بر مبنای رویکرد شار تقسیم شده هستند، اندوکتانس های نشت N موجود می باشد. ازآنجاکه N(N-1)/2 متفاوتی از ترکیبات مدار اتصال کوتاه وجود دارد، می توان مشاهده کرد که این رویکرد ها در نمایش همۀ موارد موفق نیستند (آنها تنها می توانند برای تعداد مشخصی از سیم پیچ های N کار کنند).

     برای کاهش این مسئله، در (4)، (12) و (13) پیشنهاد شده که از رساناهای پیوندی استفاده کنیم (یا شبکه معادل مش) تا مجدداً جفت های شرایط مدار اتصال کوتاه N(N-1)/2 را تولید کنیم، که این ماتریس ورودی نامحدود می تواند با روش ارائه شده در (14) (BCTRAN) محاسبه شود. بااین‌حال، نمایش اندوکتانس نشت دیگر توپولوژیکی نیست، تنها راه حلی برای تولید مجدد و دقیق مقیاس‌های مدار اتصال کوتاه است. این مورد در (13، صفحۀ 77) برجسته شده است. برای اتصال مدل هسته ای توپولوژیکی به ماتریس ورودی مدار اتصال کوتاه، به یک سیم پیچ ساختگی یا بیشتر نیاز داریم، که بخشی از ورودی مدار اتصال کوتاه با این رویکرد تقسیم می شود. این رویکرد در (4) و (15) مدل ترکیبی نامیده می شود تا بر این حقیقت تأکید کند که از مدل هسته ای توپولوژیکی همراه با نمایشی صحیح تر از ورودی مدار اتصال کوتاه استفاده می کند.

      هرچند این رویکرد ترکیبی مسئلۀ عدم کفایت میان‌ مقیاس‌های مدار اتصال کوتاه نهایی و ارزش های محاسبه‌شده را با مدل های نشت توپولوژیکی حل می کند، اما مسائل دیگری را نیز مطرح می کند. اول، به چه تعداد سیم پیچ ساختگی نیاز است؟ با توجه به (12) و (16)، دو سیم پیچ ساختگی وجود دارد α و β، درحالی که در (4) تنها یکی وجود دارد. دوم، برای بررسی این سیم پیچ ها ورودی مدار اتصال کوتاه چگونه تقسیم می شود؟ فاکتور تناسب K از (4)، برای اشتقاق "اندوکتانس نشت" میان سیم پیچ داخلی و سیم پیچ ساختگی، در (17) به صورت K=0.5 پیشنهاد می شود. در (16)، ارزش K=0.33 از فرمول‌های نمودار کلاسیک آمپر-دور (یا برگشت جریان) محاسبه می شود، فرض بر این است که سیم پیچ ساختگی بی نهایت نازک است. بااین‌حال، در (18) نشان داده شده که اگر شار محوری نباشد، این فرمول ها می توانند نادرست باشند. علاوه بر این، در (12) فاکتور پیوندی δ=10^3 برای جفت کردن ماتریس ورودی مدار اتصال کوتاه در سیم پیچ ساختگی به کار می رود. سوم، در (17) ادعا شده که: هستۀ مصنوعی سیم پیچ به کانال نشت میان سیم پیچ فیزیکی داخلی و هسته مربوط می شود." بااین‌حال، در مورد طراحی سیم پیچ ساندویچی کدام سیم پیچ، سیم پیچ داخلی است؟ این مسائلی که در اینجا مطرح شد تناقضاتی فیزیکی را نشان می دهد که در این رویکرد وجود دارد.

Abstract

     This paper presents experimental validation of the coupled leakage inductance transformer model. It is shown that the coupled approach yields the same results as the indefinite admittance matrix method of BCTRAN. A topologically correct three-phase shell-type transformer model is proposed. The connection points between the leakage and magnetizing inductances are properly identified, which makes the new model superior to BCTRAN and the hybrid models by providing physical consistency. In addition, experimental verification of a method to calculate the short-circuit inductances is presented. New explanations on the division of leakage flux and on the mathematical equivalence between the T- and -equivalent models are also given.

I. INTRODUCTION

     THE MOST accurate transformer models for low-frequency electromagnetic transients (below the winding first resonance frequency, typically a few kilohertz ([1], Section 1)) have a physical basis. In these models, the magnetic flux is confined inside predefined paths called flux tubes. These models are called topological, since each model element represents a part of the reluctance in the magnetic-field physical path. These models are used in electromagnetic-transients (EMT)-type programs instead of vectorial field models, because the computational cost involved with FEM simulations would be prohibitive due to three facts: 1) the transient nature of the phenomenon, which would require computing a field solution for each time-step; 2) the nonlinearities of transformer cores; and 3) the need to model not just one transformer but several of them (depending on the system configuration being studied).

     Even though the more recent models proposed in the literature are topological and, thus, physically based, it can be seen that for a given transformer configuration, many different “topological” models exist. For instance, for the three-phase threelegged stacked-core transformer (with two and three windings), a shortened list of models is given by [2, Fig. 5.5], [3, Fig. 4.18], [4, Fig. 3], [5, Fig. 2.15], [6, Fig. 2], [7, Fig. 6(b)], and [8, Fig. 2]. If the models are physically based, why are there so many different topological models for the same transformer type? The answer is: because of leakage flux.

     In theory, if magnetic insulators existed, the magnetic flux could be entirely confined within the magnetic “conductors,” as is the case for electric circuits at low frequencies. The key difference between electric and magnetic circuits is the difference in the relative values of magnetic permeability and conductivity between conductors and insulators. Since the ratio of permeabilities of oil (or air) and iron is in the range of – , some of the magnetic flux leaks from the magnetic core, whereas the ratio of conductivities of pure air (or polymers) and copper is in the order of – . According to [9], finding an equivalent magnetic circuit with leakage flux is similar to deriving an equivalent electric circuit for a network of copper conductors immersed in an electrolyte solution. Hence, the chosen paths (or flux tubes, to be more precise) and the points of connection of leaks to the circuit can vary from one model to another.

     Despite the fact that discrepancies exist due to the particular choice of flux paths, another key difference comes from the fact that some models divide the leakage flux between a pair of windings into two fluxes, while others do not. This can be seen in [10, Figs. 7 and 8], where the leakage flux is divided, compared to [6, Figs. 1 and 2], where it is not divided. This difference in the modeling of leakage flux is highlighted in [5, Chs. 1 and 2], where the two approaches were, respectively, called the integral flux approach and divided flux approach. Both approaches are explained in Section II.

     The main problem with those topological models is their failure to adequately represent short-circuit conditions for transformers with more than two windings (see [11, Sec. III]). For topological models of -winding transformers based on the integral flux approach, there are leakage inductances, and for those based on the divided flux approach, there are leakage inductances. Since there are different pairs of short-circuit combinations, it can be seen that these approaches will fail to represent all cases (they can work only for a given number of windings ).

     To alleviate this problem, it was proposed in [4], [12], and [13] to use coupled inductors (or a mesh-equivalent network) to reproduce the pairs of short-circuit conditions, where the indefinite admittance matrix can be calculated with the method proposed in [14] (BCTRAN). However, this representation of leakage inductances is no longer topological, it is just a solution for reproducing more accurately the terminal short-circuit measurements. This is highlighted in [13, p. 77]. In order to connect a topological core model to the short-circuit admittance matrix, one or more fictitious windings are necessary, where a portion of the short-circuit admittance is divided with this approach. This approach was called the hybrid model in [4] and [15] to emphasize the fact that it uses a topological core model with a more appropriate representation of short-circuit admittances.

      Even though the hybrid approach solves the problem of inadequacy between terminal short-circuit measurements and the values calculated with topological leakage models, it raises other issues. First, how many fictitious windings are required? According to [12] and [16], there are two fictitious windings and , whereas there is only one in [4]. Second, how should the short-circuit admittance be split to account for these windings? The proportionality factor of [4], to derive a “leakage inductance” between the inner winding and the fictitious winding, is proposed to be 0.5 in [17]. In [6], a value of 0.33 is calculated from classical ampere-turn diagram formulas, assuming that the fictitious winding is infinitely thin. However, it was shown in [18] that these formulas can be inaccurate if the flux is not axial. Furthermore, in [12], a coupling factor is used to couple the short-circuit admittance matrix to the fictitious winding. Third, it is mentioned in [17] that: “The artificial core winding is related to the leakage channel between the inner physical winding and the core.” However, which winding is the inner winding in the case of a sandwiched winding design? The issues raised here show the physical inconsistencies that exist with this approach

چکیده

1-مقدمه

2-شارهای انتگرال و تقسیم شده

3-تبدیل ستاره-مثلث

4-رویکرد شار نشتی پیوندی

5-روش ها

6-نتایج

7-بحث

8-نتیجه گیری

Abstract

I.Introduction

II.Integral and Divided Fluxes

III.Star-Delta Transformation

IV.Coupled Leakage Approach

V.Methods

VI.Results

VII.Discussion

VIII.Conclusion