دانلود رایگان مقاله مقایسه مدل های آشفتگی مختلف برای جریان ناپایدار در اطراف یک سیلندر

چکیده

     این مقاله عملکرد هشت رینولدز-میانگین ناییر استوکس (RANS) ، دو معادله مدل های آشفتگی و دو مقیاس زیر شبکه (SGS)  مدلهای شبیه سازی  غلتکی بزرگ(LES)  در سناریوی جریان ناپایدار در اطراف سیلندر دایره ای محدود در یک نسبت جنبه AR) )   1. 0 و تعداد رینولدز  s 20000 Re = مشخص شده را مقایسه می کند. متوجه شدیم که، در میان همه هشت مدل آشفته بررسی شده   RANS ، مدل K-Omega-SST (یعنی SST-V2003) توسط منتر و همکاران [1، 2] توسعه یافت که دارای بهترین عملکرد کلی (نزدیکترین به نتایج عددی دو مدل LES  بررسی شده است که می تواند به عنوان راه حل تقریبا دقیق با توجه به  مشی محاسباتی بسیار خوب  توسط هر دو مدل LES در این مطالعه مورد استفاده قرار می گیرد) براساس توزیع ضریب فشار متوسط (ip Cp)، میانگین ضریب کشش (یعنی Cd)، میانگین پروفایل ساده در برخی از صفحه های مشخص (مانند ارتفاع متوسط صفحه  و صفحه تقارن سیلندر) و توزیع میانگین استحکام تنش- برشی- لایه ای در دیوار پایین می باشد .

1.مقدمه

      مطالعه تجربی و عددی  در ساختارهای جریان سه بعدی پیچیده در اطراف جسم غیرباریک یکی از فعال ترین زمینه های تحقیق در پویش اساسی سیال  دهه های گذشته باقی می ماند، عمدتا به دلیل حضور گسترده چنین جریان هایی در طبیعت و برنامه های کاربردی مهندسی، مانند میدان باد در اطراف ساختمان های بلند، حمل و نقل آلودگی در اطراف پشته دودکش ، نیروی آیرودینامیک در برج های خنک کننده، میدان جریان در اطراف سازه های دریایی، تبادل گرما در صفحه مدارهای  الکترونیکی و غیره می باشد  [3-6] اگر چه بسیاری از مطالعات پیشین بر روی تجزیه و تحلیل  سیلندر مربعی یا مدار دو بعدی   اسماٌ نامحدود جریان گذشته (2D) تمرکز می کند، به تازگی بیشترین توجه به جریان ناپایدار در اطراف سیلندرهای کم ارتفاع محدود [7-12]، با یک انتها در جریان آزاد (یعنی جریان بسته آزاد) غوطه ور و انتهای دیگر بر روی یک دیوار صاف نصب می شود (یعنی. پایه پایانی)، که با واقعیت سازگار تر است.  به همین ترتیب، با توجه به اثر ترکیبی  جریان نفوذ آب از انتهای آزاد و لایه مرزی در نزدیکی پایین دیوار، ساختار جریان سه بعدی (3D) در اطراف سیلندر محدود معمولا بسیار  پیچیده تر از پشت یک بی نهایت است.

      می توان از پیشینه تحقیق موجود نتیجه گیری کرد که تمام شش عامل زیر می توانند اثرات بر ساختار جریان در اطراف سیلندر ارتفاع کم  باشند  [6-8]: 1). شدت آشفتگی نزدیک شدن جریان؛ 2) شکل مقطع سیلندر؛ 3) شماره Re (یعنی Re = UD / ν،  که D عرض مشخصی از سیلندر است، U - سرعت جریان آزاد است و ν - ویسکوزیته سینماتیک سیال است)؛ 4) ضخامت مرز لایه در دیوار پایین نسبت به ارتفاع سیلندر (یعنی δ / h)؛ 5) نسبت ارتفاع سیلندر به عرض مشخصی سیلندر (یعنی AR = h / D)؛ 6)  نسبت انسداد کانال (یعنی β1 = h / H و β2 = D / B،  که H و B  به ترتیب  ارتفاع و عرض کانال یا دامنه محاسباتی می باشد). از یک طرف، میزان اثر مربوطه  عوامل فوق الذکر ممکن است بطورقابل توجهی از یکدیگر متفاوت باشند زمانیکه آن به شرایط خاص می رسد. از سوی دیگر، با توجه به عوامل تاثیرگذار متعدد و میدان جریان پیچیده  تحت این شرایط در گذشته، اکثرا محققان فقط در مورد هر یک از عوامل تاثیرگذار در هر مقاله برای ساده سازی مشکل بحث می کنند، بنابراین، اثر یکپارچه به طور همزمان تغییر چند ین پارامترها مورد بررسی در آینده باقی می ماند.

       با توجه به اینکه تعداد رینولدز بالا همیشه منجر به  ساختار بسیار پیچیده  در آگاهی یک سیلندر دایره ای محدود می شود (به ویژه هنگامی که روی پایین دیوار غیر لغزش نصب شده است)، این مقاله بحث مفصلی  در رابطه با سیلندر دایره ای محدود با AR = 1 را در تعداد نسبتا بالا Re  نشان می دهد (یعنی 20000). هدف این مطالعه بطور کمی و کیفی مقایسه دقیق مدل های مختلف آشفتگی می باشد زمانیکه آن برای مشخصات میانگین ضریب فشار متوسط Cp درسطح ارتفاع سیلندر، میانگین ضریب کششی Cd سیلندر، میانگین-زمانی حوزه سرعت و فشار و توزیع میانگین تقویت استرس برشی تیرگی در دیوار پایین فرا می رسد.

2.پیکربندی و مدل عددی

 2.1 تست پیکربندی

      همانطور که پیکربندی مشابه هندسی توسط ژانگ و همکاران استفاده می شود[6,8] در این مطالعه نیز مورد استفاده قرارمی گیرد. همانطورکه در شکل 1 نشان داده شده است، یک سیلندر دایره ای محدود با عرض غیر-ابعادی D = 1 و ارتفاع غیر ابعادی h = 1  بطور عمودی بر روی یک مرزصفحه نصب شده است، و طول (جریان) عرض (عرضی) و (تقاطع) ارتفاع دامنه محاسباتی به ترتیب L = 30D، W = 22D و  H = 2D  هستند (که نسبت مسدود شدن مساحت را به 2.27٪ می دهد).  علاوه بر این، بخش اتصال بین سیلندر و دیوار پایین در مبدأ سیستم مختصات قرار دارد بدین معناست که مرز ورودی در 10D بالادست سیلندر و مرز خروجی در جریان پایین دست سیلندر  D 20  قرار دارد  (یعنی L1 = 10D، L2 = 20D).

2.2معادلات حاکم

2.2.1مدل های RANS . معادلات نینیرد استوکس (RANS)

      میانگین-رینولدز 3D  ناپایدار  در این مطالعه  استفاده شده است  که می تواند با استفاده از میانگین توده  جرم لحظه ای  و معادلات حفاظت شتاب حرکت برای جریان های تراکم ناپذیر و ایزوترمال حاصل شود، همانطور که در زیر نشان داده شده است.

3.2 شرایط مرزی و برنامه های عددی

چهار نوع شرایط مرزی درگیر هستند:

       1. ورودی: برای میدان سرعت، یک سرعت ثابت یکنواخت تجویز  (یعنی  I u =1, I v = I w =0) و، برای میدان فشار، شرایط صفر گرادیان اعمال می شود (یعنی 0 I p n  )

       2. خروجی: برای میدان سرعت، شرایط جریان مرزی  همرفتی تصویب می شود: که برکلیه سه مولفه سرعت دلالت دارد (یعنی،u,v,w (، و c u سرعت پیشرونده در مرز خروجی می باشد.  برای میدان فشار، شرایط همگن  دریشلت همگن مرز خروجی استفاده می شود (یعنی p = 0)

       3. دیواره پایین و سطح مانع: شرایط مرزی بدون نفوذ بدون لغزش برای مقادیر سرعت (یعنی u=v=w=0 ) تجویز شده و شرایط شیب صفر برای میدان فشار استفاده می شود. 

     4. مرزهای بالا و فرعی دامنه محاسباتی: شرایط لغو آزاد تجویز می شود بدین معنی است که مولفه سرعت طبیعی به مرز صفر است (یعنی u   =0 )  و گرادیان عادی هر دو فشار و مولفه سرعت مماسی صفر هستند (یعنی ub/ / n pb n 0 ,  که u b / /  مولفه سرعت مماسی است). 

Abstract

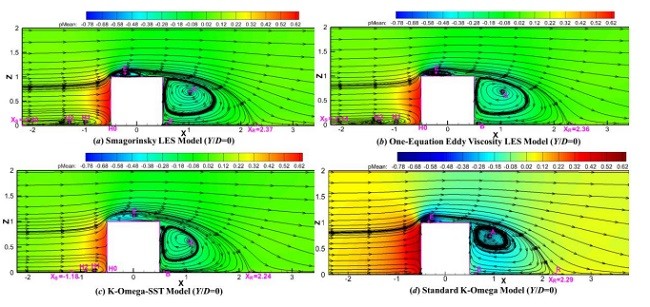
      This paper compares the performance of eight Reynolds-Averaged Navier–Stokes (RANS) two-equation turbulence models and two sub-grid scale (SGS) large eddy simulation (LES) models in the scenario of unsteady flow around a finite circular cylinder at an aspect ratio (AR) of 1.0 and a Reynolds number of Re=20000. It is found that, among all the eight RANS turbulence models considered, the K-Omega-SST model (viz. SST-V2003) developed by Menter et al.[1, 2] possesses the best overall performance (being closest to the numerical results of the two LES models considered, which can be deemed as the quasi-exact solution in view of the very fine computational mesh employed by the two LES models in this study) in terms of the mean surface pressure coefficient distribution (i.e. Cp), the mean drag coefficient (i.e. Cd), the mean streamline profiles in some characteristic planes (such as the mid-height plane and the symmetry plane of the cylinder) and the distribution of mean bed-shear-stress amplification on the bottom wall.

1. Introduction

      The experimental and numerical study on the complex three-dimensional flow structures around a bluff body remains one of the most active areas of research in fundamental fluid dynamics over the past decades, mainly due to the extensive presence of such flows in nature and engineering applications, such as the wind field around high-rise buildings, the pollutant transport around chimney stacks, the aerodynamics force on cooling towers, the flow field around offshore structures, the heat exchange on electronic circuit boards, and so on [3-6]. Although many earlier studies focused on the analysis of the flow past a nominally infinite two-dimensional (2D) circular or square cylinder, recently most attentions have been paid to the unsteady flow around finite-height cylinders [7-12], with one end immersed in the free stream (viz. the free end) and the other end mounted on a flat wall (viz. the base end), which are more consistent with the structures in reality. Correspondingly, due to the combined influence of the downwash flow from the free end and the boundary layer near the bottom wall, the three-dimensional (3D) flow structure around a finite cylinder is usually much more complicated than that behind an infinite one.

      It can be concluded from the existing literature that all the following six factors can have some effects on the flow structure around a finite-height cylinder [6-8]: 1). the turbulence intensity of the approaching flow; 2). the cross-section shape of the cylinder; 3). the Re number (viz. Re=UD/ν, where D is the characteristic width of the cylinder, U is the free stream velocity, and ν is the fluid’s kinematic viscosity.); 4). the boundary-layer thickness on the bottom wall relative to the cylinder height (viz. δ/h); 5). the ratio of the cylinder height to the characteristic width of the cylinder (viz. AR=h/D); 6). the blockage ratio of the channel (viz. β1=h/H and β2=D/B, where H and B are the height and width of the channel or the computational domain, respectively.). On one hand, the respective effect extent of the aforementioned factors may vary significantly from each other when it comes to a specific condition. On the other hand, in view of multiple influencing factors and complex flow field under this circumstance, in the past, researchers often only discuss one or two factors’ influence in each article for simplifying the problem, therefore, the integrated effect of simultaneously changing several parameters remain to be investigated in the future.

     Considering that high Reynolds numbers always result in very complicated vortex structures in the wake of a finite circular cylinder (especially when mounted on a non-slip bottom wall), this paper presents a detailed discussion on the flow around a finite circular cylinder with AR=1 at a relatively high Re numbers (viz. 20000). The purpose of this study is to quantitatively and qualitatively make a detailed comparison of different turbulence models when it comes to the mean pressure coefficient profile Cp in the mid-height plane of the cylinder, the mean drag coefficient Cd of the cylinder, the time-averaged velocity and pressure fields, and the distribution of mean bed-shear-stress amplification on the bottom wall.

2. Configuration and Numerical Model

2.1 Test Configuration

       A similar geometric configuration as that used by Zhang et al. [6, 8] is employed in this study. As illustrated in Fig. 1, a finite circular cylinder with a non-dimensional width of D=1 and a nondimensional height of h=1 is vertically mounted on a plane boundary, and the (streamwise) length, (transverse) width and (spanwise) height of the computational domain are, respectively, L=30D, W=22D and H=2D (which gives an area blockage ratio of 2.27%). Further, the junction section between the cylinder and the bottom wall is centered at the origin of the coordinate system, which means that the inlet boundary is located at 10D upstream of the cylinder and the outlet boundary is situated at 20D downstream of the cylinder (i.e. L1=10D, L2=20D).

2.2 Governing Equations

2.2.1. RANS models.

The unsteady 3D Reynolds-averaged Navier-Stokes (RANS) equations employed in this study can be obtained by taking ensemble average of instantaneous mass and momentum conservation equations for incompressible and isothermal flows, as shown in the following.

2.3. Boundary Conditions and Numerical Schemes

Four kinds of boundary conditions are involved:

       1. Inlet: For the velocity field, a fixed uniform velocity is prescribed (i.e. I u =1, I v =wI =0), and, for the pressure field, the zero-gradient condition is imposed (i.e. 0 Ip n ).

      2. Outlet: For the velocity field, the convective outflow boundary condition is adopted: (11) where denotes all the three velocity components (viz. u, v and w), and c u is the advective velocity at the outlet boundary. For the pressure field, the homogeneous Dirichlet condition is utilized at the outlet boundary (i.e. p=0).

     3. Bottom wall and the surface of the obstacle: No-slip impermeable boundary condition is prescribed for the velocity field (i.e. u=v=w=0), and the zero-gradient condition is employed for the pressure field.

     4. Top and lateral boundaries of the computational domain: Free-slip condition is prescribed, which means that the velocity component normal to the boundary is zero (i.e. 0 b u ) and the normal gradients of both the pressure and the tangential velocity component are zero (i.e. // 0 b b u n p n , where b// u is the tangential velocity component).

چکیده

1.مقدمه

2.پیکربندی و مدل عددی

2.1 تست پیکربندی

2.2 معادلات حاکم

2.3  شرایط مرزی و برنامه های عددی

3.  نتایج و بحث

3.1  ضریب نفوذ متوسط و مشخصات فشار سطح

3.2 سرعت متوسط و فشار میدان

3.3 توزیع تنش برشی تخت

4. نتیجه گیری

5. تشکر و قدردانی

Abstract

1. Introduction 

2. Configuration and Numerical Model

2.1 Test Configuration

2.2 Governing Equations

2.3. Boundary Conditions and Numerical Schemes

3. Results and Discussion

3.1. Mean Drag Coefficient and Surface-Pressure Profile 

3.2. Mean Velocity and Pressure Fields 

3.3. Bed-Shear-Stress Distribution

4. Conclusions

5. Acknowledgments

6. References